Abstract
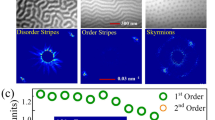
Inverse freezing and inverse melting are processes where a more symmetric phase is found at lower temperatures than at higher temperatures. Such inverse transitions are very rare1. Here we report the existence of an inverse transition effect in ultrathin Fe films that are magnetized perpendicular to the film plane. The magnetization of these films is not uniform, but instead manifests itself as stripe domains with opposite perpendicular magnetization2,3,4. Predictions relating to the disordering of this striped ground state in the limit of monolayer film thicknesses are controversial. Mean-field arguments5,6,7 predict a continuous reduction of the stripe width when the temperature is increased; other studies8,9,10,11 suggest that topological defects, such as dislocations and disclinations, might penetrate the system and induce geometrical phase transitions. We find, from scanning electron microscopy imaging, that when the temperature is increased, the low-temperature stripe domain structure transforms into a more symmetric, labyrinthine structure. However, at even higher temperatures and before the loss of magnetic order, a re-occurrence of the less symmetric stripe phase is found. Despite the widespread theoretical and experimental work on striped systems, this phase sequence and the microscopic instabilities driving it have not been observed before.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Greer, A. L. Too hot to melt. Nature 404, 134–135 (2000)
Garel, T. & Doniach, S. Phase transitions with spontaneous modulation—the dipolar Ising ferromagnet. Phys. Rev. B 26, 325–329 (1982)
Seul, M. & Andelman, D. Domain shapes and patterns: the phenomenology of modulated phases. Science 267, 476–483 (1995)
Sornette, D. Undulation instability in stripe domain structures of bubble materials. J. Phys. 48, 151–163 (1987)
Czech, R. & Villain, J. Instability of two-dimensional Ising ferromagnets with dipole interaction. J. Phys. Condens. Matter 1, 619–627 (1989)
Kashuba, A. & Pokrovsky, V. L. Stripe domain structures in a thin ferromagnetic film. Phys. Rev. B 48, 10335–10344 (1993)
Gehring, G. A. & Keskin, M. The temperature dependence of the domain spacing in ultrathin magnetic films. J. Phys. Condens. Matter 5, L581–L585 (1993)
Nelson, D. R. in Fundamental Problems in Statistical Mechanics V (ed. Cohen, E. G. D.) 53–108 (North-Holland, Amsterdam, 1980)
Abanov, Ar., Kalatsky, V., Pokrovsky, V. L. & Saslow, W. M. Phase diagram of ultrathin ferromagnetic films with perpendicular anisotropy. Phys. Rev. B 51, 1023–1038 (1995)
De'Bell, K., MacIsaac, A. B. & Whitehead, J. P. Dipolar effects in magnetic thin films and quasi-two-dimensional systems. Rev. Mod. Phys. 72, 225–257 (2000)
Stoycheva, A. D. & Singer, S. J. Stripe melting in a two-dimensional system with competing interactions. Phys. Rev. Lett. 84, 4657–4660 (2000)
Vaterlaus, A. et al. Two-step disordering of perpendicularly magnetized ultrathin films. Phys. Rev. Lett. 84, 2247–2250 (2000)
Stampanoni, M., Vaterlaus, A., Aeschlimann, M., Meier, F. & Pescia, D. Magnetic properties of thin fcc iron films on Cu(001). J. Appl. Phys. 64, 5321–5324 (1988)
Thomassen, J. et al. Magnetic live surface layers in Fe/Cu(100). Phys. Rev. Lett. 69, 3831–3834 (1992)
Kirilyuk, A., Giergiel, J., Shen, J., Straub, M. & Kirschner, J. Growth of stabilized γ-Fe films and their magnetic properties. Phys. Rev. B 54, 1050–1063 (1996)
Man, K. L., Altman, M. S. & Poppa, H. Spin polarized low energy electron microscopy investigations of magnetic transitions in Fe/Cu(100). Surf. Sci. 480, 163–172 (2001)
Pierce, J. P., Torija, M. A., Shen, J. & Plummer, E. W. Mapping the magnetic phase diagram of metastable fct Fe/Cu(100) using Co atoms. Phys. Rev. B 64, 224409 (2001)
Kivelson, S. A., Fradkin, E. & Emery, V. J. Electronic liquid-crystal phases of a doped Mott insulator. Nature 393, 550–553 (1998)
Seul, M. & Wolfe, R. Evolution of disorder in two-dimensional stripe patterns: Smectic instabilities and dislocation unbinding. Phys. Rev. Lett. 68, 2460–2463 (1992)
Yochelis, A., Hagberg, A., Meron, E., Lin, A. L. & Swinney, H. L. Development of standing-wave labyrinthine patterns. SIAM J. Appl. Dyn. Syst. 1, 236–247 (2002)
Newell, A. C., Passot, T., Bowman, C., Ercolani, N. & Indik, R. Defects are weak and self-dual solutions of the Cross-Newell phase diffusion equation for natural patterns. Physica D 97, 185–205 (1996)
Harrison, C. et al. Mechanism of ordering in striped patterns. Science 290, 1558–1560 (2000)
Pearson, J. E. Complex patterns in a simple system. Science 261, 189–192 (1993)
Acknowledgements
We thank the Swiss National Foundation and ETH Zurich for financial support.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Competing interests
The authors declare that they have no competing financial interests.
Rights and permissions
About this article
Cite this article
Portmann, O., Vaterlaus, A. & Pescia, D. An inverse transition of magnetic domain patterns in ultrathin films. Nature 422, 701–704 (2003). https://doi.org/10.1038/nature01538
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/nature01538
This article is cited by
-
Above-room-temperature strong intrinsic ferromagnetism in 2D van der Waals Fe3GaTe2 with large perpendicular magnetic anisotropy
Nature Communications (2022)
-
Inverse transition of labyrinthine domain patterns in ferroelectric thin films
Nature (2020)
-
Imaging and control of critical fluctuations in two-dimensional magnets
Nature Materials (2020)
-
On the mechanism behind the inverse melting in systems with competing interactions
Scientific Reports (2019)
-
Magnetic reversal in perpendicularly magnetized antidot arrays with intrinsic and extrinsic defects
Scientific Reports (2019)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.