Main

Bosons in an optical lattice are well described by the single-band Bose–Hubbard model9,10 when the occupancy in higher bands can be neglected, given by,

where bi(bi+) is the boson destruction (creation) operator at a site i, ni=bi+bi is the density operator and z=6is the coordination number in three dimensions. Both t and U are calculated directly for a given lattice depth V0, which can be tuned by the laser intensity10. At large t/U, the system is a normal Bose gas at high T and undergoes a classical phase transition to a superfluid at the transition temperature Tc. As t/U decreases, Tc is suppressed and eventually becomes zero at (t/U)c, the quantum critical point, as shown in Fig. 1a. As t/U is reduced further, the system becomes a Mott insulator with a gap in the excitation spectrum. In the quantum critical region, the bosons are strongly interacting and highly incoherent as the degrees of freedom reorganize between well-defined collective modes in the superfluid to well-defined single-particle modes in the Mott insulator.

Figure 1: Phase diagram.
figure 1

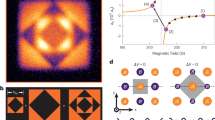
a, The superfluid transition temperature Tc (red) and the single-particle energy gap Δ in the Mott insulator (blue) vanish at the quantum phase transition as a function of tuning t/U, the ratio of hopping to onsite repulsion. For μ/U=1/2,(t/U)c=0.192(2). The corresponding lattice depth values V0 in units of the recoil energy ER=h2/8m d2 are indicated on the top axis. Here, m is the mass of the rubidium atom and d is the lattice constant. The typical interference patterns in the superfluid, Mott and critical regions are also shown. b, The superfluid density ρs in units of 1/d 3 as a function of T for various t/U. c, The superfluid density ρs and the condensate density n0 both in units of 1/d 3 as a function of t/U at T=0.1t.

Full size image

To identify the phases, we concentrate on the momentum distribution n(k), a quantity of fundamental interest that encapsulates the strong correlations of the system. n(k) is measured in time-of-flight experiments by releasing the atomic cloud from the optical lattice potential and the magnetic trap. Given the strong confinement energy released from each site in the optical lattice, the expansion is largely ballistic and the calculated n(k) then represents the interference pattern11. Here, we ignore interaction effects in the expansion, which is a small perturbation on the final observed interference pattern. The final density distribution in the interference pattern is12

where W(k) is the Fourier transform of the Wannier function in the lattice, τ is the expansion time and

is the momentum distribution function of bosons before expansion and is the central quantity of interest. The final image detects the column-integrated momentum distribution . Typically, the interference pattern has two distinct types of behaviour: (1) a blob considered to be representative of the Mott insulating incoherent phase, and (2) a bimodal structure, with a broad background above which there are visibly sharp peaks located at the reciprocal lattice vectors. The peaks are well separated from each other with a width less than the reciprocal lattice vector. The presence of sharp peaks has been considered an unequivocal signature of superfluidity in the Bose system4,5.

The questions that arise are as follows. (1) Are sharp peaks in the interference pattern a reliable diagnostic of superfluidity? (2) What is the fate of the sharp peaks as the interactions between bosons are increased at a fixed temperature moving towards the quantum phase transition? (3) Furthermore, at the quantum critical point, what happens to the interference pattern as T is reduced in a regime with no superfluidity?

To include the effect of strong interactions ‘exactly’, we use the world-line quantum Monte Carlo method13,14, applied to the hamiltonian in equation (1), based on the general idea of the directed-loop algorithm with modifications to improve efficiency near a critical point15. The accuracy of the method is tested in various ways by comparisons with exact diagonalization for small systems, and checks on the exponents with known results for the three-dimensional XY model. To further test that the true equilibrium distribution is sampled on large systems, several independent runs with varying length are carried out, showing no systematic deviation, thereby ensuring that our numerical results are ‘exact’ except for statistical errors.

We calculate the correlations and excitations of a Bose–Hubbard model as a function of t/U and temperature T at fixed μ/U=0.5. As shown in Fig. 1a, the Mott phase is identified by a finite single-particle gap Δ obtained from the imaginary time decay of Green’s function. The superfluid density ρs=T〈W2〉/(L t) is calculated from the fluctuations of the winding number16 W for a system of size L3 and is shown as a function of temperature in Fig. 1b. A finite-size scaling analysis of ρs gives the transition temperature Tc, shown in Fig. 1a. It is also interesting to compare the superfluid density ρs with the condensate density n0. In the superfluid phase, the single-particle density matrix 〈bi+bj〉 approaches a constant equal to n0 at large relative separation. The dependence of both ρsand n0 on t/U is shown in Fig. 1c. It is instructive to compare the behaviour of cold atoms in optical lattices with that of He4, in which the superfluid density ρs approaches the total density at low temperatures, whereas the condensate density n0 is only 0.1. In optical lattices, both ρs and n0 can be driven to zero at the quantum critical point by tuning the interactions.

For cold atoms in optical lattices, ρs has not been measured so far. We therefore focus on signatures of superfluidity in the momentum distribution. Turning to our calculations, we obtain the momentum distribution from equation (3) and input it in equation (2) to obtain the density distribution in the interference pattern at different temperatures and t/U. Figure 2 shows the evolution of the interference pattern for two lattice depths that correspond to the superfluid phase and close to the quantum critical point. Starting from a density profile that is a smooth gaussian at very high temperatures, a bimodal structure appears in the interference pattern, as T decreases. Sharp peaks emerge above a broad background even when T is still above Tc and the superfluid density is zero. The width of these peaks is narrower than the reciprocal lattice vector in a large temperature region above Tc. For example, in the right panel of Fig. 2b the full-width at half-maximum at t/U=0.25 is already 1/8 of the reciprocal lattice spacing at T/Tc=1.42. Especially near the transition point (t/U)c, sharp peaks can occur at temperatures much higher than Tc.

Figure 2: Interference pattern.
figure 2

a–d, The column-integrated momentum distribution (a,c) and the cut (b,d). The parameters in a and b are t/U=0.25,V0=11.5ER and Tc/t=0.7; in c and d, t/U=0.195, V0=12.4ER and Tc/t=0.19. The clearly resolved sharp peaks, on top of the broad background, represented by the red dashed curve, in the central and right panels of b and d constitute a bimodal structure in the interference pattern above Tc. In the left panel of b and d, an extra peak arises, shown as a singular feature and leads to a trimodal structure below Tc. Note that this δ-function singular feature rises above the already sharp feature (shown in solid purple) that was present even above Tc. The superfluid density ρs=0.44 and 0.04 corresponding to the left column of b and d, respectively. For all other columns, ρs=0. The lattice size is NL=123.

Full size image

It was pointed out by Diener et al. 3 that for an ideal normal bosonic gas in an optical lattice, the interference pattern can have sharp features above Tc. What is unexpected from the quantum Monte Carlo results is that even in a strongly interacting system close to the quantum critical point where interactions tend to broaden the peaks, it is nevertheless possible to see sharp peaks above Tc. Starting in the normal state for a weakly interacting system, as t/U is reduced at a fixed temperature T0, and interactions between bosons dominate, the peaks in the interference pattern get broadened. At the same time, interactions have another effect of strongly suppressing Tc and in fact driving it to zero at the quantum critical point. So, if we now reduce T below T0, still remaining above Tc, the peaks are seen to sharpen and at the quantum critical point, as we explain below, the momentum distribution at small momentum diverges at T=0. Thus, it is clear that a bimodal distribution in the interference pattern that shows sharp peaks is not a signature for superfluidity, and using it as a criterion for superfluidity can lead to serious errors.

If we next consider the interference pattern in the left panel of Fig. 2b,d for T<Tc, we see the emergence of a δ-function singularity over and above the regular sharp peaks that were already seen above Tc. This singular peak breaks off from the already bimodal distribution above Tc. Its height grows with the number of particles in the system and its width scales as ≈1/L, limited only by the system size L. It signals the emergence of the condensate and is the true and unequivocal signature of superfluidity. In the experiment, the presence of a confining harmonic trap makes the system inhomogeneous, and leads to the coexistence of multiple phases. In such a situation, the interference pattern should be examined more carefully to detect the superfluid in the system, as the width of the peak scales as the inverse of the linear size of the superfluid region.

To quantitatively analyse the interference pattern, the visibility is measured in many experiments7,8,17 defined by,

where , , G=2π/d and is a reciprocal lattice vector; is rotated by 45∘ around the axis. The robust feature about the visibility is that in the superfluid phase, υ=1 dominated by the macroscopic number of atoms in the condensate NA≈O(N). This result does not change if we include a finite bin size and average over a small area for both A and B. Certainly if the simulation size is small and the condensate does not contain a macroscopic number of particles, then changing the bin size can significantly affect the visibility and obscure the superfluid phase18. In our simulations on 123 size lattices, even with a bin size δ k=1/12 of the reciprocal lattice vector, the visibility remains robust at unity for V0=9.3ER and 0.94 for V0=11.5ER, which is already very close to the quantum critical point Vc=12.5ER. Here ER=h2/8m d 2 is the recoil energy in terms of the optical lattice spacing d and mass of atoms m. We expect the visibility in the experiments with 503–703 lattice sites to be very robust at unity below Tc. Another salient feature of the visibility is that it is almost T independent below Tc, because n(k=0) decreases slowly below Tc, as shown in Fig. 3b. On the other hand, it decreases rapidly near Tc, enabling us to roughly estimate Tc from the peak in dυ/dT. At the quantum critical point, υ can also be fairly high owing to the large population as k→0 even though there is no singularity in n(k).

Figure 3: Visibility.
figure 3

a, υ defined in equation (4) for a system of size NL=123. For each parameter V0/ER, the two curves with the same colour of symbols correspond to averaging over different bin sizes δ k. The squares correspond to δ k=0and the triangles to δ k=π/6. In the superfluid phase, the visibility becomes almost unity below Tc and is not much affected by small δ k. b, The corresponding zero momentum population n(k=0). The inset shows that Tc can be estimated roughly from the peak in the T-derivative of the visibility. Tc1=1t and Tc2=0.7t are the critical temperatures from Fig. 1 for V0=9.30ER and V0=11.5ER, respectively.

Full size image

In Fig. 2, both quantum and thermal fluctuations result in uncondensed bosons with a contribution that is convoluted with the Wannier function to produce the broad gaussian background. We directly analyse the crystal momentum distribution n(k), without any obfuscation from the Wannier function, in the first Brillouin zone before expansion19. Figure 4a,b shows the crystal momentum distribution just above and below Tc. In the superfluid phase

where N0 is the number of Bose condensed atoms at k=0, whereas nreg(k) is the regular uncondensed bosons. When the temperature goes above Tc, the δ-function singular part N0 disappears, which indicates the vanishing of superfluid order (Fig. 4b). However, in a strongly correlated superfluid close to the quantum critical point, nreg(k→0) can become large, resulting in sharp peaks in the corresponding interference pattern. The fact that n(k) can become sharply peaked but not singular at temperatures above Tc was also found in neutron scattering experiments20 on He4.

Figure 4: Crystal momentum distribution.
figure 4

n(kx,0,0) defined in equation (5) for a system of size NL=123 at t/U=0.25. a, T/Tc=0.96 showing a distinct condensate N0 that is singular and breaks off from a smooth background nreg. b, T/Tc=1.1 showing that the singular contribution N0 is absent. Note that the regular contribution nreg(k→0) can become large close to the transition.

Full size image

In analogy with the non-interacting boson distribution, Fig. 5 shows ɛk*≡Tlog(1+1/n(k)) as a function of ɛk0 for various T at (t/U)c, where is the bare single-particle energy. At high T=10t, we obtain a linear fit, which implies that the boson distribution is well described by

as weakly interacting particles. Here, is the renormalized dispersion arising from a renormalized bandwidth t* and a renormalized chemical potential μ*. At T=3t, deviations are seen from linear behaviour, which grow with decreasing T. The slope of the curve changes smoothly enabling linear fits to the low- (with parameters t1* and μ1*) and high- (with parameters t2* and μ2*) energy regimes that reveal strong renormalizations of the parameters. The significance of this finding is that in the low-energy regime n(k) from equation (6) can be described by n(k)=(T/|μ1*|)/(1+k2ξ2) at the quantum critical point where |μ1*| is found to be much less than T and t1* and the healing length is . At the quantum critical point as T→0, we find that μ1*/T→0 but t1* is finite resulting in a correlation length ξ(T) that becomes larger with decreasing T and finally diverges.

Figure 5: Momentum distribution function at the quantum critical point.
figure 5

n(k) for a NL=243 system is plotted by extracting the effective dispersion ɛk*≡T log(1+1/n(k)) in analogy with the free boson nfree(k) a, At high T=10t, the almost linear fit indicates that the bosons are well described as free particles with weakly renormalized parameters. b–d, As T is lowered to T=3t (b), T=t (c) and T=t/3 (d), there are distinct deviations from linearity that increase with decreasing T. Separate linear fits to the low- (t1* and μ1*) and high- (t2* and μ2*) energy parts of the spectrum show strong renormalizations of the effective bandwidth and chemical potential.

Full size image

The precise form of the dispersion and spectral function of bosons in optical lattices are of fundamental interest. We hope our computational and theoretical analysis will inspire further investigations of strongly interacting systems, especially in the quantum critical region21.