Abstract
Quantum devices for sensing and computing applications require coherent quantum systems, which can be manipulated in fast and robust ways1. Such quantum control is typically achieved using external electromagnetic fields, which drive the system’s orbital2, charge3 or spin4,5 degrees of freedom. However, most existing approaches require complex and unwieldy gate structures, and with few exceptions6,7 are limited to the regime of weak coherent driving. Here, we present a novel approach to coherently drive a single electronic spin using internal strain fields8,9,10 in an integrated quantum device. Specifically, we employ time-varying strain in a diamond cantilever to induce long-lasting, coherent oscillations of an embedded nitrogen–vacancy (NV) centre spin. We perform direct spectroscopy of the phonon-dressed states emerging from this drive and observe hallmarks of the sought-after strong-driving regime6,11, where the spin rotation frequency exceeds the spin splitting. Furthermore, we employ our continuous strain driving to significantly enhance the NV’s spin coherence time12. Our room-temperature experiments thereby constitute an important step towards strain-driven, integrated quantum devices and open new perspectives to investigate unexplored regimes of strongly driven multilevel systems13 and exotic spin dynamics in hybrid spin-oscillator devices14.
Similar content being viewed by others
Main
The use of crystal strain for the manipulation of single quantum systems (‘spins’) in the solid state brings vital advantages compared to established methods relying on electromagnetic fields. Strain fields can be straightforwardly engineered in the solid state and can offer a direct coupling mechanism to embedded spins8,15. As they are intrinsic to these systems, strain fields are immune to drifts in the coupling strength. Also, strain does not generate spurious stray fields, which are unavoidable with electric or magnetic driving and can cause unwanted dephasing or heating of the environment. Furthermore, coupling spins to strain offers attractive features of fundamental interest. For instance, strain can be used to shuttle information between distant quantum systems15, and has been proposed to generate squeezed spin ensembles14 or to cool mechanical oscillators to their quantum ground state16. These attractive perspectives for strain-coupled hybrid quantum systems motivated recent studies of the influence of strain on nitrogen–vacancy (NV) centre electronic spins9,10,17 and experiments on strain-induced, coherent driving of large NV spin ensembles8. Promoting such experiments to the single-spin regime, however, remains an outstanding challenge, and would constitute a major step towards the implementation of integrated, strain-driven quantum systems.
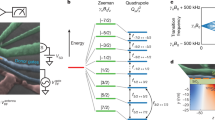
Here, we demonstrate the coherent manipulation of a single electronic spin using time-periodic, intrinsic strain fields generated in a single-crystalline diamond mechanical oscillator. We show that such strain fields allow us to manipulate the spin in the strong-driving regime, where the spin manipulation frequency significantly exceeds the energy splitting between the two involved spin states, and to protect the spin from environmental decoherence. Our experiments were performed on electronic spins in individual NV lattice point defect centres, embedded in single-crystalline diamond cantilevers. The negatively charged NV centres we studied have a spin S = 1 ground state with basis states {|0〉, |−1〉, |+1〉}, where |ms〉 is an eigenstate of the spin operator  along the NV’s symmetry axis, z (Fig. 1a). The energy difference between |±1〉 and |0〉 is given by the zero-field splitting D0 = 2.87 GHz. The levels |±1〉 are split by 2γNVBNV (with γNV = 2.8 MHz G−1) in a magnetic field BNV applied along z. Hyperfine interactions between the NV’s electron and 14N nuclear spin (I = 1 and quantum number mI) further split the NV spin levels by an energy AHF = 2.18 MHz into states |ms, mI〉 (Fig. 1a)5. In our experiments, we use optical excitation and fluorescence detection to initialize and read out the NV spin18 with a homebuilt confocal optical microscope9 (Methods). Furthermore, we use microwave magnetic fields to perform optically detected electron spin resonance (ESR; Fig. 1b) and manipulate the NV’s electronic spin states.
along the NV’s symmetry axis, z (Fig. 1a). The energy difference between |±1〉 and |0〉 is given by the zero-field splitting D0 = 2.87 GHz. The levels |±1〉 are split by 2γNVBNV (with γNV = 2.8 MHz G−1) in a magnetic field BNV applied along z. Hyperfine interactions between the NV’s electron and 14N nuclear spin (I = 1 and quantum number mI) further split the NV spin levels by an energy AHF = 2.18 MHz into states |ms, mI〉 (Fig. 1a)5. In our experiments, we use optical excitation and fluorescence detection to initialize and read out the NV spin18 with a homebuilt confocal optical microscope9 (Methods). Furthermore, we use microwave magnetic fields to perform optically detected electron spin resonance (ESR; Fig. 1b) and manipulate the NV’s electronic spin states.
a, Energy levels of the NV spin as a function of magnetic field applied along the NV axis. Electronic spin states |ms = ±1〉 each split into three levels owing to hyperfine interactions with the NV’s 14N nuclear spin (I = 1, AHF = 2.18 MHz). Wavy lines indicate strain (red) and microwave (violet) fields of frequency ω and strength Ω. b, Optically detected electron spin resonance of a single NV centre. c, Experimental set-up. NV spins (red) are coupled to a cantilever, which is resonantly driven at frequency ωm by a piezo-element. The NV spin is read out and initialized by green laser light and manipulated by microwave magnetic fields generated by a nearby antenna. d, Pulse sequence employed to observe strain-induced Rabi oscillations. e, Strain-driven Rabi oscillations. Data (blue) and a fit (black) to damped Rabi oscillations.
Coherent strain driving of NV spins is based on the sensitive response of the NV spin states to strain in the diamond host lattice. For uniaxial strain applied transverse to the NV axis, the corresponding strain-coupling Hamiltonian takes the form14

Here, ℏ is the reduced Planck constant, γ0⊥ the transverse single-phonon strain-coupling strength and  and
and  are the raising (lowering) operators for spin and phonons, respectively. Transverse strain thus leads to a direct coupling of the two electronic spin states |−1〉 and |+1〉 (ref. 9) and, in the case of near-resonant, time-varying (a.c.) strain, can coherently drive the transitions |−1, mI〉 ↔ |+1, mI〉 (ref. 8). For a classical (coherent) phonon field at frequency ωm/2π, equation (1) can be written as
are the raising (lowering) operators for spin and phonons, respectively. Transverse strain thus leads to a direct coupling of the two electronic spin states |−1〉 and |+1〉 (ref. 9) and, in the case of near-resonant, time-varying (a.c.) strain, can coherently drive the transitions |−1, mI〉 ↔ |+1, mI〉 (ref. 8). For a classical (coherent) phonon field at frequency ωm/2π, equation (1) can be written as  , where Ωm = γ0⊥xc/xZPF describes the amplitude of the strain drive, with xZPF and xc the cantilever’s zero-point fluctuation and peak amplitude, respectively (here, with xZPF ∼7.7 × 10−15 m and γ0⊥/2π ∼ 0.08 Hz, Supplementary Information). Interestingly, strain drives a dipole-forbidden transition (Δms = 2), which would be difficult to access, for example, using microwave fields.
, where Ωm = γ0⊥xc/xZPF describes the amplitude of the strain drive, with xZPF and xc the cantilever’s zero-point fluctuation and peak amplitude, respectively (here, with xZPF ∼7.7 × 10−15 m and γ0⊥/2π ∼ 0.08 Hz, Supplementary Information). Interestingly, strain drives a dipole-forbidden transition (Δms = 2), which would be difficult to access, for example, using microwave fields.
To generate and control a sizeable a.c. strain field for efficient coherent spin driving, we employed a mechanical resonator in the form of a singly clamped, single-crystalline diamond cantilever9, in which the NV centre is directly embedded (Fig. 1c and Methods). The cantilever was actuated at its mechanical resonance frequency ωm/2π = 6.83 ± 0.02 MHz using a piezo-element placed nearby the sample. We controlled the detuning between the mechanical oscillator and the |−1〉 ↔ |+1〉 spin transition by applying an adjustable external magnetic field BNV along the NV axis (Fig. 1a and Methods).
To demonstrate coherent NV spin manipulation using resonant a.c. strain fields, we first performed strain-driven Rabi oscillations between |−1〉 and |+1〉 for a given hyperfine manifold (here, mI = 1). To that end, we initialized the NV in |−1,1〉 by applying an appropriate sequence of laser and microwave pulses (Fig. 1d). We then let the NV spin evolve for a variable time τ, under the influence of the coherent a.c. strain field generated by constantly exciting the cantilever at a fixed peak amplitude xc ∼ 100 nm (Supplementary Information). After this evolution, we measured the resulting population in |−1,1〉 with a pulse sequence analogous to our initialization protocol. As expected, we observe strain-induced Rabi oscillations (Fig. 1e) for which we find a Rabi frequency Ωm/2π = 1.14 ± 0.01 MHz and hardly any damping over the 30 μs observation time. Importantly, and in contrast to a recent study on NV ensembles8, this damping timescale is not limited by ensemble averaging, because our experiment was performed on a single NV spin.
We obtain further insight into the strength and dynamics of our coherent strain-driving mechanism from ESR spectroscopy of the strain-coupled NV spin states, |+1〉 and |−1〉. For this, we employed a weak microwave tone at frequency ωMW/2π to probe the |0〉 ↔ |± 1〉 transitions as a function of BNV in the presence of the coherent strain field (Fig. 2b). This field has a striking effect on the NV’s ESR spectrum in that it induces excitation gaps at ωMW − 2πD0 = ±ωm/2, that is, for BNV ∼ 0.9,1.6 and 2.3 G. At these values of BNV the a.c. strain field in the cantilever is resonant with a given hyperfine transition, that is, the energy splitting between |−1, mI〉 and |+1, mI〉 equals ℏωm. The energy gaps which we observe in the ESR spectra under resonant strain driving are evidence of the Autler–Townes (AT) effect—a prominent phenomenon in quantum electrodynamics19,20, which has previously been observed in atoms and molecules21, quantum dots22 and superconducting qubits23. Our observation of the AT effect was performed on a single electronic spin in the microwave domain, and to the best of our knowledge constitutes the first observation of the AT effect under ambient conditions.
a, Eigenenergies of the joint spin–phonon system with basis states |ms; N〉 as a function of the spin splitting . Strain couples |1; N〉 and |−1; N + 1〉 and, whenever the resonance condition = ωm is fulfilled, leads to new eigenstates |+(N)〉 and |−(N)〉 with energy splitting ℏΩm (see text). b, Microwave spectroscopy of phonon-dressed states using a weak microwave probe at ωMW. For mI = −1, 0 and 1, resonance is separately established at BNV ∼0.9, 1.6 and 2.3 G. c, Dependence of the energy gap between |+(N)〉 and |−(N)〉 on mechanical driving strength. As expected, the gap scales linearly with Ωm for each hyperfine state. Data was recorded over the parameter range indicated by white dashed lines in b.
The observed AT splitting can be understood by considering the joint energetics of the NV spin states and the quantized strain field used to drive the spin20 (Fig. 2a). The joint basis states |i; N〉 consist of NV spin states |i〉 dressed by N phonons in the cantilever. Strain couples |+1; N〉 to |−1; N + 1〉 and leads to new eigenstates |±(N)〉, which anti-cross on resonance, where  are split by an energy ℏΩm. As expected, this splitting increases linearly with the driving field amplitude (Fig. 2c), which we control through the strength of piezo excitation.
are split by an energy ℏΩm. As expected, this splitting increases linearly with the driving field amplitude (Fig. 2c), which we control through the strength of piezo excitation.
To investigate the limits of our coherent, strain-induced spin driving and study the resulting, strongly driven spin dynamics, we performed detailed dressed-state spectroscopy as a function of drive strength (Fig. 3a). To that end, we first set BNV such that and then performed microwave ESR spectroscopy for different values of Ωm. For weak driving, Ωm ≪ ωm, the dressed states emerging from the resonantly coupled states |−1,1〉 and |+1,1〉 split linearly with Ωm. The linear relationship breaks down for owing to multi-phonon couplings involving states which belong to different sub-spaces spanned by |±(N)〉 and |±(M)〉, with N ≠ M (ref. 20). This observation is closely linked to the breakdown of the rotating-wave approximation24 and indicates the onset of the strong-driving regime we achieve in our experiment.
a, Microwave spectroscopy of the mechanically driven NV spin at BNV = 1.8 G (where ωm = ) as a function of drive strength Ωm. The resonantly coupled states (|±1, +1〉) at ωMW/2π −D0 = ±2.98 MHz first split linearly with BNV and then evolve into a sequence of crossings and anti-crossings (green circles and crosses, respectively) with higher-order dressed states, indicative of the strong-driving regime. b, Calculated transition rates from |ms = 0〉 to the dressed states obtained by Fermi’s golden rule (Methods). Blue, green and orange shaded transitions correspond to the hyperfine manifolds mI = +1, 0 and −1, respectively. In both panels, black dots indicate the calculated dressed-state energies for mI = +1. Grey lines in b show the same under the rotating-wave approximation. Deviations between black dots and the grey lines therefore indicate the onset of the strong-driving regime.
For even larger Rabi frequencies Ωm, the dressed states evolve into a characteristic sequence of crossings and anti-crossings. The (anti-)crossings occur in the vicinity of Ωm = qωm, with q an odd (even) integer, and are related to symmetries of the Hamiltonian (1) (see ref. 20; Supplementary Information). Our experiment allows us to clearly identify the q = 1 and q = 2 (anti-)crossings (circles and crosses in Fig. 3a) and thereby demonstrates that we reside well within the strong-driving regime (Ωm > ) of a harmonically driven two-level system. We have carried out an extensive numerical analysis (Fig. 3b and Methods), which shows quantitative agreement with our experimental findings. For the largest values of Ωm, some discrepancies of the transition strengths between data and model remain; we tentatively assign these to uncertainties in microwave polarization, to possible variations of linewidths with Ωm and to our particular ESR detection scheme25. Our calculation further shows that, over our range of experimental parameters, Ωm is linear in xc and reaches a maximum of Ωmmax/2π ∼ 10.75 MHz (at present limited by the maximally achievable piezo driving strength).
Continuous coherent driving can be employed to protect a quantum system from its noisy environment and thereby increase its coherence times12,26. For NV centre spins, decoherence is predominantly caused by environmental magnetic field noise5, which normally couples linearly to the NV spin through the Zeeman Hamiltonian HZ = γNVSzBNV (Fig. 1a). Conversely, for the dressed states |±(N)〉 we create by coherent strain driving, 〈 ±(N) |HZ |±(N)〉 = 0 and the lowest-order coupling to magnetic fields is only quadratic (Fig. 2a). These states are thus less sensitive to magnetic field fluctuations and should exhibit increased coherence times relative to the undriven NV.
To demonstrate such coherence enhancement by continuous driving12, we performed Ramsey spectroscopy on our strain-driven NV spin and compared the resulting dephasing times T2∗ against the undriven case (Fig. 4). For this, we adjusted BNV such that and mechanically drove the NV with Ωm/2π = 1.68 MHz to induce phonon-dressing of the NV. We then used pulsed microwaves25 to perform Ramsey spectroscopy on the two Autler–Townes split dressed states emerging from |ms = +1, mI = +1〉. The resulting coherence signal (Fig. 4a) decays on a timescale of T2∗ = 16.4 ± 0.6 μs and shows beating of two long-lived oscillations at 0.63 MHz and 1.05 MHz stemming from the two dressed states we address (Supplementary Information). Compared to the bare NV dephasing time of T2∗ = 3.6 ± 0.1 μs (Fig. 4b), this demonstrates a significant enhancement of T2∗ caused by our continuous, mechanical drive.
a, Spin coherence decay of |±(N)〉 (formI = +1) as measured by Ramsey interferometry between the state |ms = 0〉 and the |ms = +1〉 manifolds using the pulse sequence depicted on the top. The probability for the NV to occupy the |ms = 0〉 manifold after the sequence is denoted as P(|ms = 0〉). The inset illustrates the NV spin’s eigenenergies as a function of BNV and indicates the magnetic field and microwave frequencies employed (purple dot) with respect to the dressed-state spectrum shown in Fig. 2b. b, Measurement of NV spin coherence time in the undriven case, as determined by Ramsey spectroscopy between |ms = 0〉 and |ms = +1〉, in the absence of mechanical driving. Inset as in a. In both panels, the orange envelope indicates the coherence decay extracted from our fit.
Although our protocol decouples NVs from magnetic field noise, it renders them vulnerable to fluctuations in electric field and strain. Most importantly, shallow NVs experience excess dephasing from fluctuating surface electric fields27, which are likely to dominate the residual dephasing we observe. Additional dephasing mechanisms of lesser relevance to our experiment include cantilever thermal noise (Supplementary Information) or second-order couplings28 of magnetic fields to the NV. Our decoupling protocol is readily tunable: for increasing Ωm, we have observed an initially monotonic, approximately linear increase of T2∗ (Supplementary Information), which saturates for Ωm/2π ≳ 1 MHz. We assign this current limitation to the onset of technical noise12, whose mitigation might lead to further improvements of T2∗ in the future. Furthermore, tunability offers interesting perspectives to systematically study the still largely unexplored, electric-field-induced dephasing processes for shallow NVs.
Our approach to strong coherent strain driving of a single electronic spin will have implications far beyond the coherence protection and dressed-state spectroscopy that we have demonstrated in this work. By combining our strain drive with coherent microwave spin manipulation, our NV spin forms an inverted three-level ‘Δ’-system, on which all three possible spin transitions can be coherently addressed. This setting is known to lead to unconventional spin dynamics29, which here could be observed on a single, highly coherent spin and exploited for sensing and quantum manipulation of our hybrid device. Strain-induced a.c. Stark shifts can furthermore be employed to dynamically tune30 the energies of the NV hyperfine states—an attractive perspective for the use of 14N nuclear spins as quantum memories5. The decoherence protection by continuous strain driving that we have demonstrated will have an impact for any quantum technology where pulsed decoupling protocols cannot be employed (such as d.c. electric field sensing). Further studies of the remaining decoherence processes under mechanical driving, which remain largely unexplored until now, offer another exciting avenue to be pursued in the future. On a more far-reaching perspective, our experiments lay the foundation for exploiting diamond-based hybrid spin-oscillator systems for quantum information processing and sensing, where our system forms an ideal platform for implementing proposed schemes for spin-induced phonon cooling and lasing31 or oscillator-induced spin squeezing14.
Methods
Sample fabrication.
Our cantilevers consist of single-crystalline, ultra-pure [001]-oriented diamond (Element Six, ‘electronic grade’), are aligned with the [110] crystal direction and have dimensions in the range of (0.2–1) × 3.5 × (15–25) μm3 for thickness, width and length, respectively. The fabrication process is based on recently established top-down diamond nanofabrication techniques32. In particular, we use electron beam lithography at 30 keV to pattern etch masks for our cantilevers into a negative tone electron beam resist (FOX-16 from Dow Corning, spun to a thickness of ∼500 nm onto the sample). The developed pattern directly acts as an etch mask and is transferred into the diamond surface using an inductively coupled plasma reactive ion etcher (ICP-RIE, Sentech SI 500). To create cantilevers with vertical sidewalls, we use a plasma containing 50% argon and 50% oxygen (gas flux 50 sccm each). The plasma is run at 1.3 Pa pressure, 500 W ICP source power and 200 W bias power. NV centres in our cantilevers were created before nanofabrication by 14N ion implantation with dose, energy and sample tilt of 1010 cm−2, 12 keV and 0°, respectively. Based on numerical simulations (using the ‘SRIM’ software package), this yields an estimated implantation depth of ∼17 nm. To create NV centres, we annealed our samples at high vacuum (≲10−6 mbar) in a sequence of temperature steps at 400 °C (4 h), 800 °C (2 h) and 1,200 °C (2 h).
Experimental set-up.
Experiments are performed in a homebuilt confocal microscope set-up at room temperature and at atmospheric pressure. A 532 nm laser (NovaPro 532-300) is coupled into the confocal system through a dichroic mirror (Semrock LM01-552-25). A microscope objective (Olympus XLMFLN40x) is used to focus the laser light onto the sample, which is placed on a micropositioner (Attocube ANSxyz100). Red fluorescence photons are collected by the same microscope objective, transmitted through the dichroic mirror and coupled into a single-mode optical fibre (Thorlabs SM600), which acts as a pinhole for confocal detection. Photons are detected using an avalanche photodiode (Laser Components Count-250C) in Geiger mode. Scan control and data acquisition (photon counting) are achieved using a digital acquisition card (NI-6733). The microwave signal for spin manipulation is generated by a SRS SG384 signal generator, amplified by a Minicircuit ZHL-42W+ amplifier and delivered to the sample using a homebuilt near-field microwave antenna. Laser, microwave and detection signals were gated using microwave switches (Minicircuit Switch ZASWA-2-50DR+), which were controlled through digital pulses generated by a fast pulse generator (SpinCore PulseBlasterESR-PRO). Gating of the laser is achieved using a double-pass acoustic optical modulator (Crystal Technologies 3200-146). Mechanical excitation of the cantilevers was performed with a piezoelectric element placed directly below the sample. The excitation signal for the piezo was generated with a signal generator (Agilent 3320A). A three-axis magnetic field was generated by three homebuilt coil pairs driven by constant-current sources (Agilent E3644A).
Measurement procedure and error bars.
ESR measurements were performed using a pulsed ESR scheme25, where the NV spin is first initialized in |ms = 0〉 using green laser excitation, then driven by a short microwave ‘π-pulse’ of length τ (that is, a pulse such that 2πΩMWτ = π) and finally read out using a second green laser pulse. Compared to conventional, continuous-wave ESR, this scheme has the advantage of avoiding power broadening of the ESR lines by green laser light and was therefore employed throughout this work.
Our experiments were performed on three different NV centres in three different cantilevers: data in Figs 1 and 4 were obtained on NV #1, whereas Figs 2 and 3 were recorded on NVs #2 and #3, respectively. NVs #1–3 all showed slightly different values of D0 owing to variations in static local strain and transverse magnetic fields. The zero-field splittings for these three NVs were D0 = 2.870 GHz, 2.871 GHz and 2.8725 GHz, respectively. The values of ωm for the cantilevers of NVs #1–3 were ωm/2π = 6.83,9.18 and 5.95 MHz, respectively.
Throughout this paper, errors represent 95% confidence intervals for the nonlinear least-squares parameter estimates to our experimental data. The only exception is the mechanical resonance frequency ωm, where error bars represent the linewidth of the cantilever resonance curves, which we measured optically in separate experiments. The actual error bars in determining ωm are significantly smaller than the linewidth and do not influence the findings presented in this paper.
Simulations.
Following ref. 11 we employ Floquet theory to treat the time dependence of the strain-induced spin driving,  , beyond the rotating-wave approximation (RWA), as it is expected to break down in the strong-driving limit Ωm > . The key idea here is to map the Hamiltonian with periodic time dependence on an infinite-dimensional, but time-independent Floquet Hamiltonian
, beyond the rotating-wave approximation (RWA), as it is expected to break down in the strong-driving limit Ωm > . The key idea here is to map the Hamiltonian with periodic time dependence on an infinite-dimensional, but time-independent Floquet Hamiltonian  . We can then solve the eigenvalue problem
. We can then solve the eigenvalue problem  with standard methods to obtain quasi-energies ℏωj and corresponding eigenvectors |uj〉.
with standard methods to obtain quasi-energies ℏωj and corresponding eigenvectors |uj〉.
Treating the weak microwave drive up to second order in drive strength we find the rate for the system to leave the initial state with Fermi’s golden rule as11

where the sum over i and f runs over all the eigenstates of the Floquet Hamltonian and the microwave driving Hamiltonian is  with drive frequency ΩMW, assuming a linearly polarized microwave field. For the simulations shown in Fig. 3b we assumed an initial state |ui〉 = |ms = 0, mI〉 and linewidths γfi = γ = 1 MHz, and summed the result incoherently over all nuclear spin quantum numbers mI ∈ {−1,0,1}.
with drive frequency ΩMW, assuming a linearly polarized microwave field. For the simulations shown in Fig. 3b we assumed an initial state |ui〉 = |ms = 0, mI〉 and linewidths γfi = γ = 1 MHz, and summed the result incoherently over all nuclear spin quantum numbers mI ∈ {−1,0,1}.
References
Nielsen, M. A. & Chuang, I. L. Quantum Computation and Quantum Information (Cambridge Univ. Press, 2000).
Nadj-Perge, S., Frolov, S. M., Bakkers, E. P. A. M. & Kouwenhoven, L. P. Spin–orbit qubit in a semiconductor nanowire. Nature 468, 1084–1087 (2010).
Clarke, J. & Wilhelm, F. K. Superconducting quantum bits. Nature 453, 1031–1042 (2008).
Hanson, R., Kouwenhoven, L. P., Petta, J. R., Tarucha, S. & Vandersypen, L. M. K. Spins in few-electron quantum dots. Rev. Mod. Phys. 79, 1217–1265 (2007).
Dobrovitski, V., Fuchs, G., Falk, A., Santori, C. & Awschalom, D. Quantum control over single spins in diamond. Ann. Rev. Condens. Matter Phys. 4, 23–50 (2013).
Oliver, W. D. et al. Mach-Zehnder interferometry in a strongly driven superconducting qubit. Science 310, 1653–1657 (2005).
London, P., Balasubramanian, P., Naydenov, B., McGuinness, L. P. & Jelezko, F. Strong driving of a single spin using arbitrarily polarized fields. Phys. Rev. A 90, 012302 (2014).
MacQuarrie, E. R. et al. Coherent control of a nitrogen–vacancy center spin ensemble with a diamond mechanical resonator. Optica 2, 233–238 (2015).
Teissier, J., Barfuss, A., Appel, P., Neu, E. & Maletinsky, P. Strain coupling of a nitrogen–vacancy center spin to a diamond mechanical oscillator. Phys. Rev. Lett. 113, 020503 (2014).
Ovartchaiyapong, P., Lee, K. W., Myers, B. A. & Jayich, A. C. B. Dynamic strain-mediated coupling of a single diamond spin to a mechanical resonator. Nature Commun. 5, 4429 (2014).
Silveri, M., Tuorila, J., Kemppainen, M. & Thuneberg, E. Probe spectroscopy of quasienergy states. Phys. Rev. B 87, 134505 (2013).
Xu, X. et al. Coherence-protected quantum gate by continuous dynamical decoupling in diamond. Phys. Rev. Lett. 109, 070502 (2012).
Danon, J. & Rudner, M. S. Multilevel interference resonances in strongly driven three-level systems. Phys. Rev. Lett. 113, 247002 (2014).
Bennett, S. D. et al. Phonon-induced spin–spin interactions in diamond nanostructures: Application to spin squeezing. Phys. Rev. Lett. 110, 156402 (2013).
Gustafsson, M. V. et al. Propagating phonons coupled to an artificial atom. Science 346, 207–211 (2014).
Wilson-Rae, I., Zoller, P. & Imamoglu, A. Laser cooling of a nanomechanical resonator mode to its quantum ground state. Phys. Rev. Lett. 92, 075507 (2004).
MacQuarrie, E. R., Gosavi, T. A., Jungwirth, N. R., Bhave, S. A. & Fuchs, G. D. Mechanical spin control of nitrogen–vacancy centers in diamond. Phys. Rev. Lett. 111, 227602 (2013).
Gruber, A. et al. Scanning confocal optical microscopy and magnetic resonance on single defect centers. Science 276, 2012–2014 (1997).
Autler, S. H. & Townes, C. H. Stark effect in rapidly varying fields. Phys. Rev. 100, 703–722 (1955).
Cohen-Tannoudji, C., Dupont-Roc, J. & Grynberg, G. Atom–Photon Interactions: Basic Processes and Applications (Wiley, 1992).
Wrigge, G., Gerhardt, I., Hwang, J., Zumofen, G. & Sandoghdar, V. Efficient coupling of photons to a single molecule and the observation of its resonance fluorescence. Nature Phys. 4, 60–66 (2008).
Xu, X. et al. Coherent optical spectroscopy of a strongly driven quantum dot. Science 317, 929–932 (2007).
Baur, M. et al. Measurement of Autler–Townes and Mollow transitions in a strongly driven superconducting qubit. Phys. Rev. Lett. 102, 243602 (2009).
Fuchs, G. D., Dobrovitski, V. V., Toyli, D. M., Heremans, F. J. & Awschalom, D. D. Gigahertz dynamics of a strongly driven single quantum spin. Science 326, 1520–1522 (2009).
Dréau, A. et al. Avoiding power broadening in optically detected magnetic resonance of single NV defects for enhanced dc magnetic field sensitivity. Phys. Rev. B 84, 195204 (2011).
Timoney, N. et al. Quantum gates and memory using microwave-dressed states. Nature 476, 185–188 (2011).
Dolde, F. et al. Electric-field sensing using single diamond spins. Nature Phys. 7, 459–463 (2011).
Ithier, G. et al. Decoherence in a superconducting quantum bit circuit. Phys. Rev. B 72, 134519 (2005).
Buckle, S., Barnett, S., Knight, P., Lauder, M. & Pegg, D. Atomic interferometers. Opt. Acta 33, 1129–1140 (1986).
Jundt, G., Robledo, L., Högele, A., Fält, S. & Imamoğlu, A. Observation of dressed excitonic states in a single quantum dot. Phys. Rev. Lett. 100, 177401 (2008).
Kepesidis, K. V., Bennett, S. D., Portolan, S., Lukin, M. D. & Rabl, P. Phonon cooling and lasing with nitrogen-vacancy centers in diamond. Phys. Rev. B 88, 064105 (2013).
Maletinsky, P. et al. A robust scanning diamond sensor for nanoscale imaging with single nitrogen–vacancy centres. Nature Nanotech. 7, 320–324 (2012).
Acknowledgements
We thank V. Jacques for fruitful discussions, P. Appel for initial assistance with nanofabrication and L. Thiel for support with the experiment control software. We gratefully acknowledge financial support from SNI; NCCR QSIT; SNF grants 200021_143697; and EU FP7 grant 611143 (DIADEMS). A.N. holds a University Research Fellowship from the Royal Society and acknowledges support from the Winton Programme for the Physics of Sustainability.
Author information
Authors and Affiliations
Contributions
A.B. and J.T. carried out the experiment and analysed the data. E.N. provided essential support in sample fabrication. A.N. provided theoretical support and modelled the data. All authors commented on the manuscript. P.M. wrote the manuscript, conceived the experiment and supervised the project.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Information (PDF 255 kb)
Rights and permissions
About this article
Cite this article
Barfuss, A., Teissier, J., Neu, E. et al. Strong mechanical driving of a single electron spin. Nature Phys 11, 820–824 (2015). https://doi.org/10.1038/nphys3411
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys3411
This article is cited by
-
Reconfigurable quantum phononic circuits via piezo-acoustomechanical interactions
npj Quantum Information (2022)
-
Optomechanical interface between telecom photons and spin quantum memory
Nature Physics (2021)
-
Quantum guidelines for solid-state spin defects
Nature Reviews Materials (2021)
-
Electrical control of coherent spin rotation of a single-spin qubit
npj Quantum Information (2020)
-
Coherent acoustic control of a single silicon vacancy spin in diamond
Nature Communications (2020)