Abstract
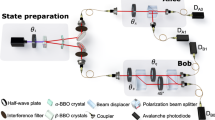
Quantum correlations, often observed as violations of Bell inequalities1,2,3,4,5, are critical to our understanding of the quantum world, with far-reaching technological6,7,8,9 and fundamental impact. Many tests of Bell inequalities have studied pairs of correlated particles. However, interest in multi-particle quantum correlations is driving the experimental frontier to test larger systems. All violations to date require supplementary assumptions that open results to loopholes, the closing of which is one of the most important challenges in quantum science. Seminal experiments have closed some loopholes10,11,12,13,14,15,16, but no experiment has closed locality loopholes with three or more particles. Here, we close both the locality and freedom-of-choice loopholes by distributing three-photon Greenberger–Horne–Zeilinger entangled states17 to independent observers. We measured a violation of Mermin's inequality18 with parameter 2.77 ± 0.08, violating its classical bound by nine standard deviations. These results are a milestone in multi-party quantum communication19 and a significant advancement of the foundations of quantum mechanics20.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Bell, J. S. On the Einstein Podolsky and Rosen paradox. Physics 1, 195–200 (1964).
Clauser, J. F. et al. Proposed experiment to test local hidden-variable theories. Phys. Rev. Lett. 23, 880–884 (1969).
Freedman, S. J. & Clauser, J. F. Experimental test of local-hidden variable theories. Phys. Rev. Lett. 28, 938–941 (1972).
Fry, E. S. & Thompson, R. C. Experimental test of local hidden-variable theories. Phys. Rev. Lett. 37, 465–468 (1976).
Aspect, A., Grangier, P. & Roger, G. Experimental realization of Einstein–Podolsky–Rosen–Bohm gedankenexperiment: a new violation of Bell's inequalities. Phys. Rev. Lett. 49, 91–94 (1982).
Bennett, C. H. & Brassard, G. in Proceedings of the IEEE International Conference on Computers, Systems, and Signal Processing 175–179 (IEEE, 1984).
Scarani, V. et al. The security of practical quantum key distribution. Rev. Mod. Phys. 81, 1301–1350 (2009).
Ladd, T. D. et al. Quantum computers. Nature 464, 45–53 (2010).
Nielsen, M. A. & Chuang, I. L. Quantum Computation and Quantum Information (Cambridge Univ. Press, 2000).
Rowe, M. A. et al. Experimental violation of Bell's inequality with efficient detection. Nature 409, 791–794 (2001).
Giustina, M. et al. Bell violation using entangled photons without the fair sampling assumption. Nature 497, 227–230 (2013).
Christensen, B. G. et al. Detection-loophole-free test of quantum nonlocality, and applications. Preprint at http://arxiv.org/abs/1306.5772 (2013).
Aspect, A., Dalibard, J. & Roger, G. Experimental test of Bell's inequalities using time-varying analyzers. Phys. Rev. Lett. 49, 1804–1807 (1982).
Weihs, G. et al. Violation of Bell's inequalities under strict Einstein locality conditions. Phys. Rev. Lett. 81, 5039–5043 (1998).
Scheidl, T. et al. Violation of local realism with freedom of choice. Proc. Natl Acad. Sci. USA 107, 19708–19713 (2010).
Barreiro, J. et al. Demonstration of genuine multipartite entanglement with device-independent witnesses. Nature Phys. 9, 559–562 (2013).
Greenberger, D. M., Horne, M. A. & Zeilinger, A. in Bell's Theorem, Quantum Theory, and Conceptions of the Universe (ed. Kafatos, M.) 73–76 (Kluwer Academic, 1989).
Mermin, N. D. Extreme quantum entanglement in a superposition of macroscopically distinct states. Phys. Rev. Lett. 65, 1838–1840 (1990).
Hillery, M., Buzček, V. & Berthiaume, A. Quantum secret sharing. Phys. Rev. A 59, 1829–1834 (1999).
Pan, J.-W. et al. Multiphoton entanglement and interferometry. Rev. Mod. Phys. 84, 777–838 (2012).
Greenberger, D. M. et al. Bell's theorem without inequalities. Am. J. Phys. 58, 1131–1143 (1990).
Bell, J. Bertlmann's socks and the nature of reality. J. Phys. Colloq. 42(C2), 41–62 (1981).
Pearle, P. M. Hidden-variable example based upon data rejection. Phys. Rev. D 2, 1418–1425 (1970).
Pan, J.-W. et al. Experimental test of quantum nonlocality in three-photon Greenberger–Horne–Zeilinger entanglement. Nature 403, 515–519 (2000).
Zhao, Z. et al. Experimental violation of local realism by four-photon Greenberger–Horne–Zeilinger entanglement. Phys. Rev. Lett. 91, 180401 (2003).
Lavoie, J., Kaltenbaek, R. & Resch, K. J. Experimental violation of Svetlichny's inequality. New J. Phys. 11, 073051 (2009).
Altepeter, J., Jeffrey, E. & Kwiat, P. Phase-compensated ultra-bright source of entangled photons. Opt. Express 13, 8951–8959 (2005).
Fedrizzi, A. et al. A wavelength-tunable fiber-coupled source of narrowband entangled photons. Opt. Express 15, 15377–15386 (2007).
Hübel, H. et al. Direct generation of photon triplets using cascaded photon-pair sources. Nature 466, 601–603 (2010).
Lamas-Linares, A., Howell, J. C. & Bouwmeester, D. Stimulated emission of polarization-entangled photons. Nature 412, 887–890 (2001).
Jennewein, T. et al. A fast and compact quantum random number generator. Rev. Sci. Instrum. 71, 1675–1680 (2000).
Gühne, O. & Tóth, G. Entanglement detection. Phys. Rep. 474, 1–75 (2009).
Svetlichny, G. Distinguishing three-body from two-body nonseparability by a Bell-type inequality. Phys. Rev. D 35, 3066–3069 (1987).
Zukowski, M., Zeilinger, A., Horne, M. A. & Weinfurter, H. Quest for GHZ states. Acta Phys. Pol. 93, 187–195 (1998).
Acknowledgements
The authors thank D. Hamel and T. Bergmann for technical discussions, J. Dengis and C. Holloway for assistance in the laboratory, M. Ditty, F. Hamdullahpur, D. Huber, D. Parent, R. Zalagenas, M. Lazaridis and G. Dixon for their support in gaining access to the roof of RAC, P. Fulcher for safety training, A. Conrad, AGFA and C. Stewart for allowing access to private property, B. Zinger, G. Doyle, D. Copeland, R. Reger, B. Mill, T. Galloway and Z. Wang for carpentry and electronics, S. Payne at Leysop Ltd for assistance with Pockels cells, M. Morelli at UBNT.ca for technical advice on wireless networks, Roncare and UW plant operations for snow ploughing, and M. Seibel for towing. The authors acknowledge financial support from the National Sciences and Engineering Research Council (NSERC), Canada Research Chairs (CRC), Canada Foundation for Innovation (CFI), European Research Council (ERC, Project “EnSeNa”), Industry Canada, Canadian Institute for Advanced Research (CIFAR), Ontario Centres of Excellence (OCE) and QuantumWorks. R.P. acknowledges support from the Fonds zur Förderung der wissenschaftlichen Forschung (FWF, J2960-N20), Ministry of Research and Innovation Canada (MRI), the Vienna International Post-Doctoral (VIPS) Program of the Austrian Federal Ministry of Science and Research and the City of Vienna, as well as the European Commission (Marie Curie, FP7-PEOPLE-2011-IIF).
Author information
Authors and Affiliations
Contributions
C.E., R.L., G.W., T.J. and K.J.R. conceived the experiment. C.E., E.M.S., K.F., J.L., B.L.H., Z.Y., C.P., J.P.B., R.P., L.R. and N.G. constructed the experiment. B.L.H. and L.K.S. performed the space–time analysis. C.E., E.M.S., K.F., J.L., C.P. and J.P.B. collected the data. C.E. analysed the data. All authors contributed to writing the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary information
Supplementary information (PDF 753 kb)
Rights and permissions
About this article
Cite this article
Erven, C., Meyer-Scott, E., Fisher, K. et al. Experimental three-photon quantum nonlocality under strict locality conditions. Nature Photon 8, 292–296 (2014). https://doi.org/10.1038/nphoton.2014.50
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphoton.2014.50
This article is cited by
-
Breaking universal limitations on quantum conference key agreement without quantum memory
Communications Physics (2023)
-
Simple proof of security of the multiparty prepare and measure QKD
Quantum Information Processing (2022)
-
Challenging local realism with human choices
Nature (2018)
-
Detector-device-independent quantum secret sharing with source flaws
Scientific Reports (2018)
-
(Nearly) optimal P values for all Bell inequalities
npj Quantum Information (2016)