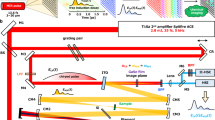
Abstract
Spectral measurements in the infrared optical range provide unique fingerprints of materials, which are useful for material analysis, environmental sensing and health diagnostics1. Current infrared spectroscopy techniques require the use of optical equipment suited for operation in the infrared range, components of which face challenges of inferior performance and high cost. Here, we develop a technique that allows spectral measurements in the infrared range using visible-spectral-range components. The technique is based on nonlinear interference of infrared and visible photons, produced via spontaneous parametric down conversion2,3. The intensity interference pattern for a visible photon depends on the phase of an infrared photon travelling through a medium. This allows the absorption coefficient and refractive index of the medium in the infrared range to be determined from the measurements of visible photons. The technique can substitute and/or complement conventional infrared spectroscopy and refractometry techniques, as it uses well-developed components for the visible range.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Stuart, B. H. Infrared Spectroscopy: Fundamentals and Applications (Wiley, 2004).
Zou, X. Y., Wang, L. J. & Mandel, L. Induced coherence and indistinguishability in optical interference. Phys. Rev. Lett. 67, 318–321 (1991).
Mandel, L. Quantum effects in one-photon and two-photon interference. Rev. Mod. Phys. 71, S274–S282 (1999).
Gisin, N., Ribordy, G., Tittel, W. & Zbinden, H. Quantum cryptography. Rev. Mod. Phys. 74, 145–195 (2002).
Gisin, N. & Thew, R. Quantum communication. Nature Photon. 1, 165–171 (2007).
Knill, E., Laflamme, R. & Milburn, G. J. A scheme for efficient quantum computation with linear optics. Nature 409, 46–52 (2001).
Ladd, T. D. et al. Quantum computers. Nature 464, 45–53 (2010).
Giovannetti, V., Lloyd, S. & Maccone, L. Advances in quantum metrology. Nature Photon. 5, 222–229 (2013).
Abadie, J. et al. A gravitational wave observatory operating beyond the quantum shot-noise limit. Nature Phys. 7, 962–965 (2011).
Klyshko, D. N. Photon and Nonlinear Optics (Gordon & Breach Science, 1988).
Burlakov, A. V. et al. Interference effects in spontaneous two-photon parametric scattering from two macroscopic regions. Phys. Rev. A 56, 3214–3225 (1997).
Korystov, D. Y., Kulik, S. P. & Penin, A. N. Rozhdestvenski hooks in two-photon parametric light scattering. J. Exp. Theor. Phys. Lett. 73, 214–218 (2001).
Kulik, S. P. et al. Two-photon interference in the presence of absorption. J. Exp. Theor. Phys. 98, 31–38 (2004).
Lemos, G. B. et al. Quantum imaging with undetected photons. Nature 512, 409–412 (2014).
Hudelist, F. et al. Quantum metrology with parametric amplifier-based photon correlation interferometers. Nature Commun. 5, 3049 (2014).
Chen, B. et al. Atom–light hybrid interferometer. Phys. Rev. Lett. 115, 043602 (2015).
Polivanov, Y. N. Raman scattering of light by polariton. Sov. Phys. Usp. 21, 805–831 (1978).
Heilweil, E. J. Ultrashort-pulse multichannel infrared spectroscopy using broadband frequency conversion in LiIO3 . Opt. Lett. 14, 551–553 (1989).
Dougherty, T. P. & Heilwell, E. J. Dual-beam subpicosecond broadband infrared spectrometer. Opt. Lett. 19, 129–131 (1994).
Klyshko, D. N. Ramsey interference in two-photon parametric scattering. J. Exp. Theor. Phys. 77, 222–226 (1993).
Belinsky, A. V. & Klyshko, D. N. Interference of classical and non-classical light. Phys. Lett. A 166, 303–307 (1992).
Bideau-Mehu, A., Guern, Y., Abjean, R. & Johannin-Gilles, A. Interferometric determination of the refractive index of carbon dioxide in ultraviolet region. Opt. Commun. 9, 432–434 (1973).
Heineken, F. W. & Battaglia, A. Absorption and refraction of ammonia as a function of pressure at 6 mm wavelength. Physica 24, 589–603 (1958).
Burch, D. E. & Williams, D. Total absorptance by nitrous oxide bands in the infrared. Appl. Opt. 1, 473–482 (1962).
Tonouchi, M. Cutting-edge terahertz technology. Nature Photon. 1, 97–105 (2007).
Kailash, C. J., Covert, P. A. & Hore, D. K. Phase measurement in nondegenerate three-wave mixing spectroscopy. J. Chem. Phys. 134, 044712 (2011).
Acknowledgements
This work was supported by DSI core funds within the framework of the Quantum Sensors programme. The authors thank G. Vienne, R. Bakker, G. Maslennikov and D. Kupriyanov for discussions and advice on the experiment.
Author information
Authors and Affiliations
Contributions
D.A.K. and L.A.K. assembled the experimental set-up and conducted the measurements. A.V.P. analysed the data and carried out numerical simulations. L.A.K. and S.P.K. conceived the idea and designed the experiment. All authors contributed to preparation of the manuscript.
Corresponding author
Ethics declarations
Competing interests
A.V.P., D.A.K. and L.A.K. are listed as inventors for a provisional patent application on the method described in Supplementary Section 1.
Supplementary information
Supplementary information
Supplementary information (PDF 419 kb)
Supplementary information
Supplementary figure (GIF 1042 kb)
Rights and permissions
About this article
Cite this article
Kalashnikov, D., Paterova, A., Kulik, S. et al. Infrared spectroscopy with visible light. Nature Photon 10, 98–101 (2016). https://doi.org/10.1038/nphoton.2015.252
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphoton.2015.252