Abstract
A fundamental challenge for quantum information processing is reducing the impact of environmentally induced errors. Here we demonstrate a quantum error detection and rejection protocol based on the idea of quantum uncollapsing, using this protocol to reduce the impact of energy relaxation owing to the environment in a three-qubit superconducting circuit. We encode quantum information in a target qubit, and use the other two qubits to detect and reject errors caused by energy relaxation. This protocol improves the storage time of a quantum state by a factor of roughly three, at the cost of a reduced probability of success. This constitutes the first experimental demonstration of the algorithm-based improvement in the lifetime of a quantum state stored in a qubit.
Similar content being viewed by others
Introduction
Superconducting quantum circuits are very promising candidates for building a quantum processor owing to the combination of good qubit performance and the scalability of planar integrated circuits1,2,3,4,5,6,7,8,9,10. In addition to recent, very significant improvements in the materials and qubit geometries in such circuits, external control and measurement protocols are being developed to improve performance. This includes the use of dynamical decoupling11, measurement-based feedback controls12,13 and preliminary experiments14 with quantum error correction (QEC) codes, which allow the removal of artificially induced errors14,15,16,17,18. To date, however, there has been little experimental progress in control sequences that reduce a significant source of qubit error: energy dissipation due to the environment.
Although there are QEC proposals to correct intrinsic dissipation errors18,19, these have not yet been demonstrated experimentally, mostly due to gate fidelity limitations. Quantum error detection and rejection (QEDR)20,21 provide an alternative, albeit non-deterministic approach to handling errors, avoiding some of the complexity of full QEC by simply rejecting errors when they are detected. Using a variant of quantum uncollapsing that combines a weak measurement with its reversal22,23,24,25, QEDR has been predicted to significantly reduce the impact of energy relaxation in qubits21, one of the dominant sources of error in superconducting quantum circuits1,2,3.
The QEDR protocol based on uncollapsing22 is illustrated in Fig. 1a. Starting with a qubit in a superposition of its ground |g› and excited |e› states, |ψi›=α|g›+β|e›, a weak measurement is performed that detects the |e› state with probability (measurement strength) p<1. In the null-measurement outcome (|e› state not detected), this produces the partially collapsed state |ψ1›=α|g›+β |e› (the squared norm equals the outcome probability). The system is then stored for a time τ, during which it can decay (jump) to the |g› state or remain in the no-jump state |ψnj›=α|g›+β
|e› (the squared norm equals the outcome probability). The system is then stored for a time τ, during which it can decay (jump) to the |g› state or remain in the no-jump state |ψnj›=α|g›+β e−Γτ/2|e›, where Γ=1/T1 is the energy relaxation rate. (Instead of using the master equation formalism for the density matrix to account for the energy relaxation, here we prefer to use the Kraus operator-based jump and no-jump scenarios, as this gives more physical insight. See Supplementary Notes 2 and 3, and elsewhere21,22 for details.) The uncollapsing measurement is then performed, comprising a πx rotation and a second weak measurement with strength pu, followed by a final πx rotation that undoes the first rotation. Only outcomes that yield a second null measurement are kept. These double-null outcomes give the result |
e−Γτ/2|e›, where Γ=1/T1 is the energy relaxation rate. (Instead of using the master equation formalism for the density matrix to account for the energy relaxation, here we prefer to use the Kraus operator-based jump and no-jump scenarios, as this gives more physical insight. See Supplementary Notes 2 and 3, and elsewhere21,22 for details.) The uncollapsing measurement is then performed, comprising a πx rotation and a second weak measurement with strength pu, followed by a final πx rotation that undoes the first rotation. Only outcomes that yield a second null measurement are kept. These double-null outcomes give the result | ›=|g› if the system jumped to |g› during the time interval τ, while in the no-jump case, the final state is
›=|g› if the system jumped to |g› during the time interval τ, while in the no-jump case, the final state is
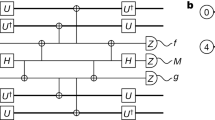
(a) Quantum uncollapsing protocol in the phase qubit22,23. Top: pulse sequence, where the weak measurement with strength p is followed by a delay (storage time) τ, and then the measurement reversal, involving a πx rotation, a weak measurement with strength pu, and a second πx rotation. Bottom: the delta-like electrical pulses lower the tunnel barrier for the qubit states on the left of the potential landscape to allow partial tunnelling of the |e state into the well on the right. (b,c) Optical micrograph and simplified schematic of the device. Circuit elements are as labelled; those not used in this experiment are in grey. (d) Illustration of the qubit–resonator–qubit (QRQ) swap, analogous to the partial tunnelling measurement. Left: schematic for the sequential qubit Q1-resonator B swap with swap probability (measurement strength) p (pu), followed by a full iSWAP between resonator B and qubit Q2 (Q3). Right: the on-resonance, unit-amplitude qubit-resonator vacuum Rabi oscillations in the qubit |e
state into the well on the right. (b,c) Optical micrograph and simplified schematic of the device. Circuit elements are as labelled; those not used in this experiment are in grey. (d) Illustration of the qubit–resonator–qubit (QRQ) swap, analogous to the partial tunnelling measurement. Left: schematic for the sequential qubit Q1-resonator B swap with swap probability (measurement strength) p (pu), followed by a full iSWAP between resonator B and qubit Q2 (Q3). Right: the on-resonance, unit-amplitude qubit-resonator vacuum Rabi oscillations in the qubit |e state probability Pe (vertical axis), starting with the qubit in |e
state probability Pe (vertical axis), starting with the qubit in |e and resonator in |0
and resonator in |0 . The measurement strength p=1−Pe is set by the interaction time (horizontal axis). (e) QEDR protocol, where we start with Q1 in |ψi
. The measurement strength p=1−Pe is set by the interaction time (horizontal axis). (e) QEDR protocol, where we start with Q1 in |ψi , consisting of the following steps: (1) the first weak measurement is performed using the first QRQ swap involving Q1–B–Q2, with strength p. Q2 is measured immediately, and only null outcomes (Q2 in |g
, consisting of the following steps: (1) the first weak measurement is performed using the first QRQ swap involving Q1–B–Q2, with strength p. Q2 is measured immediately, and only null outcomes (Q2 in |g ) are accepted. (2) The state is swapped from Q1 into memory resonator M1 and stored for a relatively long time τ2, following which the state is swapped back into Q1. (3) The weak measurement reversal is performed using a πx rotation on Q1 and a second QRQ swap with strength pu to qubit Q3. Q3 is then measured, and only null outcomes (Q3 in |g
) are accepted. (2) The state is swapped from Q1 into memory resonator M1 and stored for a relatively long time τ2, following which the state is swapped back into Q1. (3) The weak measurement reversal is performed using a πx rotation on Q1 and a second QRQ swap with strength pu to qubit Q3. Q3 is then measured, and only null outcomes (Q3 in |g ) are accepted. (4) The double-null outcomes are analysed using tomography of Q1 to evaluate Q1’s final density matrix. To save time and reduce errors, we do not perform the final πx rotation appearing in the full uncollapsing protocol.
) are accepted. (4) The double-null outcomes are analysed using tomography of Q1 to evaluate Q1’s final density matrix. To save time and reduce errors, we do not perform the final πx rotation appearing in the full uncollapsing protocol.

Remarkably, the final no-jump state is identical to |ψi› if we choose 1−pu=(1−p)e−Γτ; the probability of this (desired) outcome is  =‹
=‹ |
| ›=(1−p)e−Γτ, while the probability of the undesirable jump outcome |g› is
›=(1−p)e−Γτ, while the probability of the undesirable jump outcome |g› is  =|β|2(1−p)2e−Γτ(1−e−Γτ). These two probabilities do not add to one; the remaining probability covers situations other than these double-null measurement outcomes. As the probability Pfj falls to zero more quickly than
=|β|2(1−p)2e−Γτ(1−e−Γτ). These two probabilities do not add to one; the remaining probability covers situations other than these double-null measurement outcomes. As the probability Pfj falls to zero more quickly than  as p→1, increasing the measurement strength p towards 1 results in a higher likelihood of recovering the initial state. This comes at the expense of a low probability PDN=
as p→1, increasing the measurement strength p towards 1 results in a higher likelihood of recovering the initial state. This comes at the expense of a low probability PDN= +
+ of the double-null result. The resulting density matrix is ρf=(
of the double-null result. The resulting density matrix is ρf=( |
| ›‹
›‹ |+
|+ |g›‹g|)/PDN.
|g›‹g|)/PDN.
The QEDR protocol in Fig. 1a relies on partial tunnelling to perform the weak measurement. We have performed the protocol in this way, but found that this gave low fidelities. We therefore, in addition, implemented a weak measurement using a partial swap between the target qubit and an ancilla qubit, followed by a projective measurement of the ancilla; the two weak measurements in the QEDR protocol thus required two ancilla qubits. Using this alternative measurement, here we show that a quantum state suffering from dissipation errors can be almost fully recovered, although only by rejecting a large fraction of the measurement outcomes. This allowed us to extend the intrinsic lifetime of a quantum state by a factor of about three. A somewhat similar protocol has been demonstrated with photonic qubits, but only to suppress intentionally generated errors26.
Results
Implementation of the weak measurement
The device we used to implement the QEDR protocol is similar to that in Lucero et al.27 (shown in Fig. 1b,c), with three phase qubits, Q1, Q2 and Q3, coupled to a common, half-wavelength coplanar waveguide bus resonator B, with a memory resonator M1 also coupled to Q1. Relevant parameters are tabulated in Supplementary Table 1 (see Supplementary Note 1 for sample fabrication details).
The partial measurement method is illustrated in Fig. 1d. Qubit Q1 is the target, and Q2 and Q3 are ancillae, entangled with Q1 via the resonator bus B, such that a projective measurement of Q2 or Q3 results in a weak measurement of Q1. The entanglement begins with a partial swap between Q1 and the resonator B: When qubit Q1, initially in |e›, is tuned to resonator B, the probability Pe of finding the qubit in |e› oscillates with unit amplitude at the vacuum Rabi frequency28,29,30. A partial swap with swap probability p=1−Pe is achieved by controlling the interaction time, entangling Q1 and B. We then use a complete swap (an iSWAP) between resonator B and qubit Q2 (Q3), transferring the entanglement, followed by a projective measurement of Q2 (Q3). In general, we start with Q1 in |ψi›=α|g›+β|e› and perform the qubit–resonator–qubit (QRQ) swap, followed by measurement of the ancilla. A null outcome (Q2 or Q3 in |g›) yields the Q1 state α|g›+β |e›, as with partial tunnelling. The swap probability p is therefore equivalent to the measurement strength.
|e›, as with partial tunnelling. The swap probability p is therefore equivalent to the measurement strength.
Full QEDR sequence
Our QEDR protocol can protect against energy decay of the quantum state. However, as dephasing in these qubits is an important error source, against which the QEDR protocol does not protect, we store the intermediate quantum state in the memory resonator M1, which does not suffer from dephasing (as indicated by T2≅2T1 for the resonator; see Supplementary Table 1).
Our full QEDR protocol is thus shown in Fig. 1e, starting with the initial state of the system as

where |q1q2q3› represents the state of the qubits Q1, Q2 and Q3, with the ground state |00› of the B and M1 resonators listed last. In step 1, we use a QRQ swap between Q1, B and Q2 with swap probability (measurement strength) p, followed immediately by measurement of Q2. This step takes a time τ1 of up to 15 ns, depending on p. A null outcome (Q2 in |g›) yields |ψ1›=α|ggg›|00›+β |egg›|00› (a more precise expression appears in Supplementary Note 2). In step 2, we swap the quantum state from Q1 into M1, wait a relatively long time τ=τ2, during which the state in M1 decays at a rate Γ=1/T1, and we then swap the state back to Q1. In the no-jump case, the state becomes |
|egg›|00› (a more precise expression appears in Supplementary Note 2). In step 2, we swap the quantum state from Q1 into M1, wait a relatively long time τ=τ2, during which the state in M1 decays at a rate Γ=1/T1, and we then swap the state back to Q1. In the no-jump case, the state becomes | ›=α|ggg›|00›+β
›=α|ggg›|00›+β |egg›|00›. We then perform step 3, comprising a πx rotation on Q1 followed by the second QRQ swap with strength pu, involving Q1, B and Q3, which takes a time τ3. τ3 is between 20 and 35 ns, depending on pu, dominated by the 20 ns-duration πx pulse. Q3 is then measured, with a null outcome (Q3 in |g›) corresponding to
|egg›|00›. We then perform step 3, comprising a πx rotation on Q1 followed by the second QRQ swap with strength pu, involving Q1, B and Q3, which takes a time τ3. τ3 is between 20 and 35 ns, depending on pu, dominated by the 20 ns-duration πx pulse. Q3 is then measured, with a null outcome (Q3 in |g›) corresponding to

We recover the initial state |ψi› if we set 1−pu=(1−p)e−Γτ2, with the undesired jump cases mostly eliminated by the double-null selection. To shorten the sequence, we do not perform the final πx rotation, so the amplitudes of Q1’s |g› and |e› states are reversed compared with the initial state. In step 4, we apply tomography pulses and then measure Q1 to determine its final state, keeping the results that correspond to the double-null outcomes (Q2 and Q3 in |g›).
Characterizing the QEDR performance
We use quantum process tomography to characterize the performance of the protocol, starting with the four initial states {|g›, |g›−i|e›, |g›+|e›, |e›} and measuring the one-qubit process matrix χ. As we reject outcomes, where Q2 and Q3 are not measured in |g›, the process is not trace preserving, so the linear map satisfies ρfPDN= , where ρi and ρf are the normalized initial and final density matrices of Q1, and En is the standard Pauli basis {I,X,Y,Z}. We define the process fidelity as31 =Tr(χidealχ)/Tr(χ), where χideal corresponds to the desired unitary operation (here given by πx), and the divisor accounts for postselection. There are other ways to define the process fidelity F; for instance, one can average the state fidelity over a set of pure initial states, either with or without weighting by the selection probability21,32. We have analysed the data using various definitions of the fidelity and found similar fidelity improvement due to QEDR for all of them. See Supplementary Note 4 for more details.
, where ρi and ρf are the normalized initial and final density matrices of Q1, and En is the standard Pauli basis {I,X,Y,Z}. We define the process fidelity as31 =Tr(χidealχ)/Tr(χ), where χideal corresponds to the desired unitary operation (here given by πx), and the divisor accounts for postselection. There are other ways to define the process fidelity F; for instance, one can average the state fidelity over a set of pure initial states, either with or without weighting by the selection probability21,32. We have analysed the data using various definitions of the fidelity and found similar fidelity improvement due to QEDR for all of them. See Supplementary Note 4 for more details.
We first tested the process with no storage, entirely omitting step 2 in Fig. 1e, and choosing pu=p; we also delayed the measurement of Q2 to the end of step 3 to minimize crosstalk (see Methods). Figure 2a shows the measured χ/Tr(χ) for p=pu=0.75; the calculated process fidelity is =0.92. In Fig. 2b, we show the measured process fidelity as a function of the QRQ measurement strength p=pu (blue circles).
(a) Measured χ/Tr(χ) (bars with colour), where χ is the non-trace-preserving quantum process tomography matrix for the sequence in Fig. 1e excluding step 2, here with p=pu=0.75. The desired matrix, χideal, corresponds to a π rotation about the Bloch sphere x axis (identified by black frames). (b) Process fidelity for both the three-qubit QRQ-based uncollapsing (blue circles) and the single-qubit partial-tunnelling version (red circles)23, both as a function of p=pu. Statistical errors are shown by error bars, defined as ±1 s.d., using the repeated sets of fidelity measurement. The process fidelity is above 0.9 for p≤0.8 using the QRQ swaps, while for the partial-tunnelling scheme it decreases significantly for p≥0.5. This decrease is primarily due to reduction in qubit T2 with measurement current bias, shown in the inset; partial tunnelling occurs in the shaded region. Blue line is a simulation using κ1=κ3=0.985, κ2=1, and κϕ=0.95 (see Supplementary Note 3); the red line is a guide to the eye.
We can compare our no-storage uncollapsing fidelity to that obtained using partial tunnelling for the weak measurement of a single qubit23, shown in Fig. 2b (red circles). We see that even though the QRQ-based protocol is more complex, it achieves much better fidelities for p≥0.5. This is mostly because of strong dephasing and two-level state effects4,29 during the partial tunnelling current pulse (see inset in Fig. 2b).
Protection from energy relaxation
We then tested the full QRQ protocol’s ability to protect from energy decay. The uncollapsing strength pu is given by22 1−pu=(1−p)κ1κ2/κ3, where κ2=exp(−τ2/T1), and κ1 and κ3 are similar energy relaxation factors for the steps 1 and 3 (here κ1≈κ3≈0.985; see Supplementary Note 3). In Fig. 3a, we display the measured fidelities for the storage durations τ2=0.9, 1.7 and 3 μs for the memory resonator with T1=2.5 μs, compared with simulations using the pure dephasing factor κϕ=0.95 (see Korotkov and Keane22 and Supplementary Note 3). The simulations are in excellent agreement with the data, and we see a marked improvement in the storage fidelity using QEDR over that of free decay (dashed line in each panel).
(a) Process fidelity as a function of measurement strength p for the full QEDR protocol for three storage times τ2=0.9, 1.7 and 3 μs in a memory resonator M1 (T1=2.5 μs). The uncollapsing swap probability pu is indicated on the top axis (see text). Circles with error bars are measured data; lines are simulations (see Supplementary Note 3). Horizontal dashed lines in each panel give the free-decay process fidelity; the improvement from QEDR is most significant for larger τ2. Error bars represent statistical errors (s.d.). These statistical errors increase with increasing QRQ measurement strength p, mainly due to the decrease in sample size (fewer double-null outcomes), while errors due to uncertainties in pu are less than 10% of those shown in the figure (see Supplementary Note 3); we compensate for dynamic phases (see Supplementary Note 3). (b) Final density matrices (bars with colour) without (top row) and with (bottom row) QEDR, with p=0.75, for the four initial states as labelled, following a τ2=3 μs storage time ( =0.3). The desired error-free density matrices are shown by black frames. We only display the absolute values of the density matrix elements |ρ|. Note that the QEDR-protected final states differ from the initial state by a π rotation.
=0.3). The desired error-free density matrices are shown by black frames. We only display the absolute values of the density matrix elements |ρ|. Note that the QEDR-protected final states differ from the initial state by a π rotation.
It is interesting to note that in Fig. 3a, the process fidelity is significantly improved even for zero measurement strength p=0 (note that pu >0), implying that a simpler QEDR protocol still provides some protection against energy relaxation.
Another way to test QEDR is to monitor the evolution of individual quantum states. In Fig. 3b, we display the final density matrices measured either without (top row) or with (bottom row) QEDR, for four initial states in Q1, with storage in the memory M1 for τ2≈3 μs. Other than for the initial ground state |g›, which does not decay, we see that the QEDR-protected states are much closer to the desired outcomes than the free-decay states (note the π rotation). If we look at the off-diagonal terms in the middle panels, they have decayed from 0.5 to about 0.4; this decay takes about 1.1 μs without QEDR, so the lifetime is increased by 3 μs/1.1 μs≈3. Also, if we look at Fig. 3a, the free-decay fidelity at 0.9 μs (left panel) is about the same as the maximum QEDR fidelity at 3.0 μs (right panel), also giving a factor of three improvement.
Discussion
The price paid for the lifetime improvement is the small fraction of outcomes accepted by the QEDR postselection, shown in Fig. 4. The double-null probability PDN decreases with increasing measurement strength p for all initial states. A balance must therefore be struck between a larger T1 improvement, occurring for larger p, and a larger fraction of accepted outcomes, which occurs for smaller p.
The QEDR protocol uses postselection to reject state decay errors. The probability of accepting an outcome, that is, the double-null probability PDN, falls with measurement strength p. Here we display PDN as a function of p, corresponding to the data in Fig. 3a, for each value of storage time τ2. Error bars represent s.d. of repeated measurement results; error bars that are smaller than the symbol size are omitted for clarity. Lines are predicted by theory.
QEDR will be challenging to implement in large-scale qubit circuits, as it does not scale well, in particular when the fraction of successful outcomes is small; QEC, when it becomes an experimental reality, will clearly provide a far more efficient route to fault-tolerance, even though it requires significantly more resources (qubits and gates) than does QEDR (see Supplementary Note 5). However, the simplest QEC proposal for energy relaxation19 is expected to only yield a factor of about two improvement in the state lifetime (assuming gate execution is almost perfect), which is less than the factor of three improvement demonstrated here using QEDR. The simpler QEDR protocol can therefore serve as a suitable intermediate to QEC, applicable to small and medium scale quantum circuits.
Methods
Readout correction and crosstalk cancellation
All data are corrected for the qubit readout fidelities before further processing. The readout fidelities for |g› (Fg) and |e› (Fe) of Q1, Q2 and Q3 are F1g=0.95, F1e=0.89, F2g=0.94, F2e=0.88, F3g=0.94, F3e=0.91, respectively. Crosstalk is another concern when performing QEDR to protect quantum states. We read out Q2 immediately after the first QRQ swap in step 1 in Fig. 1e to avoid decay in Q2. However, due to measurement crosstalk in the qubit circuit, this measurement can result in excitations in resonator B; while this does not directly affect the other qubits, we must reset the resonator prior to the second QRQ swap. This is done during the storage in the memory resonator by performing a swap between B and Q3, and then using a spurious two-level defect coupled to Q3 to erase the excitation in Q3. As the storage time in M1 is several microseconds, there is sufficient time to reset both B and Q3 prior to the second QRQ swap.
The intermediate reset of B could not be performed when doing the experiments in Fig. 2, for which there is no storage interval. To avoid crosstalk in those measurements, we postponed the measurement of Q2 until the end of the second QRQ sequence to step 3 of Fig. 1e. The |e› state probability in Q2 drops by about 6% during this delay time, as estimated from Q2’s T1. We have corrected for this drop when evaluating the Q2 measurements for Fig. 2.
Additional information
How to cite this article: Zhong, Y. P. et al. Reducing the impact of intrinsic dissipation in a superconducting circuit by quantum error detection. Nat. Commun. 5:3135 doi: 10.1038/ncomms4135 (2014).
References
You, J. & Nori, F. Superconducting circuits and quantum information. Phys. Today 58, 42–47 (2005).
Clarke, J. & Wilhelm, F. K. Superconducting quantum bits. Nature 453, 1031–1042 (2008).
Devoret, M. H. & Schoelkopf, R. J. Superconducting circuits for quantum information: an outlook. Science 339, 1169–1174 (2013).
Sun, G. et al. Tunable quantum beam splitters for coherent manipulation of a solid-state tripartite qubit system. Nat. Commun. 1, 51 (2010).
Niskanen, A. O. et al. Quantum coherent tunable coupling of superconducting qubits. Science 316, 723–726 (2011).
Mariantoni, M. et al. Implementing the quantum von neumann architecture with superconducting circuits. Science 334, 61–65 (2011).
Abdumalikov, A. A. Jr et al. Experimental realization of non-Abelian non-adiabatic geometric gates. Nature 496, 482–485 (2013).
Paik, H. et al. Observation of high coherence in Josephson junction qubits measured in a three-dimensional circuit QED architecture. Phys. Rev. Lett. 107, 240501 (2011).
Rigetti, C. et al. Superconducting qubit in a waveguide cavity with a coherence time approaching 0.1 ms. Phys. Rev. B 86, 100506 (2012).
Barends, R. et al. Coherent Josephson qubit suitable for scalable quantum integrated circuits. Phys. Rev. Lett. 111, 080502 (2013).
Bylander, J. et al. Noise spectroscopy through dynamical decoupling with a superconducting flux qubit. Nat. Phys. 7, 565–570 (2011).
Ristè, D., Bultink, C. C., Lehnert, K. W. & DiCarlo, L. Feedback control of a solid-state qubit using high-fidelity projective measurement. Phys. Rev. Lett. 109, 240502 (2012).
Vijay, R. et al. Stabilizing Rabi oscillations in a superconducting qubit using quantum feedback. Nature 490, 77–80 (2012).
Reed, M. D. et al. Realization of three-qubit quantum error correction with superconducting circuits. Nature 482, 382–385 (2012).
Shor, P. W. Scheme for reducing decoherence in quantum computer memory. Phys. Rev. A 52, R2493–R2496 (1995).
Schindler, P. et al. Experimental repetitive quantum error correction. Science 332, 1059–1061 (2011).
Yao, X. C. et al. Experimental demonstration of topological error correction. Nature 482, 489–494 (2012).
Leung, D. W., Nielsen, M. A., Chuang, I. L. & Yamamoto, Y. Approximate quantum error correction can lead to better codes. Phys. Rev. A 56, 2567–2573 (1997).
Leghtas, Z. et al. Hardware-efficient autonomous quantum memory protection. Phys. Rev. Lett. 111, 120501 (2013).
Knill, E. Quantum computing with realistically noisy devices. Nature 434, 39–44 (2005).
Keane, K. & Korotkov, A. N. Simplified quantum error detection and correction for superconducting qubits. Phys. Rev. A 86, 012333 (2012).
Korotkov, A. N. & Keane, K. Decoherence suppression by quantum measurement reversal. Phys. Rev. A 81, 040103(R) (2010).
Katz, N. et al. Reversal of the weak measurement of a quantum state in a superconducting phase qubit. Phys. Rev. Lett. 101, 200401 (2008).
Korotkov, A. N. & Jordan, A. N. Undoing a weak quantum measurement of a solid-state qubit. Phys. Rev. Lett. 97, 166805 (2006).
Sun, Q., Al-Amri, M. & Zubairy, M. S. Reversing the weak measurement of an arbitrary field with finite photon number. Phys. Rev. A 80, 033838 (2009).
Kim, Y.-S., Lee, J.-C., Kwon, O. & Kim, Y.-H. Protecting entanglement from decoherence using weak measurement and quantum measurement reversal. Nat. Phys. 8, 117–120 (2012).
Lucero, E. et al. Computing prime factors with a Josephson phase qubit quantum processor. Nat. Phys. 8, 719–723 (2012).
Hofheinz, M. et al. Synthesizing arbitrary quantum states in a superconducting resonator. Nature 459, 546–549 (2009).
Wang, Z. L. et al. Quantum state characterization of a fast tunable superconducting resonator. Appl. Phys. Lett. 102, 163503 (2013).
Amri, M. A., Scully, M. O. & Zubairy, M. S. Reversing the weak measurement on a qubit. J. Phys. B 44, 165509 (2011).
Kiesel, N., Schmid, C., Weber, U., Ursin, R. & Weinfurter, H. Linear optics controlled-phase gate made simple. Phys. Rev. Lett. 95, 210505 (2005).
Pedersen, L. H., Moller, N. M. & Molmer, K. Fidelity of quantum operations. Phys. Lett. A 367, 47–51 (2007).
Acknowledgements
This work was supported by the National Basic Research Program of China (2012CB927404, 2014CB921201), the National Natural Science Foundation of China (11222437, 11174248, and J1210046), Zhejiang Provincial Natural Science Foundation of China (LR12A04001), IARPA/ARO grant W911NF-10-1-0334, and ARO MURI grant W911NF-11-1-0268. H.W. acknowledges support by Program for New Century Excellent Talents in University (NCET-11-0456). Devices were made at the UC Santa Barbara Nanofabrication Facility, a part of the NSF-funded National Nanotechnology Infrastructure Network.
Author information
Authors and Affiliations
Contributions
Y.P.Z., A.N.K. and H.W. designed and analysed the experiment carried out by Y.P.Z. All authors contributed to the experimental set-up and helped to write the paper.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Figure 1, Supplementary Table S1, Supplementary Notes 1-5 and Supplementary References (PDF 151 kb)
Rights and permissions
About this article
Cite this article
Zhong, Y., Wang, Z., Martinis, J. et al. Reducing the impact of intrinsic dissipation in a superconducting circuit by quantum error detection. Nat Commun 5, 3135 (2014). https://doi.org/10.1038/ncomms4135
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/ncomms4135
This article is cited by
-
Optical tomography dynamics induced by qubit-resonator interaction under intrinsic decoherence
Scientific Reports (2022)
-
Dynamics of Correlations in the Presences of Intrinsic Decoherence
International Journal of Theoretical Physics (2016)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.