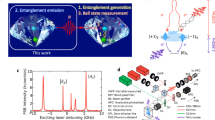
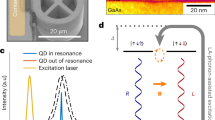
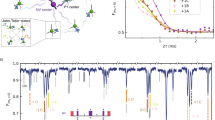
Abstract
Quantum entanglement is among the most fascinating aspects of quantum theory1. Entangled optical photons are now widely used for fundamental tests of quantum mechanics2 and applications such as quantum cryptography1. Several recent experiments demonstrated entanglement of optical photons with trapped ions3, atoms4,5 and atomic ensembles6,7,8, which are then used to connect remote long-term memory nodes in distributed quantum networks9,10,11. Here we realize quantum entanglement between the polarization of a single optical photon and a solid-state qubit associated with the single electronic spin of a nitrogen vacancy centre in diamond. Our experimental entanglement verification uses the quantum eraser technique5,12, and demonstrates that a high degree of control over interactions between a solid-state qubit and the quantum light field can be achieved. The reported entanglement source can be used in studies of fundamental quantum phenomena and provides a key building block for the solid-state realization of quantum optical networks13,14.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Nielsen, M. A. & Chuang, I. L. Quantum Computation and Quantum Information (Cambridge Univ. Press, 2000)
Aspect, A., Grangier, P. & Roger, G. Experimental realization of Einstein-Podolsky-Rosen-Bohm Gedankenexperiment: a new violation of Bell's inequalities. Phys. Rev. Lett. 49, 91–94 (1982)
Blinov, B. B., Moehring, D. L., Duan, L. M. & Monroe, C. Observation of entanglement between a single trapped atom and a single photon. Nature 428, 153–157 (2004)
Volz, J. et al. Observation of entanglement of a single photon with a trapped atom. Phys. Rev. Lett. 96, 030404 (2006)
Wilk, T., Webster, S. C., Kuhn, A. & Rempe, G. Single-atom single-photon quantum interface. Science 317, 488–490 (2007)
Yuan, Z.-S. et al. Experimental demonstration of a BDCZ quantum repeater node. Nature 454, 1098–1101 (2008)
Matsukevich, D. et al. Entanglement of a photon and a collective atomic excitation. Phys. Rev. Lett. 95, 040405 (2005)
Sherson, J. F. et al. Quantum teleportation between light and matter. Nature 443, 557–560 (2006)
Cabrillo, C., Cirac, J. I., Garcia-Fernandez, P. & Zoller, P. Creation of entangled states of distant atoms by interference. Phys. Rev. A 59, 1025–1033 (1999)
Chou, C. W. et al. Measurement-induced entanglement for excitation stored in remote atomic ensembles. Nature 438, 828–832 (2005)
Moehring, D. L. et al. Entanglement of single-atom quantum bits at a distance. Nature 449, 68–71 (2007)
Scully, M. O. & Drühl, K. Quantum eraser: a proposed photon correlation experiment concerning observation and “delayed choice” in quantum mechanics. Phys. Rev. A 25, 2208–2213 (1982)
Kimble, H. J. The quantum internet. Nature 453, 1023–1030 (2008)
Childress, L., Taylor, J. M., Sørensen, A. S. & Lukin, M. D. Fault-tolerant quantum communication based on solid-state photon emitters. Phys. Rev. Lett. 96, 070504 (2006)
Duan, L.-M. & Monroe, C. Robust quantum information processing with atoms, photons, and atomic ensembles. Adv. At. Mol. Opt. Phys. 55, 419–464 (2008)
Neumann, P. et al. Multipartite entanglement among single spins in diamond. Science 320, 1326–1329 (2008)
Ansmann, M. et al. Violation of Bell's inequality in Josephson phase qubits. Nature 461, 504–506 (2009)
DiCarlo, L. et al. Demonstration of two-qubit algorithms with a superconducting quantum processor. Nature 460, 240–244 (2009)
de Riedmatten, H., Afzelius, M., Staudt, M. U., Simon, C. & Gisin, N. A solid-state light-matter interface at the single-photon level. Nature 456, 773 (2008)
Balasubramanian, G. et al. Ultralong spin coherence time in isotopically engineered diamond. Nature Mater. 8, 383–387 (2009)
Fuchs, G. D., Dobrovitski, V. V., Toyli, D. M., Heremans, F. J. & Awschalom, D. D. Gigahertz dynamics of a strongly driven single quantum spin. Science 326, 1520–1522 (2009)
Dutt, M. V. G. et al. Quantum register based on individual electronic and nuclear spin qubits in diamond. Science 316, 1312–1316 (2007)
Tamarat, P. et al. Spin-flip and spin-conserving optical transitions of the nitrogen-vacancy centre in diamond. N. J. Phys. 10, 045004 (2008)
Manson, N., Harrison, J. & Sellars, M. Nitrogen-vacancy center in diamond: model of the electronic structure and associated dynamics. Phys. Rev. B 74, 104303 (2006)
Santori, C. et al. Coherent population trapping of single spins in diamond under optical excitation. Phys. Rev. Lett. 97, 247401 (2006)
Kaiser, F. et al. Polarization properties of single photons emitted by nitrogen-vacancy defect in diamond at low temperature. 〈http://arXiv.org/abs/0906.3426〉 (2009)
Englund, D., Faraon, A., Fushman, I., Stoltz, N. & Petroff, P. Controlling cavity reflectivity with a single quantum dot. Nature 450, 857–861 (2007)
Schietinger, S., Schröder, T. & Benson, O. One-by-one coupling of single defect centers in nanodiamonds to high-Q modes of an optical microresonator. Nano Lett. 8, 3911–3915 (2008)
Wang, C. F. et al. Fabrication and characterization of two-dimensional photonic crystal microcavities in nanocrystalline diamond. Appl. Phys. Lett. 91, 201112 (2007)
Fleischhauer, M., Imamoğlu, A. & Marangos, J. P. Electromagnetically induced transparency: optics in coherent media. Rev. Mod. Phys. 77, 633–673 (2005)
Acknowledgements
We thank F. Jelezko, J. Wrachtrup, V. Jacques, N. Manson, J. Taylor and J. MacArthur for discussions and experimental help. This work was supported by the Defense Advanced Research Projects Agency, NSF, Harvard-MIT CUA, the NDSEG Fellowship and the Packard Foundation. The content of the information does not necessarily reflect the position or the policy of the US Government, and no official endorsements should be inferred.
Author information
Authors and Affiliations
Contributions
All authors contributed extensively to the work presented in this paper.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
This file contains 1 Supplementary Methods, 2 Level structure and polarization properties of the NV centre, 3 Spin readout, 4 Verification of polarization selection rules for A2 state, 5 Effects of magnetic environment, detunings, and echo, 6 Fidelity estimates, Supplementary Figures S1-S7 with legends and References. (PDF 1003 kb)
Rights and permissions
About this article
Cite this article
Togan, E., Chu, Y., Trifonov, A. et al. Quantum entanglement between an optical photon and a solid-state spin qubit. Nature 466, 730–734 (2010). https://doi.org/10.1038/nature09256
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/nature09256
This article is cited by
-
Radiative loss of coherence in free electrons: a long-range quantum phenomenon
Light: Science & Applications (2024)
-
Isotope engineering for spin defects in van der Waals materials
Nature Communications (2024)
-
Heralded entanglement between error-protected logical qubits for fault-tolerant distributed quantum computing
Science China Physics, Mechanics & Astronomy (2024)
-
Controlling single rare earth ion emission in an electro-optical nanocavity
Nature Communications (2023)
-
Dopant-assisted stabilization of negatively charged single nitrogen-vacancy centers in phosphorus-doped diamond at low temperatures
npj Quantum Information (2023)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.