Main

Currents of electrons moving on multiply connected paths are modulated by an applied magnetic flux with a period of h/e (ref. 1), as predicted by Aharonov and Bohm2. In superconducting rings, the order parameter also responds periodically to a magnetic flux, as London recognized when he analysed the implications of a single-valued superconducting wavefunction3; different condensate states, which differ by integer flux quanta, are related by a gauge transformation. London concluded that the flux periodicity in superconducting rings is h/e (ref. 3). He missed, however, a class of supercurrent-carrying wavefunctions, which were identified years later4,5,6, and enabled explanation of the experimentally observed h/2e flux quantization 7,8. Indeed, according to the Bardeen–Cooper–Schrieffer (BCS) theory of superconductivity9, the electronic condensate is formed by Cooper pairs, which carry twice the elementary charge. However, fundamentally it is not just the pairing motivated substitution of e by 2e, from which the periodicity in h/2e originates, but rather the subtle requirement of the degeneracy in energy 4,5,6 of the two distinct classes of supercurrent-carrying states.

The original flux trapping experiments7,8, which proved the h/2e flux quantization in superconductors, as well as the later experiments10,11,12 were considered a manifestation of the formation of Cooper pairs in the then known conventional superconductors. The discovery that magnetic flux changes the magnetization of YBa2Cu3O7-δ rings with a periodicity of h/2e was similarly argued to also provide evidence for Cooper pairs in high-temperature superconductors13.

Does, vice versa, the existence of Cooper pairs or the h/2e flux quantization necessarily imply an h/2e periodicity of the energy or the current in superconducting loops? In fact, the h/2e periodicity requires that multiply connected superconductors threaded by a flux n h/2e are degenerate in energy for different integers n. In superconducting s-wave rings or hollow cylinders with inner diameter d, this degeneracy occurs if d≫, where is the coherence length4,5,6. In the opposite regime, d≲, the discrete quantum nature of the electronic states in the ring matters and the energies at half-integer and integer flux quanta are generally different; correspondingly, the superconducting behaviour is only h/e periodic (see Fig. 8-8 in ref. 14). This behaviour should be observable, possibly in Al rings with d<=1.6 μm.

The oscillation period of energy and currents in superconducting rings is therefore not always h/2e. In fact, as we report here, the BCS theory strictly predicts that for rings of superconductors with nodes in their gap functions, such as the high-Tc cuprates, Sr2RuO4 or the heavy-fermion superconductors, the ground-state energy is generically h/e periodic. For all of these superconductors, the states that yield the BCS-condensate state also include current-carrying states with energies close to the Fermi energy EF. As a result of the magnetic-field-driven change of occupation of these states and the concomitant reconstruction of the condensate, the superconducting rings develop an h/e periodicity of the supercurrent.

The flux periodicity in mesoscopic loops of d-wave superconductors is contained in the solution of the Bogoliubov–de Gennes (BdG) equations15 for the pairing hamiltonian:

The operators cj σ (cj σ†) annihilate (create) an electron on lattice site j with spin σ=↑,↓; t is the hopping matrix element between nearest-neighbour sites, is the Peierls phase factor and A is the vector potential of the magnetic field. The order parameter of the superconducting state Δi j is defined on the links between neighbouring sites with phase factors appropriate for d-wave symmetry.

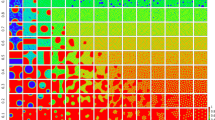
Figure 1 shows the probability density of the wavefunction for a state with energy close to EF on a square loop, whose edges are oriented parallel to the [100] and [010] directions, respectively. The d-wave loop eigenstates are obviously far more complex than the angular momentum eigenstates of a one-dimensional circular ring (see ref. 14), and the current flow in this loop can only be evaluated numerically. Nevertheless, a qualitative discussion also allows insight into the underlying physics.

Figure 1: Real-space representation of a square loop with a typical electronic probability density |Ψ|2 of a single state in the condensate.
figure 1

An eigenstate of the d-wave pairing hamiltonian, calculated for a square loop with 80×80 lattice sites with a pairing interaction of 0.3t. The hole in the centre has a size of 28×28 unit cells. To enhance the contrast of the complex pattern, the special colour code shown on the right is used and the discrete lattice points are smoothly interpolated.

Full size image

To assess the global quantities, namely, energy and current, the evolution of the eigenenergies with magnetic flux has to be calculated. The eigenstates with energies below EF form the ground-state condensate (Fig. 2). In the following, only flux values Φ between 0 and h/2e are discussed, because all quantities are either symmetric or antisymmetric with respect to flux reversal Φ→−Φ. Two clearly distinct regimes are found: the flux intervals between 0 and ≃h/4e and from ≃h/4e to h/2e.

Figure 2: Energy spectrum of the d-wave BCS model.
figure 2

The eigenenergies in the gap region are shown for a square 40×40 loop with a hole of 14×14 unit cells and pair interaction 0.3t as a function of flux Φ (in units of h/e). The energies are given in units of the superconducting order parameter Δ0 at Φ=0 (Δ0≈0.22t). The superconducting condensate consists of the states below EF=0 (red lines). Reconstruction of the condensate takes place near Φ=±(2n+1)h/4e, where the eigenenergies jump abruptly. The blue labels ‘A’ and ‘B’ refer to the current patterns shown in Fig. 4.

Full size image

Up to Φ≃h/4e, the supercurrent J generates a magnetic field that tends to compensate the applied field. This is achieved by a continuous shift of the eigenenergies in the condensate. At Φ=0, pairs of states with opposite circulation compensate their respective currents, and thus J=0. The well-separated states at Φ=0 in Fig. 2 are the states in the vicinity of the nodes of the mesoscopic d-wave superconductor. At energies further away from EF, the state density is higher; these are the states near the maximum energy gap that provide most of the condensation energy. For Φ>0, the energy of the states with orbital magnetic moment antiparallel (parallel) to the magnetic field is increased (decreased). Correspondingly, the supercurrent carried by these states depends on the details of level crossings and avoidings. The main contribution to the supercurrent arises from the occupied levels closest to EF, because the contributions from the lower-lying states tend to cancel in adjacent pairs.

As the highest occupied state shifts with increasing flux to lower energies, the current in the square loop first increases for small Φ (Fig. 3), then decreases, when the highest occupied level with an orbital moment opposite to the applied magnetic field starts to dominate. With increasing flux, this state approaches EF. For s-wave rings, this ‘Doppler shift energy’ (see ref. 15) corresponds to the critical value of the superfluid velocity, for which the indirect energy gap closes. For d-wave loops, the order parameter is protected by the numerous states that form the ‘lobes’ of the d-wave gap parameter.

Figure 3: Flux dependence of energy and current.
figure 3

a,b, Total energy (E(Φ)−E(0))/E(0) (a) and total circulating current J (b) for a square 40×40 loop with a hole of 14×14 unit cells and pair interaction 0.3t as a function of flux Φ in units of h/e. J is given in units of e t/ℏ=6×10−5A for a typical choice of t=250 meV. There is a clear difference between condensate states with an even and an odd winding number q of the order parameter, reflected, for example, in the deformation of the q=0 parabola. The overall Φ-periodicity for E and J is h/e.

Full size image

For d-wave loops and rings with other unconventional order parameter symmetries, the states in the vicinity of the nodes evolve with increasing flux as in small-gap s-wave rings. They do not necessarily cross EF (Fig. 2) owing the hybridization of the respective states above and below EF. Nevertheless, a state with one direction of current is replaced by a state of opposite direction (Fig. 4). The current-carrying states of the condensate are thereby continuously changing near the extrapolated crossing points. As a consequence, the energy ‘parabola’ centred at zero flux is different from the ground-state energy parabola centred at Φ=h/2e (Fig. 3). The deviation from a parabolic shape near zero flux is due to the evolution of the near-nodal states; the vertical offset of the energy minima at Φ=n h/e results mostly from the flux dependence of the states near the maximum value of the anisotropic gap.

Figure 4: Current distribution in a square loop of 40×40 lattice sites.
figure 4

The current expectation value of the occupied state closest to EF=0 is shown for flux Φ=0.17h/e (a; the state is marked with ‘A’ in Fig. 2) and for Φ=0.21h/e (b; marked with ‘B’ in Fig. 2). The colour encodes the projection of the current onto a square path around the loop whereby red presents an anticlockwise and blue a clockwise circulation. The maximal current is Jmax=0.15e t/ℏ for a, and 0.13e t/ℏ for b. The current distribution of each of the two states has strong spatial variations and does not fulfil the continuity condition which, however, is restored for the total current.

Full size image

For a flux value near h/4e the condensate reconstructs. The reconstructed superconducting state belongs to the class of wavefunctions introduced by Byers and Yang 4 in which, for a circular geometry, each pair increases its centre of mass angular momentum by ℏ (ref. 14). Remarkably, in the flux interval from near h/4e to h/2e, a full energy gap also exists for d-wave superconductors (Fig. 2). Here, the circulating current enhances the magnetic field; the paramagnetic moment of the current is parallel to the field. The resulting energy gain is responsible for the field-induced energy gap. This reconstruction of the condensate is the origin of the h/e periodicity in energy and current. Intriguingly, for superconductors with unconventional order parameter symmetries, larger loops (d≫) are also h/e periodic.

The numerical solution of the BdG equations with a self-consistency condition for the order parameter is adequate for ≃15 nm rings. However, to examine systems of micrometre size, the nodal states have to be described using a continuous gapless density of states. The flux induces a Doppler shift that modifies the states and alters their occupation near EF, thereby causing an h/e component of the current J. Whereas the h/2e component of J∝1/d, the h/e component decreases with 1/d2 (see the Supplementary Information). In quantitative agreement, the h/e component reduces by a factor of 60 on increasing the size of the ring, shown in Fig. 1, to the micrometre scale. Using typical parameter values for a yttrium barium copper oxide ring of 1 μm size, the ratio of the h/e versus the h/2e Fourier component remains in the per cent range. The frame width w of the ring has little influence on the weight of the h/e component for loops for which w is smaller than the penetration depth λ. A similar behaviour is also shown by loops with w>λ, because only states that result in the current-transport channels within λ significantly affect the h/e component.

Our calculations show that although changes in geometry, the number of transverse channels and elastic scattering by impurities, modify the J(Φ) characteristics in detail, they do not eliminate the h/e component. As long as the single-particle states are well defined, electronic correlation effects, which are responsible for the renormalization of states and of coupling parameters, are also not expected to have a strong influence on the discussed phenomena.

The robust magnetic-flux-induced presence of currents that flow opposite to the main screening currents affect many properties of unconventional superconductors. Of particular importance are a resulting enhancement of the London penetration depth and a weakening of the radiofrequency shielding. Furthermore, at any temperature, including T=0, the condensation energies, the screening current densities, the kinetic inductances and the penetration depths of rings of nodal superconductors are h/e periodic, the relative intensity of the h/e Fourier component decreasing with 1/d. The same properties are predicted for loops of s-wave superconductors with small gaps such as rings with diameters smaller than . These predictions are strict, free of fitting parameters and are therefore open to stringent experimental tests. The h/e periodicity of the supercurrent is a fundamental property of loops formed by unconventional superconductors.