Main

Earlier work9,10,11,12,13,14,15 on one-dimensional (1D) conduction in graphene ribbons (long and narrow ballistic strips) has shown that they may support a propagating mode arbitrarily close to the Dirac point, and that this mode lacks the valley degeneracy of modes that propagate at higher energies. For armchair edges of the ribbon, this lowest propagating mode is constructed from states in both valleys, but for zigzag edges only a single valley contributes9,10,11,12,13. In accord with time-reversal symmetry, the mode switches from one valley to the other on changing the direction of propagation.

Here, we consider a 2D geometry consisting of a quantum point contact (QPC) in a graphene sheet. A QPC is a short and narrow constriction with a quantized conductance G=n×2e2/h (ref. 16). (The factor of two accounts for the spin degeneracy.) A current, I, is passed through the QPC by application of a voltage difference, V, between the wide regions on opposite sides of the constriction (see Fig. 1). The orientation of the graphene lattice is such that the constriction has zigzag edges along the direction of current flow. We demonstrate by numerical simulation that on the first conductance plateau the QPC produces a strong polarization of the valleys in the wide regions. Our finding signifies that the two valleys in graphene can be addressed individually as independent internal degrees of freedom of the conduction electrons. This is only possible in a 2D geometry, because no well-separated valleys exist in 1D.

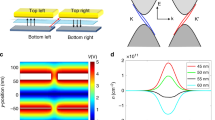
Figure 1: Schematic diagram of the valley filter.
figure 1

Middle panel: Honeycomb lattice of carbon atoms in a strip containing a constriction with zigzag edges. Top panel: Dispersion relation in the wide and narrow regions. An electron in the first valley (modes n=0,1,2,…) is transmitted (filled circle), whereas an electron in the second valley (modes n=−1,−2,…) is reflected (open circle). Bottom panel: Variation of the electrostatic potential along the strip, for the two cases of an abrupt and smooth potential barrier (solid and dashed lines). The polarity of the valley filter switches when the potential height, U0, in the constriction crosses the Fermi energy, EF.

Full size image

We show that the polarization of this valley filter can be inverted by locally raising the Dirac point in the region of the constriction, by means of a gate voltage, such that the Fermi level lies in the conduction bands in the wide regions and in the valence band inside the constriction. Two valley filters in series, one acting as a polarizer and the other as an analyser, can block the current if they have the opposite polarity (see Fig. 2), demonstrating that a QPC can operate as a ‘valley valve’—a purely electronic counterpart of the magneto-electronic spin valve. This extends to a 2D geometry the findings in a 1D geometry by Wakabayashi and Aoki12. We emphasize that their earlier work could not have demonstrated the selective population of a single valley—simply because valleys do not exist independently in 1D.

Figure 2: Schematic diagram of the valley valve (top) and corresponding potential profile (bottom).
figure 2

The current through the constriction is blocked if μL=EF−UL and μR=EF−UR have opposite signs.

Full size image

Our calculations start from the tight-binding model of graphene, with hamiltonian

The hopping matrix element τi j=−τ if the orbitals |i〉 and |j〉 are nearest neighbours on the honeycomb lattice, otherwise τi j=0. The electrostatic potential energy Ui=U(xi) varies only along the axis of the constriction. It equals in the wide regions and rises to U0 inside the constriction. We smooth the stepwise increase of the potential over a length Ls, according to the function

The potential barrier is rectangular for Ls=0 (solid line in Fig. 1, bottom panel), whereas it has a sinus shape for Ls=L (dashed line).

The dispersion relation of the honeycomb lattice in a strip with zigzag edges is shown schematically in Fig. 1 (top panel) and exactly in Fig. 3. The wide regions support 2N+1 propagating modes at the Fermi energy, EF, which form a basis for the transmission matrix, t. Modes n=1,2,…,N lie in the first valley (with longitudinal wavevector k a∈(π,2π)), whereas modes n=−1,−2,…,−N lie in the second valley (with k a∈(0,π)). The zeroth mode, n=0, lies in a single valley fixed by the direction of propagation. The conductance of the constriction is determined by the Landauer formula

The valley polarization of the transmitted current is quantified by

where we consider the case (illustrated in Fig. 1) that the zeroth mode lies in the first valley. The polarization P∈[−1,1], with P=1 if the transmitted current lies fully in the first valley and P=−1 if it lies fully in the second valley.

Figure 3: Dispersion relation of a graphene strip with zigzag edges.
figure 3

The spacing of the low-lying modes approaches for W/a≫1. The zeroth and first modes have a larger spacing, approaching 3Δ/2 for W/a≫1. The vertical lines mark the valley centres at k=2π/3a and 4π/3a.

Full size image

We have calculated the transmission matrix numerically by adapting to the honeycomb lattice the method developed by Ando for a square lattice17. The results are shown in Figs 4 and 5. We have fixed the width of the wide regions at (in units of the lattice spacing a). The electrochemical potential in the wide regions is set at , corresponding to 2N+1=29 propagating modes. The narrow region has width . We measure the electrochemical potential EF−U0≡μ0 in the narrow region in units of the mode spacing

(with being the energy-independent velocity in graphene). For our parameters Δ=πτ/40, as indicated in Fig. 3.

Figure 4: Conductance (top panel) and valley polarization (bottom panel) for the valley filter of Fig. 1, as a function of the electrochemical potential in the narrow region.
figure 4

The solid and dashed lines correspond to abrupt (Ls=0) and smooth (Ls=8a) potential steps, respectively. The inset in the bottom panel shows the degradation of the average valley polarization at μ0=0.25 Δ when a randomly chosen fraction, η, of sites at the edges of the constriction contain a vacancy.

Full size image
Figure 5: Conductance for the valley valve of Fig. 2 at fixed μL as a function of μR.
figure 5

The solid and dashed lines correspond to abrupt (Ls=0) and smooth (Ls=8a) potential steps, respectively.

Full size image

The operation of the valley filter is demonstrated in Fig. 4. The top panel shows the conductance, whereas the bottom panel shows the valley polarization—both as a function of the electrochemical potential, μ0, in the narrow region. For positive μ0, the current flows entirely within the conduction band, and we obtain plateaus of quantized conductance at odd multiples of 2e2/h (as predicted by Peres et al. 13). Smoothing of the potential step improves the flatness of the plateaus (compare the solid and dashed lines). The plateaus in the conductance at G=(2n+1)×2e2/h correspond to plateaus in the valley polarization at P=1/(2n+1). On the lowest n=0 plateau, and for 0<μ0≲Δ, the polarization is more than 95%.

For negative μ0, the current makes a transition from the conduction band in the wide regions to the valence band in the narrow region. This interband transition has previously been studied in an unbounded system18,19, where it leads to selective transmission at normal incidence. In the QPC studied here, we find that the interband transition destroys the conductance quantization—except on the first plateau, which remains quite flat in the entire interval −3Δ/2<μ0<3Δ/2. The resonances at negative μ0 are due to quasi-bound states in the valence band20,21. The polarity of the valley filter is inverted for negative μ0, with some loss of quality (in particular for the smooth potential).

Because of the large Fermi wavelength at small μ0, the quality of the valley filter is quite robust against edge imperfections. To demonstrate this, we have randomly introduced a fraction, η, of vacancies among the sites at the edges of the constriction. The resulting degradation of the polarization (averaged over a few hundred random configurations of vacancies) is shown in Fig. 4 (inset of bottom panel). The polarization remains above 95% if a few per cent of the atoms at the edge are removed, and removing as many as 1/10 of the edge atoms still leaves a polarization of 85%.

The operation of the valley valve is demonstrated in Fig. 5. The current is blocked for −3Δ/2<μR<0 with μL on the first conductance plateau, so that the constriction contains two valley filters of opposite polarity in series. The switching behaviour of Fig. 5 is similar to that obtained by Wakabayashi and Aoki in a simulation of a zigzag graphene ribbon containing a potential barrier12. We anticipate that the experimental realization of this device will make it possible to exploit the valley degree of freedom, in addition to spin and charge degrees of freedom, as a carrier of information in carbon electronics.