Introduction

The ability to render an object invisible has been a goal since the days of mythology and the Ring of Gyges. It is only recently that invisibility became a plausible subject of inquiry thanks to theoretical advances in electromagnetism1,2,3,4,5,6. Such cloaks fulfilled the two basic elements of invisibility: anything hidden inside was isolated as if hidden by a perfect insulator (mean values still transmittable) and the perfect insulator had no scattering. This first requirement typically entailed singular, anisotropic materials, while the second required inhomogeneity. These extreme material requirements turned attention to reduced cloaks which merely approximate perfect cloaking7,8,9,10 or conditions where these constraints are relaxed11,12,13,14,15,16,17. This in turn led to the study the detectability of these cloaks18,19,20,21,22. Concurrently, cloaking was extended to other classes of electromagnetic phenomena23,24,25,26,27,28,29, wave equations30,31,32,33,34,35,36,37. and diffusion equations38,39,40,41,42,43,44,45,46,47,48,49,50,51 (refs 52, 53 provide a review of these last categories).

The diffusive cloaks found greatest success with the heat equation:

(where ρ is the density, cp the specific heat capacity, T temperature, and κ the thermal conductivity), so we shall confine our attention to thermal cloaks and then generalize our results to other diffusion effects. Because the steady-state temperature is independent of the volumetric heat capacity ρcp, cloaking has focused on engineering κ with ρcp constant. This has led to the widespread acceptance of three related classes of diffusive cloaks. First, there are strictly steady-state cloaks that ignored ρcp through the assumption that they would only be used in a steady state. While the utility of such a device is limited (truly steady-state conditions are unlikely to be maintained outside of specialized circumstances), they unquestionably work as expected. Their success, however, led to a second class of cloaks, which sought to extend these steady-state designs to time-varying and transient temperatures. For example Schittny et al.43 adopt a theoretical approach from ref. 42 (which assumed constant ρcp as an explicit approximation) while still claiming to achieve transient thermal cloaking. Indeed, they even found experimentally that their specific heat capacity varied between their materials but sought to correct for it to maintain a homogeneous ρcp. In addition, Ma et al.45, simplify their design by assuming that ρcp commutes with spatial derivates, i.e. that homogeneity of ρcp is obtained to good approximation. The third class of diffusive cloaks were chemical diffusion cloaks49,50, which are governed by ∂tn = ∇ · (Dn∇n) for chemical concentration n and diffusion constant Dn. There, researchers again assumed that ρcp was negligible based upon the performance of steady-state thermal cloaks, and therefore that steady-state thermal cloak designs could be extended to transient effects in chemical diffusion (even though this analogy maps ρcp to unity everywhere). Thus, although the steady-state cloak was developed for the limited frequency range of ω = 0, there now exists a broad class of cloaks (which we refer to collectively as steady-state cloaks due to their common origin) which assume that careful engineering of κ is sufficient to create a perfect cloak in any regime. Note that an alternative approach to thermal cloaking was also presented in ref. 54, which used scattering cancellation theory. The theoretical justification for this type of cloaking is thus outside of the analysis we develop here, although it is worth noting that they explicitly engineer ρcp and κ. However, as a complementary media method, it only cloaks specific objects (or at most a class of objects all having the same effective parameters), whereas transformation media cloaks work for arbitrary objects. In addition, the technique works by cancelling out finite terms in the multipole expansion of the cloaked object’s scattering cross section, and so remains detectable using higher-order terms in the expansion (e.g. the quadrupole term in their example).

In this paper, we show a homogeneous ρcp results in a detectable, transient signal. Under changing boundary conditions, a thermal cloak will flicker and become visible, although it will help to obscure anything hidden inside it. The implications of this imperfection can be seen by considering a faulty cloak with one observer hidden inside and another searching outside (see Fig. 1). The searcher can send out signals and detect the diffuse “scattering” that is reflected back. Furthermore, they can search for signals emanating from the cloak’s interior − thereby determining the material composition or temperature distribution hidden inside. Conversely, the observer hiding in the cloak can detect incoming signals to observe any searchers and eavesdrop on the outside. Moreover, by sending out their own signals and detecting them, they can confirm that the cloak is present and functioning.

Figure 1
figure 1

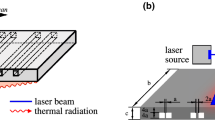
Simple model for detecting a cloak.

Cloaked region of radius a inside a cloak of radius b is protected from outside searchers. To detect the cloak, it is surrounded by two heat baths (red and blue rectangles) in a thermally isolated domain. Heat (red lines) flows through the cloak and emerges without distortion. However, for an imperfect cloak heat is also scattered. The scattered heat diffuses, but a thermometer placed near the cloak can detect it.

Full size image

Results

We begin by considering the analytic solution to eq. 1 for the cylindrical perfect cloak (PC) (assuming no z dependence). For a homogeneous medium (κ = κ0, ρcp = ρ0cp0), source-free medium the solution can be expressed as a linear combination of the fundamental solutions

where l the rotational symmetry eigenvalue, Rl is a modified Bessel function of the first or second kind (Il and Kl respectively), , and ω is a frequency >0 (the steady state of ω = 0 is discussed in the supplement). A PC of interior radius a and exterior radius b (Fig. 1) is constructed from

the solution becomes

where kC/k0 = b/(b − a). Whereas, for a steady-state cloak (SSC) κ is the same as eq. 3 but ρcp = (b/(b − a))ηρ0cp0 (η a mismatch parameter, η = 1 is eq. 3 evaluated at r = b), the lowest order perturbation is

where , and is given in the supplement (along with the full analytic solution). Crucially, λ determines the strength of the perturbation, meaning the effectiveness of an impedance matched SSC (η = 1) is proportional to the size of the cloaked region over the diffusion length. For reference, the various cloaks considered in this paper are summarized in Table 1.

Table 1 Equations for cloaks considered in this paper.
Full size table

Since the solution to eq. 5 is not a tabulated function, we use COMSOL multiphysics55 to solve eq. 1 directly for the SSC. Following the most common test of a cloak, we model the cloak in a rectangular region where one pair of ends are held at fixed temperature and the other pair admit no heat (Fig. 1). Given the linearity of eq. 1, one boundary is set to 0 (as is the initial T) and the other to 1 (ΔT ≡ 1). It is helpful to use the natural units of L (the separation of the heat sources) and the diffusion time τD = L2ρ0cp0/κ0 (all parameters values are in the supplement). Figure 2 is the result of these calculations. Each column is a snapshot at a different time. The first row is the homogeneous background that would be observed if there was no cloak, the second is the solution to SSC (with η = b/(b − a) to increase contrast), and the third is the difference . This deviation δT is what must be detected to reveal a cloak. Initially δT is small and mostly localized to where the cloak has been heated (see Fig. 2g). Later (Fig. 2h) δT grows and is clearly observed outside the cloak. Finally in the steady state (Fig. 2i) invisibility is restored, as expected for a SSC (δT ≠ 0 confined to within the cloak).

Figure 2
figure 2

Simulated temperature snapshots for mismatched SSC (η = b/(b − a)).

Columns correspond to 2.08τD/100, 2.08τD/10, and 2.08τD respectively. Rows correspond to the homogeneous case (no cloak), SSC, and T(SSC) − T(H). Black circles denote the location of the cloak (for reference in the homogeneous case), colored domains are isotherms, and grey lines are constant separation isotherms.

Full size image

To clarify the time-dependence of δT we select several points outside the cloak and compare δT for SSC with η = 1 (i.e. impedance matched, cloak has the same properties as a PC at r = b), η = b/(b − a) >1 (impedance mismatch but ), and the PC in Fig. 3. As we prove in the supplement, δT = 0 outside the cloak for the PC (i.e. that a perfect cloak is perfect), so the non-zero δT must be a numerical artifact of discretizing κ and thereby removing κθ → ∞ (removal of this singularity introduces scattering fields which scale to lowest order like 1/ln kCδ, where δ is the deviation at the inner boundary. This is further corroborated by the penetration of heat into the cloaked region). However, for both SSC models |δT(SSC)| > |δT(PC)| outside the cloak. This is the effect a homogeneous ρcp. Note that the position dependence of δT is approximately just a scaling factor so that the peaks all nearly coincide instead of being separated by a propagation time. Both this and the time dependence (linear growth for small t, exponential decay for large) are derived in the supplement, where we show that this separable space dependence implies that δT is dominated by a small number of Fourier-modes.

Figure 3
figure 3

Temperature deviation δT/ΔT for representative points outside the cloak as a function of time.

Black (a), blue (b), and red (c) curves correspond to the PC, impedance matched SSC, and impedance mismatched SSC. Line styles correspond to individual points, as shown in the inset.

Full size image

The results of Fig. 3a present a finite element, (i.e. discretized) approximation of the exact inhomogeneity relations that constitute a PC. They are therefore not just a numerical approximation of an analytic cloak but also a computational approximation of how a near ideal, experimentally realizable cloak might behave. The reduction in the cloak’s scattering that we gain by incorporating the inhomogeneity of ρcp is reflected drop in the temperature deviation peaks between Fig. 3a,b (a factor of 2 for the impedance matched SSC and a factor of 4 for the impedance mismatched SSC).

While the SSC model uses a realistic ρcp, it still contains an idealized κ. The exact inhomogeneity and anisotropy profiles of eq. 3 are not physically realizable. Thus, experimental verification of our predictions for the SSC is impeded by its use of an idealized κ. In real thermal cloaks, rings of discretized, constant κ are used in an approximation of this ideal inhomogeneity curve. Hence, for a direct comparison, we move from the SSC model that we have developed to the case of a bilayer cloak (BC)48 (simulations and further experimental data for the BC are in the supplement). The BC is particularly interesting to consider as it is a SSC that was derived directly from Laplace’s equation rather than a coordinate transformation (similar to54). In Fig. 4 we plot the normalized temperature deviation for the simulated BC and our experimental realization. This shows a good agreement, with a slight discrepancy near the boundaries of the system. This is due to a slight difference in the experimental temperature gradients applied to the BC and homogeneous cases.

Figure 4
figure 4

Comparison of simulations and experimental for the BC.

Columns correspond to 1.14τD/100, 1.14τD/10, and 1.14τD respectively. Rows correspond to δT for the simulation and experiment respectively.

Full size image

Finally, we turn to the question of detecting objects hidden inside a cloak. For the PC and the SSC at the boundary (κr = 0), so there should be no heat transferred and therefore no discernable signal (although, as in ref. 18, this is extremely sensitive to deviations of κr from 0 and as in refs 23, 24 and 37 even a PC will transmit the mean value of T at the boundary). However, taking the BC and changing the material hidden inside will effect the temperature distribution. An exterior temperature profile like those considered above must pass through the cloak twice (entering and exiting), so the cloak’s ability to suppress detection is stronger here than in the case of hiding the cloak. In particular, simulations of the BC with different materials inside differ by less than 0.1% (see Fig. 5). Assuming a thermometer of sensitivity of 0.2 K, a gradient of over 200 K would be necessary for the determination of the material hidden within the cloak (whereas merely detecting the presence of the BC requires a temperature difference of 3.64 K).

Figure 5
figure 5

Deviation of temperature profiles with changing cloaked object composition (T(cloak+object) − T(cloak))/ΔT for representative points outside the cloak as a function of time.

Color corresponds to different points (see inset for key).

Full size image

On the other hand, one could try to detect the temperature distribution hidden by the cloak, rather than the material. In this case, heat initially confined to the cloak would diffuse out and only pass through the cloak once. Simulations in this case show a detectable signal of 1.5% (see Fig. 6, computational details in the supplement), meaning that a thermometer of 0.2 K sensitivity could by used to detect a temperature difference of at least 13.3 K from the background. It is therefore likely that heat sources can be detected through the cloak, i.e. that it acts like an imperfect insulator. Comparing the efficacy of the BC as an insulator to that of a thermal insulator with properties equal to the insulating layer of the BC (essentially, removing one of the layers) indicates that the BC is no better at suppressing this diffusion of heat into the environment. This suggests that realizable cloaks (i.e. those without a perfectly insulating inner boundary) are no better than conventional insulators for maintaining a temperature difference. This is not entirely surprising if we think of the thermal cloak as a composite or inhomogeneous wall, but it reveals a way in which thermal cloaks deviate for our intuition of what cloaking means (indeed, suggestions that thermal cloaks can hide hot spots or the thermal signatures of objects are somewhat commonplace in the community54, is one such example). Moreover, even a PC23,24 with a perfectly insulating inner boundary would still not prevent the matching of the average temperature inside and outside of the cloak, so this set up should still eventually equilibrate. For the PC, though, this should take much longer and be a much smaller signal, so the lack of a perfect insulating layer aids experimental detectability of the internal temperature distribution.

Figure 6
figure 6

Temperature response of the BC used as an insulator.

Plot (a) shows the time dependence of a representative point outside the cloak while plot (b) shows the spatial dependence of a slice through the cloak (see inset) at various times (in seconds). The fluctuation near the inner boundary is a numerical artifact of the discontinuity in initial temperature.

Full size image

Discussion

We have shown that a SSC can be detected by its transient response. Because the distinction between a PC and a SSC is just ρcp, the ability to engineer the volumetric heat capacity is necessary to prevent the ω ≠ 0 response from revealing the cloak. However, the narrow range of ρcp in currently available materials makes it extremely difficult to design this inhomogeneity (indeed, even efforts to construct a “transient” thermal cloak have assumed constant ρcp43,45). This is particularly true for other classes of diffusion cloaks where the analog of ρcp is necessarily constant everywhere49,50. It remains an open question, however, if a diffusive cloak (thermal or otherwise) could be designed to make its time-dependent response undetectable in practice even if the response exists in principle.

Methods

Analytic solutions

Given the heat equation with homogeneous materials

in polar coordinates we take the Fourier transform of time and use a separable solution T(r, θ, t) = R(r)eilθeiωt giving

This is the differential equation for a modified Bessel function (Il(z) or Kl(z)) of for ω ≠ 056. The time-dependent solution is therefore

For the steady state of ω = 0 the solutions become the solution to Laplace’s equation

for l ≠ 0 and

for l = 0. The general solution is therefore .

For a perfect cloak

we can make the coordinate transformation

to reduce the solution in the primed coordinates to the homogeneous case.

For a steady-state cloak (κ as for the perfect cloak, ρcp = ρ0cp0(b/(b − a))η, i.e. evaluating ρcp at r = b when η = 1) no transformation will reproduce a homogeneous solution. Using and separation of variables we find

where . This can be solved by the method of Frobenius with recurrence relation

This relation is exact, but additional insight can be gained by expanding the solution by powers of Ka. For even terms in the series this is

which is the same as series expansion for Il and Kl respectively. On the other hand, for odd terms it becomes

Because is completely determined by the odd terms are therefore a function of the modified Bessel functions. Ergo, we term these components . A similar derivation can be carried out for a spherical cloak where l becomes half-integer instead of integer.

Computational and Experimental Methods

For the PC and SSC we use COMSOL multiphysics to model a rectangular domain of dimensions L = 70 mm by L⊥ = 50 mm centered around a cloak of dimension a = 13 mm, b = 20 mm. The background medium is κ0 = 71.4 W/m · K, ρ0 = 2100 kg/m3, and cp0 = 1000 J/kg · K. This gives a diffusivity of D = κ0/ρ0cp0 = 3.4 · 10−5 m2/s and diffusion timescale τD = L2/D = 144.12 s. The initial temperature was 293.15 K with thermal baths at 300 K, and T0 = 293.15 K giving a ΔT of 6.85 K. After confirming that the simulations were invariant under a change of scale we use the natural units of x/L, y/L, t/τD, (T − T0)/ΔT.

For the BC, we follow48 and model it as rectangular domain of dimensions L = 45 mm by L⊥ = 45 mm centered around a cloak with hidden region of size a = 6 mm, first layer of r2 = 9.5 mm, and second layer of b = 12 mm. The background medium is κ0 = 2.3 W/m · K, ρ0 = 2000 kg/m3, and cp0 = 1500 J/kg · K, the outer layer’s medium is κ1 = 9.8 W/m · K, ρ1 = 8440 kg/m3, and cp1 = 400 J/kg · K, the inner layer’s medium is κ2 = 0.03 W/m · K, ρ2 = 50 kg/m3, and cp2 = 1300 J/kg · K, and the interior medium is κ3 = 205 W/m · K, ρ3 = 2700 kg/m3, and cp3 = 900 J/kg · K. This gives a diffusivity of D0 = κ0/ρ0cp0 = 7.67 · 10−7 m2/s and diffusion timescale  s. The initial temperature was 273.15 K with thermal baths at 333.15 K, and T0 = 273.15 K giving a ΔT of 60 K. The experimental tests of the BC were performed with the same setup, following the procedure outlined in ref. 48.

As for the ability of a cloak to insulate a cloaked object and thus disguise the temperature profile, it is helpful to use different boundary and initial conditions. Instead of applying a thermal gradient across the boundaries, the cloaked region is initially set to 60 K above the background (and cloak) at 273.15 K (These values are then rescaled to 1 and 0). Because the thermal baths are at fixed temperature and perfectly absorb heat flux, energy is not conserved in this simulation and so the steady state should have all the heat removed from the cloak.

Additional Information

How to cite this article: Sklan, S. R. et al. Detecting Thermal Cloaks via Transient Effects. Sci. Rep. 6, 32915; doi: 10.1038/srep32915 (2016).