Introduction

Silicon has been a material of choice for direct solar to electrical power conversion due to its abundance, reliability and mature manufacturing technology. However, the indirect electronic band gap of silicon hinders absorption of sunlight at long wavelengths. Gallium Arsenide (GaAs), on the other hand, has a direct electronic band gap and can provide greater power conversion efficiency. Since it is more scarce and costly than silicon, it is vital to optimize solar absorption using the least amount of GaAs. This can be achieved by sculpting GaAs into sub-wavelength periodic nanostructures known as photonic crystals. In this work, we show using coupled numerical modeling, that nearly 90% of sunlight, in the available wavelength range, can be trapped and absorbed in ultra-thin films of nanostructured GaAs consisting of only 200 nm of GaAs. This enables photonic crystal solar cells, utilizing less than one-tenth the volume of GaAs than state-of-the-art single-junction GaAs solar cells, to achieve record high power conversion efficiencies, beyond 30%.

Resonant light scattering and wave-interference effects provide powerful light-trapping mechanisms1,2 in nanostructured thin-film solar cells. It has been shown3,4 that these wave effects can be exploited in certain photonic crystal5,6,7,8,9,10 designs to achieve very high solar absorption using one or two orders of magnitude less active material than conventional solar cells. These photonic crystal architectures have an effective graded refractive index near the top surface to minimize reflection and scatter incoming sun light into slow-light modes that propagate nearly parallel to the interface between the active region and air above5. The resulting long dwell time of sunlight within a thin-film enables stronger light absorption than anticipated in the so – called Lambertian limit7. Enhanced solar absorption occurs in a wavelength range in which the electromagnetic density-of-states is much higher in the photonic crystal than in a homogeneous medium. These effects cannot be described using a ray optics picture and require detailed numerical solution of Maxwell’s wave equation within the solar cell. In the case of relatively thick (~100 microns) silicon solar cells, a detailed balance method11 based on Lambertian ray trapping has been used to estimate the limiting power conversion efficiency. However, this approach neglects wave interference effects that can provide stronger light-trapping and solar absorption using considerably thinner photonic crystal solar cells7,12.

The maximum Shockley-Queisser13 solar power conversion efficiency for a single junction GaAs solar cell is around 33.5% using an AM 1.5 solar spectrum. Recently, the efficiency of ~3 micron thick single-junction GaAs solar cells has improved considerably, reaching almost 29%14,15,16. By minimizing surface non-radiative recombination and maximizing photon trapping8 and recycling effects17,18,19,20,21,22,23 further efficiency increase toward the Shockley-Queisser limit remains possible.

An order of magnitude reduction in the volume of GaAs required to achieve such high efficiency is an equally important target. A two-fold strategy is essential to realize these goals. Firstly, optimized light management requires simultaneous antireflection and light-trapping5,6,7,8. This enables higher photon collection efficiency and correspondingly higher photo-current. Light-trapping also facilitates re-absorption of photons generated by electron-hole recombination. This effect, known as photon recycling19,20,21, leads to further charge carrier generation and an increase in open-circuit voltage. Under suitable circumstances, as we show below, this provides up to 2% additive increase in overall power conversion efficiency using only 200 nanometers equivalent bulk thickness of GaAs (similar additive increase was found using active layer thicknesses of more than a micron22,23).

Secondly, optimized electronic management involves minimization of Schottky-Read-Hall24 and other non – radiative recombination. Non-radiative carrier recombination is a particularly critical issue in the case of large surface and contact areas. To prevent large losses, surface passivation25 is required. Unpassivated surfaces, defective with dangling bonds, induce rapid non-radiative recombination. This is a major factor in the low open-circuit voltages observed in some fabricated solar cells26. Recent advances in surface passivation facilitate the deployment of nanostructured solar cells with a large surface area. For example, surface recombination velocities27 as low as 10 cm/s have been achieved on black silicon, using an aluminum oxide coating. The concentration of defects is usually maximal at metallic contacts. Metallic back-reflectors serving as a back contact generally need to be offset from the active region of the solar cell to reduce losses. Building electronic barriers also helps to deflect minority carriers away from the points where electrical contacts touch the active region. Such heavily doped regions function through what is called a back-surface-field24. Without proper minority carrier deflectors, the recombination loss at contacts can be significant. Substantial increase in solar cell performance is achieved by addressing these photonic and electronic management issues.

In this paper, we present the results of combined numerical solution of Maxwell’s equations coupled to semiconductor drift diffusion equations in ultra-thin-film gallium arsenide photonic crystal solar cells. With only 200 nanometers equivalent bulk thickness of GaAs, it is possible to achieve solar power conversion efficiencies near 30%. We show (Supplementary Information) that this choice of thickness provides the optimum balance between high power conversion efficiency and the volume of GaAs required. We also highlight the interplay between carrier radiative recombination leading to “photon recycling” and surface non-radiative recombination. In particular, a well-passivated surface can lead to nearly 2% additive increase in power conversion efficiency through the trapping and recycling of internally emitted light.

We present full 3D numerical simulations of photo-current, voltage and power conversion efficiencies in thin-film photonic crystal solar cells based on slanted conical nano-pores in GaAs8. Preliminary slanted-conical-pore structures have been fabricated on small scales using ion beam etching at S. Juodkazis group (private communication) and closely related vertical-pore structures have been fabricated on large scales using dry-etching methods at S.Y. Lin group (private communication). Light-trapping in a slanted conical nano-pore architecture enables 90% solar absorption in the wavelength range from 300–860 nm using only 200 nm equivalent bulk thickness of GaAs8. We calculate the dependence of power conversion efficiency on the degree of surface passivation. Despite the significant surface profile of our photonic crystal, efficiency is high provided the surface recombination velocity remains below 104 cm/s. We also show the increased role of photon recycling when the surface recombination velocity is reduced by improved passivation.

While untreated GaAs can have an extremely high surface recombination velocity (106 cm/s), passivation with a wide band gap semiconductor such as AlxGa1−xAs provides long-term surface stability. For epitaxially- passivated, planar GaAs surfaces, recombination velocities below 60 cm/s have been reported28. Nonplanar GaAs surfaces are harder to passivate, because of a higher density of electronic surface states. For GaAs nanowires29, a surface recombination velocity of 1700 cm/s was reported, indicating that the surface states were only partially passivated or that stacking faults and bulk impurities were further contributing to carrier non-radiative recombination. Advances in epitaxial growth of III-V semiconductors through complex 3-D structures with non-planar surfaces are nevertheless forthcoming30. In the present study, we demonstrate a strong synergy between surface passivation and photon recycling effects, highlighting the efficacy of surface recombination velocities as low as 10 cm/s. Although this lowest value has not yet been experimentally achieved for GaAs, we anticipate that our theoretical roadmap may stimulate research in this important direction.

To calculate an efficiency of our photonic crystal solar cells, we combine optical and electrical finite-difference time-domain (FDTD) simulations31. The solution of Maxwell’s equations provides the absorption profile inside the structure that defines the charge carrier generation profile. This profile is used as the input for the semiconductor drift-diffusion equations to calculate solar cell efficiency, taking into account radiative recombination throughout the bulk and non-radiative recombination at surfaces. When all internally generated light from radiative recombination is assumed to escape the solar cell into free space, we obtain a power conversion efficiency of 28.3% in our 200 nm GaAs photonic crystal with surface recombination velocity of 103 cm/s. In the opposite limit, when all light from radiative recombination is assumed to be recycled, this efficiency improves to 29%. Most strikingly, if the surface recombination velocity is reduced to 10 cm/s, the role of photon recycling is significantly elevated. In this case, complete recycling of re-radiated light leads to a theoretical power conversion efficiency of 30.6%.

Numerical model

We calculate solar absorption inside our solar cell using the standard FDTD algorithm32,33, in which a plane wave impulse having Berenger’s form32 with a broad spectrum impinges onto the structure. Electromagnetic fields are recorded, transformed to the frequency domain and normalized to the incident spectrum. We define (in CGS units) a frequency – and spatially – dependent absorption coefficient at each point r inside the structure:

here, ω is the angular frequency, is the electric field amplitude calculated at each point of a computational grid located within GaAs, ε(ω) is the frequency-dependent and complex dielectric permittivity of GaAs8, c is speed of light, E0 and H0 are electric and magnetic vectors of the incident plane wave, the superscript * indicates the complex conjugate, is a normal unit vector pointing from air to the surface of the absorbing medium and integration is over a unit cell area of the surface. If is integrated over all in the unit cell volume (defined by the unit cell surface area times the depth of the absorbing medium), it yields the frequency - dependent absorption coefficient: . Assuming that each absorbed photon leads to the generation of a single e-h pair, we calculate an initial charge carrier generation rate (in units of number per unit time, per unit volume) produced by the incident sunlight. This generation rate is obtained by the integration of the calculated absorption with the incident Air Mass Global Spectrum intensity I(ω) over the wavelength range 300–865 nm:

We use this spatially – dependent generation rate as an input parameter to the semiconductor drift – diffusion equations:

Here, R and G are the total recombination and generation rates, p and n are electron and hole densities, NA and ND are acceptor and donor doping concentrations, ψ is the electrostatic potential, q is an elementary charge, ɛ is the dielectric function of GaAs, Jn and Jp are electron and hole currents and μn and μp are electron and hole mobilities. In principle, the total generation rate G = Gsolar + Grecycle, where Grecycle is the generation rate from re-absorbed photons produced internally by electron-hole recombination. The electron-hole recombination rate here is a sum of contributions from radiative and non-radiative processes: R = Rrad + RSRH, where RSRH is the Shockley-Read-Hall non-radiative recombination from a single-trap level at the electronic midgap24 of GaAs. In our ultra-thin film photonic crystal, we can safely neglect bulk non-radiative recombination (RSRH = 0), since the diffusion length of crystalline GaAs is more than one hundred times the maximum charge carrier transport distance in our structure. The non-radiative recombination consists entirely of surface recombination, which is treated as boundary condition. This is prominent at GaAs surfaces, especially near electrical contacts. Non-radiative recombination is described by the boundary conditions: , , where is the unit normal vector to the surface, Vsr is a surface recombination velocity and n1, p1 are equilibrium electron and hole concentrations defined below. The boundary condition for the electrostatic potential at the metal-semiconductor interface is: ψ = Vappl + ψbi, where Vappl is the voltage applied across the contacts (we put V = 0 at upper contact and then Vappl at bottom contact), ψbi is the built-in potential (potential across the depletion region in thermal equilibrium that inhibits further carrier diffusion across the junction) given by: , where T is temperature and ni is the intrinsic carrier concentration of GaAs (ni = 1.9·106 cm−3 at T = 300 K). Equilibrium concentrations are given by24:

These concentrations are simple consequences of the charge neutrality condition: ND + p = NA + n and the law of mass action: .

Radiative recombination of electrons and holes (in units of number per unit time per unit volume) is given by24:

here, B = 1.3·10−10 (with units of volume per time) is the recombination coefficient24 of GaAs.

For the semiconductor-insulator interfaces we use following Neumann boundary conditions: , which implies that there are no surface charges (the normal component of the electric field is zero) and no current flow through the surface.

We discretize (3) using the Scharfetter–Gummel scheme31. This leads to a system of nonlinear equations that can be efficiently solved by Newton’s technique31. In general, photon recycling requires repetitive iterative solution of the set of equation (3) in steady state. While the solar generation rate Gsolar is known, the internal generation rate Grecycle is an outcome of calculation. In principle, Grecycle is obtained by placing FDTD sources that emit with an intensity governed by the rate Rrad in equation (5) and then calculating absorption from these sources, using Maxwell’s equations throughout the active region. This process must be repeated until the input generation rate and the output generation rate converge throughout the sample volume. This iterative process will be described in detail elsewhere. For simple estimation purposes, we consider two limiting cases. In the first case, we assume that all light from radiative recombination simply escapes the solar cell without re-absorption. In the second case, we assume that all light from radiative recombination is trapped by the photonic crystal architecture and re-absorbed very close to the emitter. The latter is implemented by setting Grecycle = Rrad throughout the sample. As we discuss below, our light-trapping photonic crystal architecture enables re-absorption of nearly 70% of the re-emitted light.

Solar Cell Architecture

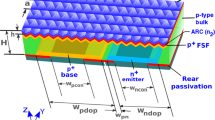
Our detailed photonic crystal GaAs solar cell design is depicted in Fig. 1. Here, we show one unit cell of the square lattice of slanted conical nano-pores in GaAs with the equivalent bulk thickness of 200 nm, filled (encapsulated) with glass (n = 1.45) and placed on silver back-reflector. The height of GaAs between the contacts is 290 nm (for a 200 nm equivalent bulk thickness of GaAs). As in the case of silicon solar cells7, slanted conical pores provide more effective solar absorption than their non-slanted counterparts. The optimal geometrical parameters, for maximal absorption are8: a = 550 nm, r = 300 nm, where a is the lattice constant and r is the cone base (top) radius. To calculate the absorption (1) and the generation profile (2), we perform FDTD simulations following the algorithm described above. The maximum achievable photo-current density is given by: , where a is the lattice constant and integration is done over the entire volume of GaAs in a single unit cell. In the case that all light from radiative recombination is assumed to escape the structure, we achieve a MAPD of 27.6 mA/cm2 in the 300–865 nm wavelength range, which corresponds to 88% of absorption of incoming solar power in the specified range. In this calculation, the surface of each pore is passivated with a thin layer of Al0.4Ga0.6As of 10 nm width and the cones are filled with glass (n = 1.45). We also use a 50 nm thick buffer layer of Al0.4Ga0.6As with higher doping (N+A = N+D = 5·1018 cm−3) between the silver substrate and GaAs to deflect the minority carriers and reduce contact recombination. A similar 10 nm thick buffer layer with higher doping is used for the same purpose on the top of the cell covered with transparent (n = 1.9) indium tin oxide (ITO) contact with the thickness of 10 nm (see Fig. 1). A p-n junction is formed in the middle of the cell (magenta plane), with equal doping on either side: NA = ND = 1018 cm−3. We also vary the position of p-n junction and find that it affects the final power conversion efficiency only slightly, since the diffusion length in crystalline GaAs is more than one hundred times longer than the typical carrier transport distance in our structure. Most carriers reach the contacts without non-radiative recombination in the volume. Solar power conversion efficiency in our structure is limited by surface and contact recombination.

Figure 1
figure 1

Unit cell of square lattice photonic crystal solar cell architecture based on slanted conical pores in bulk GaAs filled with glass.

The photonic crystal slab rests on a silver substrate of 100 nm width. The depth of GaAs cones is 290 nm (for a 200 nm equivalent bulk thickness of GaAs). The radius of cones and the lattice constant are r = 300 nm and a = 550 nm respectively. The ITO 10 nm thick contact (refractive index n = 1.9) rests on 10 nm thick Al0.4Ga0.6As with higher doping (N+D = 5·1018 cm−3). The width of Al0.4Ga0.6As buffer layer with higher doping (N+A = 5·1018 cm−3) placed in between GaAs and the silver substrate is 50 nm. The surface of the pore is coated with a layer of neutral Al0.4Ga0.6As of 10 nm width and the rest of the pore is filled with glass (n = 1.45). The p-n junction is formed in the middle of the cell (magenta plane), with equal doping above and below: NA = ND = 1018 cm−3.

Full size image

Power Conversion Efficiency

The results of solving the coupled Maxwell and drift-diffusion equations for the structure are shown in Fig. 2. The I–V characteristics for surface non-radiative recombination velocities of Vsr = 103cm/s and Vsr = 10 cm/s are shown in Fig. 2. In both cases the insulating surface and contact recombination velocities are set equal. For Vsr = 103cm/s the power conversion efficiency is 28.3%, whereas for Vsr = 10 cm/s, we achieve 28.6% efficiency. In the above cases, all light generated by radiative recombination was assumed to escape the structure. In the opposite limit that all radiative recombination is recycled (Rrad = Grecycle), 0.7% additive efficiency increase is achieved in the first case (it reaches 29%), whereas 2% is achieved in the second case (30.6% is achieved).

Figure 2
figure 2

The I–V curves of the solar cell shown in Fig. 2.

Solid (no photon recycling) and dash (perfect photon recycling) lines represent the same case with equal surface recombination. Blue curves correspond to the case when surface recombination velocity Vsr = 10 cm/s and red curves when surface non-radiative recombination velocity Vsr = 103 cm/s. Power conversion efficiency is denoted as ‘PCE’.

Full size image

The enhanced role of photon recycling with the suppression of non-radiative decay provides significant increase in Voc. The open circuit voltage behavior in Fig. 2, can be understood from the simple 1D diode equation34 for net current flow: I = Isc − Isat[exp(qV/kT)−1]. Here, Isc is the short-circuit photocurrent (when V = 0), V is the voltage across the contacts, k is Boltzman’s constant, the temperature T = 300 K and Isat is a saturation current, determined by carrier recombination. The open circuit voltage Voc is obtained by setting I = 0:

The saturation current34 in our case, arises from surface nonradiative recombination and bulk radiative recombination: Isat = Isurf + Irad. A rough estimate of the surface recombination current, based on dimensional considerations is given by: , where Vsr is the surface recombination velocity and S = 0.565 μm2 is the combined area of the GaAs – AlGaAs and GaAs – contact surfaces in each unit cell. This gives Isurf  = 6.5·10−19mA for Vsr = 103cm/s. Likewise, the radiative recombination current, in the absence of photon recycling is estimated by integration of the radiative recombination coefficient Rrad (5) over the unit cell volume: . In the case of perfect photon recycling, we set Isat = Isurf. When Vsr = 10 cm/s, equation (6) yields Voc1 = 1.18 V with no photon recycling and Voc2 = 1.29 V with perfect photon recycling. On the other hand, when Vsr = 103 cm/s, equation (6) yields Voc3 = 1.15 V with no photon recycling and Voc4 = 1.17 V for perfect photon recycling.

The crude model above overestimates the actual open circuit voltage in our system, but provides a simple physical interpretation of the enhanced role of photon recycling with low surface recombination velocity. In our numerical simulations (see Fig. 2), we find the corresponding voltages: Voc1 = 1.14 V, Voc2 = 1.23 V for Vsr = 10 cm/s and Voc3 = 1.12 V, Voc4 = 1.15 V for Vsr = 103cm/s. Clearly, the ratios Voc1/Voc2 = 0.91 and Voc3/Voc4 = 0.98 estimated from (6) are close to those (Voc1/Voc2 = 0.92 and Voc3/Voc4 = 0.97) of our numerical simulation results in Fig. 2. This underscores the value of improved surface passivation in photonic crystal solar cells to enable the strong synergy between light-trapping and photon recycling.

Setting Grecycle = Rrad is equivalent to 100% collection of photons emitted by radiative recombination. In reality this effect is slightly less pronounced, since some of the regenerated photons escape the solar cell. We estimate the amount of re-cycled light by FDTD simulation of spatially distributed point dipoles with oscillation amplitude determined by the semiconductor drift-diffusion equations. The re-radiation strength of each dipole is chosen such that the number of photons per unit frequency interval near ω, per unit volume near is given by:

. The distribution of dipole oscillation frequencies is governed by the thermal distribution of electrons and holes at the operating voltage of the solar cell35. For each radiating dipole at position within the active region, we calculate the escaping Poynting vector flux, , where S is a surface surrounding the entire solar cell and is the Poynting vector field of the i-th dipole. The total emitted radiation from the ith dipole is: , where Sd is a surface surrounding the dipole. Sd consists of a small box with faces 10 nm from the ith dipole within the active region. The radiation from each dipole depends strongly on the local EM density of states. This is automatically recaptured by our FDTD algorithm. The average amount of radiation re-absorbed by the solar cell is: , where N is the number of dipoles. As a representative simulation, we uniformly spread N = 121 dipoles throughout the active region of a vertical plane bisecting the slanted cone. For radiative recombination in this representative plane, we find that 70% of the emitted light is recycled. A more detailed description of photon recycling using a self-consistent iterative solution of the coupled Maxwell and drift-diffusion equations will be presented elsewhere.

In Fig. 3 we present the dependence of power conversion efficiency on surface non-radiative recombination velocity. The blue curve illustrates the drop in efficiency with Vsr in the absence of photon recycling when both surface and contact recombination are set equal. This suggests that Vsr = 104 cm/s is the tipping point, below which solar cell performance is very good but above which performance drops dramatically24,26. We attain 28.3% power conversion (no recycling) for Vsr = 103 cm/s with Voc = 1.12 V and JSC = 27.6 mA/cm2. These values are already very close to the current world record for single-junction GaAs solar cell efficiency without solar concentration. On the other hand, our photonic crystal solar cell uses 15 times less volume of GaAs compared to the record-setting solar cell.

Figure 3
figure 3

Solar cell efficiency as a function of surface recombination velocities.

Blue curve depicts the efficiency when surface and contact recombination velocities are equal and there is no photon recycling. Green curve represents efficiency vs. contact recombination velocity with surface recombination velocity fixed at 10 cm/s. The red curve depicts efficiency with perfect photon recycling (Grecycle = Rrad) with equal surface and contact recombination rates.

Full size image

The red curve in Fig. 3 depicts efficiency improvement with the perfect photon recycling. As before we set Grecycle = Rrad. The most pronounced effect is achieved (see also Fig. 2), when surface and contact recombination velocities are minimal (Vsr = 10 cm/s). The additive contribution to solar cell performance is 2% for Vsr = 10 cm/s, 0.7% for Vsr = 103 cm/s and becomes negligible for Vsr > 105 cm/s.

Finally, we isolate the impact of contact recombination on solar cell performance (dotted green curve). In this simulation, the insulating surface recombination velocity is fixed at Visr = 10 cm/s, while the contact recombination velocity Vcsr is varied. It is seen that below 104 cm/s, blue and green curves almost coincide. The difference becomes more pronounced for Vcsr > 105 cm/s. In this case, contact recombination losses alone degrades solar cell performance irrespective of the quality of passivation of all insulating surfaces.

Conclusion

We have identified opportunities for reducing the volume of GaAs by more than an order of magnitude relative to conventional solar cells while increasing power conversion efficiencies beyond the current world record. The critical trade-off in our photonic crystal architectures is between wave-interference-based light-trapping and non-radiative carrier recombination at surfaces. The large surface area of nanostructured photonic crystals makes them prone to non-radiative losses while at the same time providing strong scattering and trapping of sunlight. As a consequence of this trade-off, we identify roughly 200 nm equivalent bulk thickness of GaAs as an ideal photonic crystal solar cell. For lower volumes of GaAs, solar absorption decreases rapidly. For larger volumes of GaAs, only slight increase in power conversion efficiency is possible. This estimate is based on a surface non-radiative recombination velocity of 103 cm/s. Recent advances27 in surface passivation of complex silicon surfaces have provided recombination velocities on the order of 10 cm/s. If a similar degree of passivation is realized for GaAs, a 2% additive increase in power conversion efficiency is possible from the strong luminescence properties of GaAs. Our photonic crystal architecture not only traps incoming sunlight but it also facilitates trapping and re-absorption of light produced by radiative recombination of charge carriers. The effect of photon recycling is most prominent when non-radiative recombination is strongly suppressed, enabling our 200 nm GaAs solar cell to reach power conversion efficiency as high as 30.6%. This theoretical prediction is substantially above the world – record at one-sun incident flux, for a single-junction GaAs solar cell of any thickness.

Additional Information

How to cite this article: Eyderman, S. and John, S. Light-trapping and recycling for extraordinary power conversion in ultra-thin gallium-arsenide solar cells. Sci. Rep. 6, 28303; doi: 10.1038/srep28303 (2016).