Introduction

Recent progress in the development of x-ray focusing optics has pushed the frontier of hard x-ray nanofocusing into a regime well below 30 nm, providing high focusing efficiencies even for hard x-rays with photon energy above 10 keV1,2. Technological advances in optics fabrication offer a unique opportunity for x-ray imaging with high spatial resolution and allow to benefit from x-ray's excellent penetration power, high sensitivity to structural, elemental and chemical properties of a specimen and insensitivity to external electromagnetic fields. We have pursued the development of MLL optics as a route towards nanometer scale hard x-ray imaging and have built a scanning microscope using MLL optics3,4,5,6,7,8. Fluorescence imaging with a 2D resolution of 25 × 27 nm2 has been demonstrated at the energy of 12 keV and comparable performance was achieved at 19.5 keV8. This emerging new capability enables studies of nanostructures with unprecedented spatial resolution by fully exploiting various contrast mechanisms for quantitative analysis.

In addition to the conventional absorption and fluorescence contrasts, it has been demonstrated that the differential phase contrast can be obtained in the scanning mode by analyzing the transmitted diffraction pattern in the far-field9,10,11,12,13. Unlike other phase imaging techniques14,15,16,17,18,19, DPC neither requires a fully coherent beam nor suffers from a phase wrapping problem, because it measures the phase gradient. On the other hand, its resolution is limited to the probe size. Different methods utilizing Wiener filter10, differential intensity9 and moment analysis13 have been developed to retrieve the phase quantitatively. Other methods based on wedged absorber12 or pinhole11 are mostly variants of the differential intensity method. The Wiener filter method assumes a weak phase object and is not valid for a thick specimen, which is often a case for real samples. The differential intensity method assumes a uniform exit wave field or pupil function for the focusing optic. The moment analysis method measures the convolution of the phase gradient with the x-ray probe and artifacts can be introduced if the probe function cannot be described by a simple model. To enable quantitative phase imaging capability for MLL optics and to take advantage of its high spatial resolution, we propose a highly robust and generic DPC algorithm that seeks a best-fit solution to the measured far-field intensity. Our method neither requires prior knowledge of a specimen nor the probe, allowing a maximum generality.

Results

Concept description

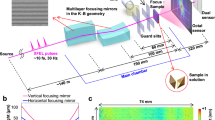
A schematic drawing in Fig. 1 depicts the experiment setup and a detailed description is given in the Methods section. At the far-field detector plane, the Fraunhoffer diffraction condition is satisfied and the wave field arriving at the detector is related to that at the focal plane by a Fourier transform. We assume that the complex wave field at the focal plane is simply a product of the probe function, and a specimen transmission function, , where is the translation vector in a 2D scan. For a nanoprobe, the value of P is appreciable only within a small area. We can therefore expand the amplitude, a, and phase φ, of the transmission function into a Taylor series up to the first order about r,

Due to the property of Fourier transform, the resulting diffraction pattern in the far-field will be displaced and attenuated as compared with the case when the specimen is not present, corresponding to . The displacement of the diffraction pattern is proportional to the local phase gradient vector and the attenuation is determined by . The absorption gradient contribution is very small and can be neglected9. For equation (1) to be valid, the beam size must be small as compared to the characteristic length of the local phase variation; locally the specimen can be approximated to a prism with a linear phase gradient. Once the phase gradient vector is found the phase can be reconstructed by integration.

Figure 1
figure 1

Schematic drawing of the experimental setup.

An incident plane wave is focused to a spot by two crossed MLLs. The fluorescence signal and the far-field diffraction pattern are recorded simultaneously as the specimen is raster-scanned.

Full size image

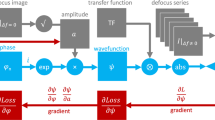
As mentioned earlier, the significant dynamical diffraction effect of MLL optics introduces a very nonuniform far-field diffraction pattern and a probe profile that cannot be approximated well to a delta function (see Figures S1 and S2 in supplementary materials). As a result a correct quantification of the phase gradient with existing methods becomes very difficult. To tackle this difficulty, we employ a non-linear fitting algorithm to seek a solution of the type shown in equation (1) that will give a least-square error to the measured far-field data. If the far-field wave field from a nanoprobe passing through a specimen only experiences attenuation and a shift due to the phase gradient, according to the Parseval's equation, a least-square fit of these two parameters can be found equivalently after an inverse Fourier transform of the diffraction pattern,

where is the residue error, is the reference far-field diffraction intensity distribution in the absence of specimen and is inverse Fourier transform operator. The Fourier-shift fitting process not only ensures a minimum error caused by noises but also removes contributions from high order phase terms by allowing a residue error. Because fitting is done in Fourier space, a sub-pixel intensity variation can be measured accurately, leading to high detection sensitivity. In the described method, only the shift of the diffraction pattern (not the pattern itself) is of interest. Thus a coherent beam is not required. A detailed derivation of the algorithm can be found in the Methods section.

Phase imaging of a SOFC anode

To verify the validity and demonstrate the advantages of our method, we investigated a SOFC anode sample, composed of Nickel (Ni) and Yttria-stablized Zirconia (YSZ) cermet20. The scanning electron microscope (SEM) image of the sample is shown in Fig. 2a. Figs. 2b–2d show the horizontal phase-gradient maps of the specimen obtained by the differential intensity, the moment analysis and Fourier-shift fitting algorithms, respectively. As it is evident from the comparison, the former two suffer from strong artifacts, resulting in false phase gradient distribution in the center and blurred contrast over the entire specimen. The poor image quality prevents using them for a quantitative phase reconstruction. In contrast, the Fourier-shift fitting algorithm gives superior contrast with no obvious artifacts.

Figure 2
figure 2

(a) SEM image of the SOFC specimen adhered on a Si3Ni4 window with Pt welding. (b–d) are horizontal phase-gradient images obtained by differential intensity, moment analysis and Fourier-shift fitting algorithms, respectively. Artifacts and blurring effects can be seen in (b) and (c), as compared to (d).

Full size image

Fig. 3a–3b show Ni and Pt21 fluorescence images and Fig. 3c–3d x-ray transmission image and reconstructed phase image of the specimen. The fluorescence signal from the YSZ electrolyte suffered significant absorption and was too weak to be used for quantitative analysis22. The absorption and phase measure the integral change of the imaginary and real components of the refractive index along the beam direction through the specimen, therefore allowing the detection of the buried interfacial structures. Both of them reveal the isolated and connected pores under the surface in the Ni anode and YSZ electrolyte, which cannot be seen in the SEM image. The phase image displays even higher levels of structural details due to a higher phase contrast, capturing all the structural features both in the Ni anode and the YSZ electrolyte. For example, a crack on the top of the sample, indicated by an arrow in Fig. 3d, can be clearly seen in the phase image but is barely detectable in both the absorption and the fluorescence images.

Figure 3
figure 3

Ni Kα fluorescence (a), Pt Lα fluorescence (b), x-ray transmission (c) and reconstructed phase (d) images (units in radian) of the SOFC sample shown in Fig. 2a. The arrow in (d) points to a crack, which is barely seen in (a), (b) and (c). A zoom-in image of the rectangle area in (d) with a high resolution can be found in the supplementary material.

Full size image

Composition imaging via absorption and phase measurements

Owing to the robustness and high detection sensitivity of our method, we were able to determine the refractive index decrement, δ and the absorption index, β, of the specimen with sufficiently high accuracy and consequently, able to exploit β/δ values as the imaging contrast. At fixed photon energy, this ratio provides unique fingerprint of the constituent elements, largely independent of the specimen thickness or the porosity. The composition image in Fig 4a, constructed from β/δ values, exhibits excellent correlation with the Ni and Pt fluorescence map. For a quantitative analysis, we plot the β/δ value along the yellow horizontal line in Fig. 4b. Comparison with the values for bulk Ni and YSZ indicates that the region from x ≈ −1.5 through x ≈ 3 is occupied by Ni and YSZ phases and the region afterward predominantly by YSZ phase. To verify the consistency of the data, we introduce a constant scaling factor to correlate the thickness of Ni phase to its fluorescence intensity. Provided that the total thickness of the specimen is known, the composition can be calculated as well from the Ni fluorescence data. The constant scaling factor is determined by minimizing the error between composition values obtained from absorption and phase (shown as circles in Fig. 4b) and fluorescence (shown as black line in Fig. 4b). If all measured data contained no error, the two curves should coincide. The observed discrepancy is ascribed to the noisy absorption data, in particular in weak absorption region and an oversimplified model for fluorescence. Nevertheless, the overall agreement is better than 30%, which is very reasonable. This allows us to estimate the fraction of YSZ within the specimen in confidence (blue curve in Fig. 4b).

Figure 4
figure 4

(a) is the composition map displays the variation of the β/δ ratio.The bright region correlates well to the Pt- and Ni-rich areas. The darker region in the bottom-right quadrant is mostly occupied by YSZ. A line plot across the yellow line is shown in (b) (black circle). The two red dashed lines correspond to the β/δ ratio for pure Ni (upper) and YSZ (lower) phases. The black solid curve is the β/δ ratio calculated from the Ni fluorescence data (see text for explanation). The two curves agree reasonably well, showing the consistency of the data. The blue line in (b) is the YSZ mole fraction extracted from the measurement.

Full size image

Discussion

Linking the micro/nano-structure evolution with SOFC's performance is a critical, yet unsolved, problem due to the lack of quantitative characterization tools with sufficient elemental, structural and chemical sensitivity at the nanoscale23,24. In particular for the detection of YSZ electrolyte, quantitative analysis using x-ray fluorescence mapping remains challenging. The robust yet generic DPC analysis algorithm based on a Fourier-shift fitting process developed in this letter enable us to use MLL opitcs to perform parallel measurements of fluorescence, absorption and phase with spatial resolution better than 50 nm on a SOFC anode. The quantitative phase measurement leads to a new composition analysis technique that is complimentary to the conventional fluorescence analysis. Because of its generality, our DPC algorithm will benefit microscopy systems based on other nanofocusing optics which also produce complicated pupil function25 and significantly advance the phase imaging capability for scanning x-ray microscopy.

Methods

Experiment setup

The experiment was conducted at 26-ID of Advanced Photon Source (APS) at Argonne National Laboratory. A monochromatic x-ray beam with photon energy of 12 keV was focused to a ~30 × 52 nm2 area by a pair of MLL optics placed orthogonally with respect to each other (see Figure S1 in supplementary material for the focus profiles). A set of MLLs with apertures of 13.5 um and 43.5 um were used to achieve focused flux of ~4 × 107 photon/second. An order-sorting-aperture (OSA) was not used because the focus spot was separated from the direct beam8. An energy-dispersive silicon drift detector (Ketek, 30 mm2 active area) placed at about 90° to the incident beam, was used to collect the x-ray fluorescence signal emitted by a specimen positioned at the focal plane. The x-rays transmitted through the specimen were captured by a 2D pixel-array detector (Pilatus 100 k, 487 × 195 pixels, 172 × 172 μm2 pixel size) positioned about 1.4 m downstream from the specimen.

Sample preparation

The Ni/YSZ sample was fabricated using focused ion beam (FIB) milling26 and mounted on the silicon nitride window using a modification of the commonly used "lift out" procedure27,28. The sample has a plate shape with dimensions of 12 × 8 × 1.5 μm3. First a section of a Ni/YSZ anode was mounted onto an SEM stand using carbon tape and loaded into the FIB/SEM vacuum chamber. Next, two staircase like trenches were milled into the bulk sample using the ion beam at 30 keV with a current of 21 nA, on either side of a thin strip of the bulk material that is left intact. Once the craters were milled to a depth of 10 microns, the thin strip of Ni/YSZ was then thinned to 1.5 microns beginning with a current of 2.8 nA at 30 keV and then using progressively lower beam currents for the final surface cleaning. Following this thinning, a micromanipulator was attached to the top of the thinned sample using ion beam assisted Pt deposition. Next, the thinned sample was detached from the bulk material and was moved to the silicon nitride window. Using the ion and electron SEM images, the sample was rotated and moved near the silicon nitride window until one corner of the sample was just touching the window. Pt was then deposited on the corner to lightly attach the sample to the window. The micromanipulator was then detached from the sample and was used to push over the Ni/YSZ until it was flat on the window. Finally, Pt was deposited on additional corners to hold the sample in place.

Image analysis algorithm

Assuming that the complex transmission function of the specimen is , we have,

where t is the thickness, λ is the wavelength and n is the complex refractive index. Provided that Fraunhofer diffraction condition is satisfied, the complex wave field at the detector plane at each scanned point, Dl,m, can be related to the wave field at the focal plane by a Fourier transform,

Here A is a complex number contains all constants of no importance for this study, L is the distance from the focal plane to the detector and r′ is the coordinates at the detector plane. For a small beam size, the following Taylor expansion is valid,

where both a and φ are real functions. According to Fourier transform theorem, we have,

where is the far-field diffraction intensity distribution in the absence of specimen.

For a measured 2D diffraction pattern , we seek a vector and a constant which will give the least-square error,

Based on the Parseval's equation, the integral is the same if we apply an inverse Fourier transform on the right-hand side of the equation,

Then a nonlinear fitting algorithm is employed to find the best estimates of and which will give a least-square error to the measured far-field data29, provided that the transmission function can be approximated to the simple expression shown in equation (5). The residue error, , also has a physical meaning. It contains not only background noise but also the deviation from the approximation shown in equation (5). If, for example, high order phase terms in equation (5) become more appreciable, a larger residue error is expected. Therefore, a map of the residue error indicates how dramatically the phase of the transmission function deviates from a linear variation in the range defined by the size of the focused beam. It also serves as a quantity indicating the validity of the linear approximation. Such maps are shown in Fig. S4 in supplementary materials.

In order to find a phase solution that will give a best estimate to the measured phase gradient data, we consider a continuous function consisting of a Fourier series,

Here Δx and Δy are the scan step sizes in each direction. Again we seek a solution for the complex coefficients, , which will give a least-square error,

Here and are the RMS noise of the phase gradient in each direction and w is the weight. According to the differential property of Fourier transform and the Parseval's equation, one has,

The superscript corresponds to x or y direction. Differentiating S with respect to the real and imaginary component of each coefficient and equating them to zero, we arrive at,

The coefficient for the zero frequency is determined from a known phase value at a reference point. One can prove that the commonly used Fourier integration method30 is equivalent to the special case here with w equal to 1.