Abstract
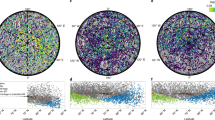
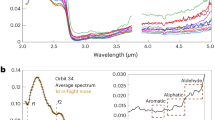
Ceres, a dwarf planet located in the main asteroid belt, has a low bulk density1, and models predict that a substantial amount of water ice is present in its mantle and outer shell2–4. The Herschel telescope and the Dawn spacecraft5 have observed the release of water vapour from Ceres6,7, and exposed water ice has been detected by Dawn on its surface at mid-latitudes8. Water molecules from endogenic and exogenic sources can also be cold-trapped in permanent shadows at high latitudes9–11, as happens on the Moon12,13 and Mercury14,15. Here we present the first image-based survey of Ceres’s northern permanent shadows and report the discovery of bright deposits in cold traps. We identify a minimum of 634 permanently shadowed craters. Bright deposits are detected on the floors of just 10 of these craters in multi-scattered light. We spectroscopically identify one of the bright deposits as water ice. This detection strengthens the evidence that permanently shadowed areas have preserved water ice on airless planetary bodies.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 digital issues and online access to articles
$119.00 per year
only $9.92 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Park, R. S. et al. Interior structure of dwarf planet Ceres from measured gravity and shape. Nature 537, 515–517 (2016).
Thomas, P. C. et al. Differentiation of the asteroid Ceres as revealed by its shape. Nature 437, 224–226 (2005).
Zolotov, M. Y. On the composition and differentiation of Ceres. Icarus 204, 183–193 (2009).
Castillo-Rogez, J. & McCord, T. B. Ceres’ evolution and present state constrained by shape data. Icarus 205, 443–459 (2010).
Russell, C. T. & Raymond, C. A. The Dawn mission to Vesta and Ceres. Space Sci. Rev. 163, 3–23 (2011).
Kìppers, M. et al. Localized sources of water vapour on the dwarf planet (1) Ceres. Nature 505, 525–527 (2014).
Nathues, A. et al. Sublimation in bright spots on (1) Ceres. Nature 528, 237–240 (2015).
Combe, J.-Ph . et al. Detection of local H2O exposed at the surface of Ceres. Science 353, 3010 (2016).
Arnold, J. R. Ice in the lunar polar regions. J. Geophys. Res. 84, 5659–5668 (1979).
Hayne, P. O. & Aharonson, O. Thermal stability of ice on Ceres with rough topography. J. Geophys. Res. 120, 1567–1584 (2015).
Schorghofer, N. et al. The permanently shadowed regions of dwarf planet Ceres. Geophys. Res. Lett. 43, 6783–6789 (2016).
Feldman, W. C. et al. Fluxes of fast and epithermal neutrons from Lunar Prospector: Evidence for water ice at the lunar poles. Science 281, 1496–1500 (1998).
Colaprete, A. et al. Detection of water in the LCROSS ejecta plume. Science 330, 463–468 (2010).
Paige, D. A. et al. Thermal stability of volatiles in the north polar region of Mercury. Science 339, 300–303 (2013).
Chabot, N. L. et al. Images of surface volatiles in Mercury’s polar craters acquired by the MESSENGER spacecraft. Geology 42, 1051–1054 (2014).
Sierks, H. et al. The Dawn Framing Camera. Space Sci. Rev. 163, 263–327 (2011).
Schorghofer, N. Predictions of depth-to-ice on asteroids based on an asynchronous model of temperature, impact stirring, and ice loss. Icarus 276, 88–95 (2016).
Watson, K. et al. The behavior of volatiles on the lunar surface. J. Geophys. Res. 66, 3033–3045 (1961).
Chabot, N. L. et al. Areas of permanent shadow in Mercury’s south polar region ascertained by MESSENGER orbital imaging. Geophys. Res. Lett. 39, L09204 (2012).
Mazarico, E. et al. Illumination conditions of the lunar polar regions using LOLA topography. Icarus 211, 1066–1081 (2011).
Preusker, F. et al. Dawn at Ceres – shape model and rotational state. 47th Lunar and Planetary Science Conf. abstract 1954 (2016).
Zhang, J. A. & Paige, D. A. Cold-trapped organic compounds at the poles of the Moon and Mercury: Implications for origins. Geophys. Res. Lett. 36, L16203 (2009).
Skoglov, E., Magnusson, P. & Dahlgren, M. Evolution of the obliquity of ten asteroids. Planet. Space Sci. 44, 1177–1183 (1996).
Hiesinger, H. et al. Cratering on Ceres: Implications for its crust and evolution. Science 353, 4759 (2016).
Crider, D. H. & Vondrak, R. R. Space weathering effects on lunar cold trap deposits. J. Geophys. Res. 108, 5079 (2003).
Crider, D. & Killen, R. M. Burial rate of Mercury’s polar volatile deposits. Geophys. Res. Lett. 32, L12201 (2005).
Fanale, F. P. & Salvail, J. R. The water regime of asteroid (1) Ceres. Icarus 82, 97–110 (1989).
De Sanctis, M. C. et al. Ammoniated phyllosilicates with a likely outer Solar System origin on (1) Ceres. Nature 528, 241–244 (2015).
Schröder, S. E. et al. In-flight calibration of the Dawn Framing Camera. Icarus 226, 1304–1317 (2013).
Anderson, J. A. et al. Modernization of the Integrated Software for Imagers and Spectrometers. 35th Lunar and Planetary Science Conf. abstract 2039 (2004).
Keszthelyi, L. et al. Support and future vision for the Integrated Software for Imagers and Spectrometers (ISIS). 44th Lunar and Planetary Science Conf. abstract 2546 (2013).
Bussey, D. B. et al. Illumination conditions at the lunar south pole. Geophys. Res. Lett. 26, 1187–1190 (1999).
Speyerer, E. J. & Robinson, M. S. Persistently illuminated regions at the lunar poles: Ideal sites for future exploration. Icarus 222, 122–136 (2013).
van Hemelrijck, E. The oblateness effect on the solar radiation incident at the top of the atmosphere of the outer planets. Icarus 51, 39–50 (1982).
Vasavada, A. R. et al. Near-surface temperatures on Mercury and the Moon and the stability of polar ice deposits. Icarus 141, 179–183 (1999).
Byrne, S. & Ingersoll, A. P. A sublimation model for Martian south polar ice features. Science 299, 1051–1053 (2003).
Michael, G. G. & Neukum, G. Planetary surface dating from crater size–frequency distribution measurements: Partial resurfacing events and statistical age uncertainty. Earth Planet. Sci. Lett. 294, 223–229 (2010).
Michael, G. G. et al. Planetary surface dating from crater size-frequency distributions: Poisson timing analysis. Icarus 277, 279–285 (2016).
Acknowledgements
We acknowledge the outstanding work of the Dawn design, engineering and operations team. We thank NASA, the Max Planck Society, and the German and Italian Space Agencies for support for this investigation.
Author information
Authors and Affiliations
Contributions
T.P. designed the concept of this study, developed the threshold methodology, performed the image processing and prepared the manuscript. A.N., N.Scho., F.P., E.M., S.E.S., M.S., M.H., P.G.-M., W.D., J.R., K.-D.M. and C.T.R. contributed to the development of data analysis and interpretation. S.B. and M.E.L. performed the temperature calculations. Crater analysis and impact simulation were performed by T.K. and N.Schm., respectively. J.-P.C. and G.S.T. analysed the VIR data. All authors discussed and contributed to the preparation of this manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supporting Information
Supplementary Figures 1–8 with captions, Supplementary Table 1 with caption, captions for Supplementary Videos 1 and 2. (PDF 2318 kb)
Supplementary Video 1
Supplementary Video 1 (GIF 14067 kb)
Supplementary Video 2
Supplementary Video 2 (MOV 34209 kb)
Rights and permissions
About this article
Cite this article
Platz, T., Nathues, A., Schorghofer, N. et al. Surface water-ice deposits in the northern shadowed regions of Ceres. Nat Astron 1, 0007 (2017). https://doi.org/10.1038/s41550-016-0007
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41550-016-0007
This article is cited by
-
Future Directions for the Investigation of Surface-Bounded Exospheres in the Inner Solar System
Space Science Reviews (2023)
-
Surface Exospheric Interactions
Space Science Reviews (2023)
-
Water Group Exospheres and Surface Interactions on the Moon, Mercury, and Ceres
Space Science Reviews (2021)
-
GAUSS - genesis of asteroids and evolution of the solar system
Experimental Astronomy (2021)
-
Micro cold traps on the Moon
Nature Astronomy (2020)