Main

The spin carries a quantum bit of information, which makes spin-based devices, also called spintronic devices, extremely promising for quantum-information processing8. More specifically, the spin Hall effect (SHE) has recently raised an increasing interest owing to its potentialities in spintronics2,3. In a similar way to the Hall effect, in which a magnetic field causes a particle to deviate depending on its charge, the spin Hall effect results in a spin current owing to the spin-dependent scattering of electrons by charged impurities or other defects (extrinsic SHE1,9) or to the spin–orbit effects on the carrier energy dispersion (intrinsic SHE10,11). It leads to a separation in real space and momentum space between spin-up and spin-down electrons and thus enables control of electron spin in semiconductor spintronic devices12. Optical2,3 and electronic4 measurements of the extrinsic SHE have been reported. Although successfully generated, electronic spin currents are subject to rapid dephasing and decay, mostly due to electron scattering. Recent theoretical studies5,13 show that the intrinsic SHE is fully suppressed in macroscopic-sized samples because of electron scattering, which directs the system towards equilibrium. The use of neutral particles as carriers for spin currents is an alternative approach that may overcome the problem of dephasing. Here, we demonstrate the spin currents carried by (neutral) exciton–polaritons in a semiconductor microcavity and propagating coherently over 100 μm. We demonstrate for the first time experimentally that the spin currents can be controlled by an optical spin Hall effect (OSHE), which exhibits remarkable analogy to the spin Hall effect.

Exciton–polaritons (or polaritons for short) are the ‘mixed’ exciton–photon quasiparticles associated with the normal modes of semiconductor microcavities. They have been studied extensively since the discovery of strong light–matter coupling in these systems in 1992 (ref. 14). Many unique properties of polaritons are derived from their non-parabolic in-plane dispersion and composite bosonic nature7. Polaritons are particularly suitable as spin current carriers owing to their long coherence length, of the order of 100 μm (ref. 15), compared with that of electronic gases. Furthermore, the recent observation of Bose–Einstein condensation of polaritons in microcavities16 confirms their high potential for fascinating quantum coherent effects17,18.

Polaritons can be created by directly shining a laser onto the microcavity where the angle of incidence determines their in-plane wavevector. The polaritons scatter elastically with disorder in the system and redistribute around an elastic circle in reciprocal space (the modulus of the in-plane wavevector is conserved). A splitting in energy between polaritons with transverse electric and transverse magnetic linear polarizations causes different spin currents to develop in different quadrants of the elastic circle. This OSHE can be considered as the result of an effective magnetic field acting on a pseudospin.

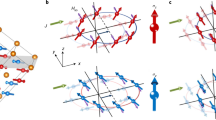
The polariton spin (angular momentum) has ±1 projections on the growth axis of the microcavity. Therefore, the polariton can be treated as a two-level system with an effective pseudospin S=1/2. Furthermore, there is a strict correspondence between the polariton pseudospin vector in the Poincaré sphere (Fig. 1a) and the polarization of light emitted by the microcavity at a given angle. Similar to an angular momentum, the polariton spin state can be controlled by an appropriate polarized excitation and can be analysed by a polarization-resolved detection. The polarization of light can be fully characterized by measuring a set of values, the so-called Stokes parameters19 that correspond to the following polarization degrees:

where l and d denote the linear polarization degree measured in the x y and diagonal axes, respectively, and c denotes the circular polarization. and I−45∘ represent the intensities measured through linear polarizers oriented at 0∘, 90∘, 45∘ and − 45∘ to the x axis; Iσ+ and Iσ− represent the intensities measured in the circular polarization basis. The OSHE is the precession of the pseudospin of the polariton around an effective magnetic field, , owing to the longitudinal–transverse splitting, ΔLT (ref. 20), which is induced by the long-range part of the exciton exchange interaction21 and by the transverse electric–transverse magnetic splitting of the photon mode of the cavity22. The value of this magnetic field is proportional to ΔLT and its direction depends on the polar angle, θ, of the polariton in-plane wavevector, k=(kx,ky) in momentum space6:

Figure 1: Angle-dependent evolution of the pseudospin vectors.
figure 1

a, The pseudospin vector represents the polariton polarization and lies on the surface of the Poincaré sphere for a pure polarized state. Initially the pseudospin lies in the x-direction (purple arrow), representing a horizontal linearly polarized state. If the effective magnetic field representing the longitudinal–transverse splitting lies in the y direction (orange arrow) then the pseudospin rotates until it is parallel to the z axis (red arrow); the initial linear polarization becomes a σ+ polarization. b, If the effective magnetic field lies in the opposite direction, then the pseudospin is rotated until it is antiparallel to the z axis (blue arrow) and the state becomes σ− polarized. c, Although the effective magnetic field always lies in the microcavity plane, its direction is dependent on the angle of the wavevector (orange arrows). The evolution of pseudospins is therefore anisotropic. For an initial linearly polarized state of polaritons around the elastic circle, the pseudospins will all lie along the x axis (not shown). After some time, all of the pseudospins point in different directions corresponding to different polarizations. Red arrows represent σ+ polarizations; blue arrows represent σ− polarizations; purple arrows represent linear polarizations.

Full size image

The evolution of a pseudospin is critically dependent on the orientation of . Figure 1a,b shows the evolution of a pseudospin having an initial linear polarization into a left (σ+) or right (σ−) circular polarization depending on the orientation of . Figure 1c shows the dependence of the orientation on the orientation of k and its consequences on the pseudospin evolution. Similar to the role of the Rashba field in the intrinsic SHE10,11, the effective magnetic field causes different polarizations to develop in different directions in the OSHE. Recently, the existence of this field has been demonstrated in experiments on linear polarization conversion in the ballistic transport of exciton–polaritons23,24.

The evolution of the pseudospin can be well described by the following precession equation:

The first term describes the precession of the pseudospin. The second term describes the input term due to the Rayleigh scattering from the initial state (τ1 is the Rayleigh scattering time constant). The last term describes the radiative relaxation (τ is the polariton lifetime).

Resonant Rayleigh scattering is due to the static disorder in the microcavity25, which is inevitably present in any realistic microcavity owing to thickness variations in the cavity and Bragg mirror layers. Thus, when an initial polariton state with a given in-plane wavevector, k=(kx,ky), and a given polarization state, transverse magnetic or transverse electric, is created, it is efficiently scattered towards states with in-plane wavevectors different from the initial one and with the same polarization state. As the modulus of k is conserved, this scattering gives rise to the Rayleigh scattering ring in the far-field emission26,27. Then the anisotropy in the orientation of the effective magnetic field creates an anisotropy in the polarization: in two opposite quadrants of the elastic circle, σ+ polarization appears, whereas in the two others, σ− polarization appears (see Fig. 1c). The spin separation on the elastic circle leads to spin separation in real space owing to ballistic propagation of the polaritons, thus the polariton spin currents are formed.

To detect the OSHE, we use the experimental set-up shown schematically in Fig. 2. We use a continuous titanium-sapphire pump laser at 836 nm with an angle of incidence of 12∘. The polarization of the pump is linear and can be adjusted to transverse magnetic or transverse electric polarization. The far-field emission transmitted by the microcavity is polarization resolved and imaged on a 1,024×1,024 CCD (charge-coupled device) camera. This set-up allows a precise measurement of the Stokes parameters of the light in the far-field, thus providing information on the pseudospin of a polariton state with a given in-plane wavevector. The polariton spin currents in real space have been detected using a near-field polarization-resolved set-up. A complete description of the experiment is available in the Supplementary Information.

Figure 2: Experimental set-up.
figure 2

A linearly polarized pump beam, kp, with an incidence angle of 12∘ is focused on the microcavity sample. The microcavity emission is collimated by a lens (f=50 mm). The emission is then resolved in polarization and split into two parts. One is imaged on a CCD camera to obtain the far-field. The other is sent through a lens to another CCD camera to obtain the near-field. PBS: Polarizing beam splitter.

Full size image

The microcavity was excited by a transverse magnetic polarized pump resonant with the lower polariton branch with a slight cavity–exciton detuning of 0.3 meV. The intensities Iσ+ and Iσ− of the light transmitted by the microcavity were imaged and measured successively using a CCD camera (Fig. 3a,b). The value of the circular Stokes parameter, ρc, was calculated for each pixel using equation (1). The result is shown in Fig. 3c. The dependence on θ of the circular parameter is in excellent agreement with the theoretical predictions shown in Fig. 1c. The maxima are located at ±π/4 and ±3π/4 and the sign of ρc is reversed between π/4 and 3π/4. For our experimental values of ΔLT (0.05 meV) and τ (∼10 ps), the maximal expected value of ρc is then close to 0.45 in excellent agreement with our experimental value of 0.48±0.03. Similar results are shown in Fig. 3d, where the experiment is carried out in the same conditions but with a transverse electric polarized excitation. The sign of ρc is then clearly reversed. We have also checked that ρc is very close to zero on the whole far-field if another spot with ΔLT=0 of the microcavity sample is excited. These experimental results are also in very good agreement with numerical simulations based on the solution of a two-component Schrödinger equation for the exciton polaritons that accounts for the disorder potential responsible for the Rayleigh scattering (Fig. 3e,f). The details of our formalism are given in the Supplementary Information.

Figure 3: Observation of the OSHE in reciprocal space and comparison with theory.
figure 3

a, The σ+ component of the emission from the microcavity in the far-field. The intensity scale is linear. The transverse magnetic (x) polarized pump beam (on the right) and the backscattering (on the left) are blocked to protect the CCD from over exposure. The polaritons undergo resonant Rayleigh scattering and become distributed around an elastic circle. The speckles are a characteristic of Rayleigh scattering in microcavities24. b, The complementary σ− polarized far-field emission. c, The measured degree of circular polarization, ρc, in the far-field emission for a transverse magnetic (x) polarized pump. The colour scale is linear, distinguishing σ+ polarizations in red and σ− polarizations in blue. The anisotropy in the polarized emission is in agreement with Fig. 1. d, The same as in c but for a transverse electric (y) polarized pump. Note that the regions of σ+ and σ− polarizations are interchanged. e, Prediction of the far-field polarization degree from numerical solution of the two-component Schrödinger equation with a random potential for the case of a transverse magnetic (x) polarized pump. The polarization degrees vary in an identical way around the elastic circle to that measured in c. f, The same as in e but for a transverse electric (y) polarized pump. Again there is an excellent agreement with the experimental result (d). In our calculation, we used the following parameters: kp=1,560 mm−1, ΔLT(kp)=0.05 meV, τ=10 ps, cavity photon effective mass, mC=10−5me (me is the free electron mass), exciton effective mass, mexc=0.22me, cavity–exciton detuning, δ=0.3 meV, Rabi splitting=5.1 meV and pump spot diameter 50 μm. The disorder potential was generated using the procedure in ref. 28 to generate a single-layer disorder potential. The potential had an r.m.s. amplitude of 0.5 meV and an isotropic correlation length of 0.75 μm.

Full size image

Figure 4a shows the near-field images of the circular polarization degree of emission by the microcavity excited by transverse magnetic polarized light. It can be seen that the polaritons carrying the σ+ or σ− polarization propagate ballistically over about 100 μm, which means that macroscopic spin currents are generated. Their direction is controlled by the polarization of excitation light. This observation is in excellent agreement with the results of the simulation (Fig. 4b).

Figure 4: Polariton spin currents in the real space.
figure 4

a, The circular polarization degree of the spatially resolved secondary emission by the microcavity for the case of a transverse magnetic polarized pump. b, Results of simulation obtained by a numerical Fourier transform of the polariton distributions in the reciprocal space (Fig. 3). Near the centre of the pump spot, any circular polarization of polaritons is overwhelmed by the linear polarization of the pump. However, once polaritons have migrated from the centre of the pump spot, their polarization depends on the orientation of their wavevector; the OSHE causes the different spin polarizations to separate in real space on the edges of the pump spot. The directions of the σ+ and σ− spin currents are shown by black arrows.

Full size image

In the OSHE, the spin currents are carried by electrically neutral exciton–polaritons, which are subject to bosonic statistics. This represents two important advantages with respect to the electronic SHE: there is no dephasing owing to the Coulomb repulsion of the carriers and there is a possibility to create superfluid spin currents. Clearly, our microcavity acts as an optically controlled spin generator allowing for integration into photonic circuits. Future research is needed to investigate the OSHE in the nonlinear regime and superfluid spin currents.