Abstract
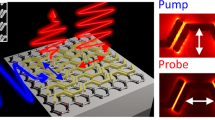
The success of metal-based plasmonics for manipulating light at the nanoscale has been empowered by imaginative designs and advanced nano-fabrication. However, the fundamental optical and electronic properties of elemental metals, the prevailing plasmonic media, are difficult to alter using external stimuli. This limitation is particularly restrictive in applications that require modification of the plasmonic response at sub-picosecond timescales. This handicap has prompted the search for alternative plasmonic media1,2,3, with graphene emerging as one of the most capable candidates for infrared wavelengths. Here we visualize and elucidate the properties of non-equilibrium photo-induced plasmons in a high-mobility graphene monolayer4. We activate plasmons with femtosecond optical pulses in a specimen of graphene that otherwise lacks infrared plasmonic response at equilibrium. In combination with static nano-imaging results on plasmon propagation, our infrared pump–probe nano-spectroscopy investigation reveals new aspects of carrier relaxation in heterostructures based on high-purity graphene.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Boltasseva, A. & Shalaev, V. M. All that glitters need not be gold. Science 8, 1086–1101 (2014).
MacDonald, K. F., Samson, Z. L., Stockman, M. I. & Zheludev, N. I. Ultrafast active plasmonics. Nature Photon. 3, 55–58 (2009).
Atwater, H. A. The promise of plasmonics. Sci. Am. 296, 56–62 (2007).
Wang, L. et al. One-dimensional electrical contact to a two-dimensional material. Science 342, 641–617 (2013).
Grigorenko, A. N., Polini, M. & Novoselov, K. S. Graphene plasmonics. Nature Photon. 6, 749–758 (2012).
Basov, D. N., Fogler, M. M., Lanzara, A., Wang, F. & Zhang, Y. Colloquium: graphene spectroscopy. Rev. Mod. Phys. 86, 959–993 (2014).
Javier García de Abajo, F. et al. Graphene plasmonics: challenges and opportunities. ACS Photon. 1, 135–152 (2014).
Fei, Z. et al. Gate-tuning of graphene plasmons revealed by infrared nano-imaging. Nature 487, 82–85 (2012).
Chen, J. et al. Optical nano-imaging of gate-tunable graphene plasmons. Nature 487, 77–81 (2012).
Woessner, A. et al. Highly confined low-loss plasmons in graphene–boron nitride heterostructures. Nature Mater. 14, 421–425 (2014).
Principi, A. et al. Plasmon losses due to electron–phonon scattering: the case of graphene encapsulated in hexagonal boron nitride. Phys. Rev. B 90, 165408 (2014).
Echtermeyer, T. J. et al. Strong plasmonic enhancement of photovoltage in graphene. Nature Commun. 2, 458 (2011).
Koppens, F. H. L. et al. Photodetectors based on graphene, other two-dimensional materials and hybrid systems. Nature Nanotech. 9, 780–793 (2014).
Mak, K. F., Ju, L., Wang, F. & Heinz, T. F. Optical spectroscopy of graphene: from the far infrared to the ultraviolet. Solid State Comm. 152, 1341–1349 (2012).
Brida, D. et al. Ultrafast collinear scattering and carrier multiplication in graphene. Nature Commun. 4, 1987 (2014).
Wagner, M. et al. Ultrafast and nanoscale plasmonic phenomena in exfoliated graphene revealed by infrared pump-probe nanoscopy. Nano Lett. 14, 894–900 (2014).
Wagner, M. et al. Ultrafast dynamics of surface plasmons in InAs by time-resolved infrared nanospectroscopy. Nano Lett. 14, 4529–4534 (2014).
Eisele, L. et al. Ultrafast multi-terahertz nano-spectroscopy with sub-cycle temporal resolution. Nature Photon. 8, 841–845 (2014).
Fei, Z. et al. Infrared nanoscopy of Dirac plasmons at the graphene-SiO2 interface. Nano Lett. 11, 4701–4705 (2011).
Dai, S. et al. Tunable phonon polaritons in atomically thin van der Waals crystals of boron nitride. Science 343, 1125–1129 (2014).
Lui, C. H., Mak, K. F., Shan, J. & Heinz, T. F. Ultrafast photoluminescence from graphene. Phys. Rev. Lett. 105, 127404 (2010).
Ulbricht, R., Hendry, E., Shan, J., Heinz, T. F. & Bonn, M. Carrier dynamics in semiconductors studied with time-resolved terahertz spectroscopy. Rev. Mod. Phys. 83, 543–586 (2011).
Frenzel, A. J., Lui, C. H., Shin, Y. C., Kong, J. & Gedik, N. Semiconducting-to-metallic photoconductivity crossover and temperature-dependent Drude weight in graphene. Phys. Rev. Lett. 113, 056602 (2014).
Gierz, I. et al. Snapshots of non-equilibrium Dirac carrier distributions in graphene. Nature Mater. 12, 1119–1124 (2013).
Caldwell, J. D. et al. Sub-diffractional, volume-confined polaritons in a natural hyperbolic material: hexagonal boron nitride. Nature Commun. 5, 5221 (2014).
Ni, G. X. et al. Plasmons in graphene moiré superlattices. Nature Mater. 14, 1217–1222 (2015).
Winnerl, S. et al. Carrier relaxation in epitaxial graphene photoexcited near the Dirac point. Phys. Rev. Lett. 107, 237401 (2011).
Kashuba, A. B. Conductivity of defectless graphene. Phys. Rev. B 78, 085415 (2008).
Briskot, U. et al. Collision-dominated nonlinear hydrodynamics in graphene. Phys. Rev. B 92, 115426 (2015).
Das, A. et al. Monitoring dopants by Raman scattering in an electrochemically top-gated graphene transistor. Nature Nanotech. 3, 210–215 (2008).
Acknowledgements
We thank P. Kim, Z. Sun, A. Sternbach, S. Dai and J.-S. Wu for helpful discussions. Research on static plasmon interferometry of high-mobility graphene is supported by DOE-BES DE-FG02-00ER45799. Work on ultrafast imaging of non-equilibrium plasmons is supported by ONR N00014-15-1-2671. The development of ultrafast pump–probe spectroscopy is supported by DOE-BES DE-SC0012592 and DE-SC0012376. The development of nano-imaging is supported by AFOSR and ARO. D.N.B is supported by the Gordon and Betty Moore Foundation's EPiQS Initiative through Grant GBMF4533. J.H. acknowledges support from ONR N00014-13-1-0662. G.X.N., B.O., and A.H.C.N. acknowledge the National Research Foundation, Prime Minister Office, Singapore, under its Medium Sized Centre Program and CRP award ‘Novel 2D materials with tailored properties: beyond graphene’ (R-144-000-295-281). B.O. acknowledge NRF-Competitive Research Programme (CRP award no. NRF-CRP9-2011-3).
Author information
Authors and Affiliations
Contributions
All authors were involved in designing the research performing the research and writing the paper.
Corresponding author
Ethics declarations
Competing interests
F.K. is one of the cofounders of Neaspec, producer of the s-SNOM apparatus used in this study.
Supplementary information
Supplementary information
Supplementary information (PDF 2202 kb)
Rights and permissions
About this article
Cite this article
Ni, G., Wang, L., Goldflam, M. et al. Ultrafast optical switching of infrared plasmon polaritons in high-mobility graphene. Nature Photon 10, 244–247 (2016). https://doi.org/10.1038/nphoton.2016.45
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphoton.2016.45
This article is cited by
-
Manipulating hyperbolic transient plasmons in a layered semiconductor
Nature Communications (2024)
-
Ferroelectric-controlled graphene plasmonic surfaces for all-optical neuromorphic vision
Science China Technological Sciences (2023)
-
Observation of chiral and slow plasmons in twisted bilayer graphene
Nature (2022)
-
Active control of micrometer plasmon propagation in suspended graphene
Nature Communications (2022)
-
Two-dimensional Dirac plasmon-polaritons in graphene, 3D topological insulator and hybrid systems
Light: Science & Applications (2022)