Heat capacity peak at the quantum critical point of the transverse Ising magnet CoNb₂O₆

Abstract

The transverse Ising magnet Hamiltonian describing the Ising chain in a transverse magnetic field is the archetypal example of a system that undergoes a transition at a quantum critical point (QCP). The columbite CoNb₂O₆ is the closest realization of the transverse Ising magnet found to date. At low temperatures, neutron diffraction has observed a set of discrete collective spin modes near the QCP. Here, we ask if there are low-lying spin excitations distinct from these relatively high-energy modes. Using the heat capacity, we show that a significant band of gapless spin excitations exists. At the QCP, their spin entropy rises to a prominent peak that accounts for 30% of the total spin degrees of freedom. In a narrow field interval below the QCP, the gapless excitations display a fermion-like, temperature-linear heat capacity below 1 K. These novel gapless modes are the main spin excitations participating in, and affected by, the quantum transition.

Introduction

In the transverse Ising magnet (TIM), a magnetic field applied transverse to the easy axis of the spins induces a zero-Kelvin phase transition from the magnetically ordered state to the disordered state. Because it is the archetypal example of a system displaying quantum critical behaviour¹, the TIM is prominently investigated in many areas of topical interest, for example, quantum magnetism^2,3, integrable field theories^4,5 and investigations of novel topological excitations^6,7,8. The columbite CoNb₂O₆ is the closest realization found to date of the TIM in a real material. The spin excitations have been investigated by neutron diffraction spectroscopy near the quantum critical point (QCP)³ and in the paramagnetic state⁹, THz spectroscopy¹⁰ and ⁹³Nb nuclear magnetic resonance¹¹, but little is known about their thermodynamic properties at the QCP. Are there low-lying spin excitations distinct from the neutron-excited modes? What are their characteristics at the QCP?

Here we report a low-temperature heat capacity experiment that addresses these questions. We establish the existence of a large population of spin excitations that are gapless (after the phonon contribution is subtracted). As the transverse magnetic field is tuned towards the QCP, the spin heat capacity rises to a prominent peak. Below 1 K, the gapless modes display a temperature (T)-linear heat capacity similar to fermionic excitations. From the spin entropy, we infer that, at 1 K, the gapless modes account for of the total spin degrees of freedom.

Results

Heat capacity versus temperature

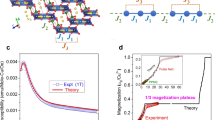
In CoNb₂O₆, the stacking of edge-sharing CoO₆ octahedra along the c axis defines the Ising chain (inset in Fig. 1c). The isolated chain is described by the TIM Hamiltonian:

Figure 1: The heat capacity of CoNb₂O₆ versus temperature measured in a transverse magnetic field.

The field H is applied parallel to the b axis. The quantity plotted is the heat capacity C divided by temperature T. (a) Curves of C/T versus T at fixed H<H_c (=5.24 T). In field H=0, a transition to an incommesurate phase occurs at T_c(0)=2.85 K. With increasing H, the transition T_c(H) is decreased. Below 1 K, C/T approaches saturation instead of decreasing to 0. At 5 T, C/T is T independent below 0.8 K. (b) Behaviour of C/T in the paramagnetic state (H>H_c). Just above H_c (curve at H=5.3 T), C/T falls montonically as T increases above 0.5 K. For H slightly above 5.6 T, a field-dependent gap Δ appears. (c) the crystal structure of CoNb₂O₆. The easy axis (red arrows) is in the a–c plane at an angle ±31° to the c axis.

with J₀ the ferromagnetic exchange along the easy axis c||x and Γ the transverse field. S_n^x and S_n^z are, respectively, the x and z components of the spin operator at lattice site n. In the a–b plane, the chains assume a triangular coordination^12,13,14, with antiferromagnetic interactions |J₁|, |J₂|<<J₀ between adjacent chains. Geometric frustration effects lead to competing antiferromagetic and ferrimagnetic ground states¹⁴. In a magnetic field H||b, CoNb₂O₆ exhibits a sharp transition to a three-dimensional (3D)-ordered phase at a critical temperature T_c(H) that decreases from 2.85 K (at H=0) to zero as H→H_c.

To investigate the low-energy spin excitations in CoNb₂O₆, we have measured its low-temperature heat capacity C(T,H) by a.c. calorimetry over the T–H plane (see Methods). First, we discuss the curves of the heat capacity C versus T measured in fixed H. Figure 1a plots these curves as C/T(T,H) versus T for H<H_c=5.24 T. In each curve, C/T displays a prominent peak when T crosses T_c(H). In zero H, C/T decreases steeply below T_c(0), and approaches zero at 1 K, consistent with the existence of a full gap. The shoulder feature near 1.7 K signals the transition from an incommensurate to commensurate AF (antiferromagnetic) phase¹³. At finite H, we observe significant enhancement of C/T throughout the ordered phase. Instead of falling to zero, the curves become T independent at low T (curve at 5 T). Between 4 and 5 T, the saturation value increases by more than a factor of 3. In the disordered phase (H>H_c), we observe a profile that also reveals a gap Δ, but one that increases sharply with the reduced field H−H_c (Fig. 2a).

Figure 2: Phase diagram of CoNb₂O₆ in a transverse magnetic field and heat capacity peak at the QCP.

(a) The phase diagram inferred from C/T. The transition T_c(H) defines the ordered phase (solid circles and triangles represent T-constant and H-constant measurements, respectively). Gapless excitations with T-independent C/T are observed in the blue shaded region (4.2 T<H<H_c). The gap Δ above H_a (solid diamonds) is inferred from fits to the one-dimensional exact solution. The error bars are estimated from the goodness of the fits at each H. H_a is the crossover field at which d(C/T)/dT changes sign at low T. The dashed curves are nominal boundaries of the low-T phases in which glassy behaviour is observed. (b) Curves of C/T measured versus H at constant T. Above ∼1 K, C/T climbs to a sharp peak when H crosses the boundary T_c(H), and then falls monotonically. Below 1 K, however, the constant-T contours lock to the left branch of the critical peak profile (C/T)₀ measured at 0.45 K (which peaks at H_c=5.24 T). The locking implies C/T is T-independent below 0.8 K. In both panels, the blue shaded regions represent the field interval within which the locking is observed. Above H_c, the contours are well separated at all T. The derivative d(C/T)/dT at low T is negative for H_c<H<H_a (yellow region), but positive for H>H_a.

Heat capacity versus field

To supplement the constant-H curves in Fig. 1, we performed measurements of C/T versus H at constant T. Figure 2a shows the phase diagram obtained from combining the constant-H and constant-T curves. The boundary of the ordered phase, T_c(H) defined by the sharp peak in C/T, falls to zero as H→H_c (solid circles and triangles). Above H_c, the gap Δ in the disordered phase (solid diamonds) is estimated from fits to the free-fermion solution discussed below. The dashed curves are the nominal boundaries below which glassy behaviour is observed (see below).

Figure 2b displays the constant-T scans in the region close to the QCP. If the temperature is fixed at a relatively high value, for example, T=1.76 K, C/T(T,H) initially rises to a sharp peak as H is increased from 0 to 4.1 T. Above 4.1 T, C/T falls monotonically with no discernible feature at H_c. As we lower T, the peak field shifts towards H_c=5.24 T, tracking T_c(H) in the phase diagram in Fig. 2a. Below 1.5 K, the constant-T contours converge towards the prominent profile measured at 0.45 K, which is our closest approximation to the critical peak profile (C/T)₀ at T=0. The contours under this profile reveal a remarkable structure. On the low-field side of the peak (4.2 T<H<H_c), shaded blue in Fig. 2a,b, the contours lock to the critical peak profile as T decreases. This implies that, if H is fixed inside this interval, C/T assumes the T-independent value (C/T)₀ at low T. Hence the T-independent plateau seen in the curve at 5 T in Fig. 1a is now seen to extend over the entire blue region. When H exceeds H_c, however, the locking pattern vanishes. The different T dependencies reflect the distinct nature of the excitations on either side of H_c. (Slightly above H_c, the derivative d(C/T)/dT changes from negative to positive at a crossover field H_a∼5.6 T.)

Spectrum of C_eff and glassy response

In a.c. calorimetry, the spectrum of the effective (observed) heat capacity C_eff(ω) varies in a characteristic way with the measurement frequency ω. For each representative local region of the T–H phase diagram investigated, we measured over the frequency range 0.02–100 Hz, where P₀ is the applied power and is the complex temperature (see Methods). The spectrum of C_eff(ω) has a hull-shaped profile characterized by the two characteristic times τ₁ (set by the sample’s parameters) and τ_ext set by coupling with the bath (defined in Methods). In both the low-ω and high-ω regions (ω<<1/τ_ext and ω>>1/τ₁, respectively) C_eff(ω) rises steeply above the true (equilibrium) heat capacity C. However, there exists a broad frequency range in between where C_eff(ω) is nearly ω-independent and equal to the intrinsic equilibrium heat capacity C of the sample. All results reported here are taken with ω within this sweet spot. Within the regions denoted as glassy in Fig. 2, the spectrum is anomalous (Methods). Instead of the hull-shaped spectrum, the measured C_eff decreases monotonically over the accessible frequency range. We define these regions of the phase diagram as glassy. We note that the QCP region lies well away from the glassy regions.

Temperature-linear heat capacity at critical field

To make explicit the T-independent behaviour below H_c, we have extracted the values of C/T(T,H) and replotted them in Fig. 3 as constant-H curves for eight values of H between 4 and 5.2 T. As is evident, the curves approach a constant value when T decreases below 0.8 K. The flat profiles reflect the locking of the contours described above. We have also plotted the constant-H curves measured at 4, 4.5 and 5 T (continuous curves) to show the close agreement between the two sets of data. The critical peak profile in Fig. 2b and the T-independent contours shown in Fig. 3 are our key findings in this report.

Figure 3: Low-temperature heat capacity and spin entropy in expanded scale near the critical field.

The discrete symbols (solid circles) are values of C/T extracted from continuous measurements of C/T versus H at fixed T (the constant-T scans plotted in the phase diagram). Here they are plotted versus T at fixed H to bring out the fixed-field contours. Below 0.8 K, the values of C/T saturate to a T-independent value that depends on H. These plateau values occur within the region of the phase diagram where the contours display locking behaviour. To supplement the discrete data points, we also measured C/T continuously versus T at fixed H. These curves are shown as continuous curves at H=4.0, 4.5 and 5.0 T. The agreement between the two distinct experiments is very close.

The T-linear behaviour of C at low T illuminates the nature of the low-lying excitations. For fermions, C is linear in T and given by , where is the density of states at the Fermi level. The Hamiltonian equation (1) can be diagonalized by transforming to free fermions^15,16 (see Free-fermion solution in Methods). The heat capacity of the isolated Ising chain may then be calculated¹⁷. The issue whether the free fermions are artifacts or real observables is currently debated^6,7. However, our system is 3D with finite J₁ and J₂. To our knowledge, fermionic excitations in the ordered phase have not been anticipated theoretically.

Low-temperature spin entropy at critical field

We next show that the critical peak profile ((C/T)₀ in Fig. 2b) accounts for a surprisingly large fraction of the total spin degrees of freedom (d.o.f.). Before extracting the spin entropy from the measured C, we need to subtract the phonon contribution. Fortunately, the spin contributions may be readily distinguished from the phonon term. Following the procedure of Hanawa et al.¹², we have carried out this subtraction to isolate the spin part of the heat capacity C_s(T,H) (see Methods). The spin entropy is then given by the integral .

First, we verified that, at H=0, the curve of S_s(T) obtained by integrating C_s/T rises rapidly above 20 K to closely approach the value R ln 2 (R is the universal gas constant), thus accounting for the total spin d.o.f. By integrating C_s/T with respect to T, we obtain the total spin entropy S_s(T). In Fig. 4a, the variation of S_s(T) versus T inferred from the data at zero H is plotted. Above ∼5 K, S_s rises rapidly attaining 90% of R ln 2 by 20 K.

Figure 4: The spin entropy of CoNb₂O₆ derived from its heat capacity.

To obtain the spin entropy S_s, we first subtract the phonon contribution from the measured heat capacity to isolate the spin contribution C_s. The spin entropy S_s(T) is then obtained by integrating C_s(T)/T with respect to T. (a) The profile of S_s in zero magnetic field. At 20 K, 90% of the spin entropy frozen out at low T is recovered. Although our measurements extend only to 30 K, the curve of S_s is expected to asymptote to 1 above room temperature. (b) The spin entropy S_s versus T at H=0, 5 and 8 T. S_s is obtained by integrating the curves of C_s/T after subtracting the phonon contribution (derived from the results of Hanawa et al. on the nonmagnetic analogue ZnNb₂O₆). At 5 T, S_s accounts for nearly of the spin d.o.f. at 1 K.

Our interest here is the behaviour of S_s at low T, which we plot in Fig. 4b. In contrast to S_s(T) at H=0 and 8 T, the curve for S_s(T) at 5 T is strongly enhanced and varies linearly with T with a slope equal to C_s/T. As H→H_c, the spin entropy rises to ∼30% of R ln 2 at 1 K. Hence, the gapless excitations account for nearly of the total spin d.o.f.

Paramagnetic state heat capacity and the free-fermion solution

In the paramagnetic state above H_c, it is instructive to compare the measured C_s/T with the heat capacity calculated from the free energy in the free-fermion solution ¹⁷ (see Methods) given by

where R is the gas constant, β=1/(k_BT), and the energy of the fermions is , with λ=J₀/2Γ. For each field H>H_c, we took Γ and λ as adjustable parameters (J₀ is fixed at 21.4 K for all H).

As shown in Fig. 5, the fits (dashed curve) are reasonable only above 10 K. Below 10 K, deviations become increasingly prominent as we lower H towards H_c. In particular, the striking divergence of the curve at H=5.4 T (as T→0) lies well beyond the reach of equation (2). These deviations reveal that the incipient magnetic ordering effects extend deep into the paramagnetic phase. The curves in Figs 2 and 3 reveal how these deviations smoothly evolve into the gapless excitations. Models that include interchain exchange terms (for example, as proposed in ref. 9) are more realistic. Comparison of our data with the behaviour of the C_s predicted near the QCP should be highly instructive. To our knowledge, solutions in the quantum regime have not been reported.

Figure 5: The heat capacity in the paramagnetic phase at three values of magnetic field above the critical value.

The phonon contribution to the measured C has been subtracted. For clarity, the curves have been displaced vertically. The dashed curves are fits to the free-fermion solution using the values Γ=11, 13.24 and 16.3 K for the curves at H=5.4, 6.5 and 8 T, respectively. The corresponding values of λ are 0.97, 0.806 and 0.655. The value of J₀ is fixed at 21.4 K. Deviations from the fits become pronounced as H→H_c.

Negligible contribution from nuclear spin degrees

We discuss whether contributions of the nuclear spins to the heat capacity play any role in the experiment. The nuclear spins contribute as a Schottky term given by¹⁸ C_N=Nk_BX²e^X/(e^X+1)² (for the two-level case), where X=ΔE/k_BT, and ΔE is the energy splitting of the levels. C_N peaks near ΔE/k_B (typically 10–30 mK^18,19) and falls off as (ΔE/k_BT)²≡A/T² for T>>ΔE/k_B. The most favourable situation is when H increases ΔE by the Zeeman energy, which is ΔE=μμ_NH, where μ is the nuclear moment and μ_N=0.37 mK T⁻¹ the nuclear Bohr magneton. We have μ=6.17 for ⁹³Nb and 4.63 for ⁵⁹Co. The larger moment gives A=0.032 mJK mol⁻¹ at 5 T. At T=1 K, this yields values for C_N that are extremely small (by a factor of 10⁵) compared with C displayed in Figs 1 and 3. Hence, the nuclear spin d.o.f. cannot be resolved in our experiment.

Discussion

In the isolated Ising chain, the excitations are domain walls (kinks) that separate degenerate spin-↑ from spin-↓ domains. In our 3D system, the self-consistent fields derived from J₁ and J₂ lift the degeneracy. As a result, kinks and antikinks interact via a linear potential (the energy cost of the unfavoured domain) to form bound pairs^20,21. The quantized excitations of the bound pairs have been detected by neutron diffraction spectroscopy³ and by time-domain THz spectroscopy¹⁰ as discrete modes (the lowest mode has energy 1.2 meV at H=0 and 0.4 meV at 5 T). As H→H_c, the ratio of the two lowest modes approaches the golden ratio, consistent with the E8-Lie group spectrum³. However, these modes are too high in energy to contribute to C/T below 1 K. Rather, our experiment provides firm evidence for a band of low-lying, gapless spin excitations that are entirely distinct from the high-energy modes. The steep increase of the spin entropy S_s at 5 T (Figure 4b) shows that S_s has attained 30% of its high-T value already at 1 K. Thus, the large anomalous peak centred at the QCP accounts for a significant fraction of the total spin d.o.f. In addition to the remarkable spin modes observed at discrete energies by neutron diffraction spectroscopy and THz spectroscopy, a substantial fraction of the spin d.o.f. exists as (essentially) gapless modes, which peak in weight at the QCP. How the two sets of excitations co-exist is a problem that confronts the theoretical description of the QCP in this material.

Perhaps, the most surprising finding from the experiment is the T-independent profile of C_s(T)/T below 1 K in the field interval 4.2<H<H_c abutting H_c. The results imply that the excitations obey Fermi–Dirac statistics. In the one-dimensional TIM model, the solution obtained via the Jordan–Wigner transformation (see Methods) yield free fermions. However, as mentioned above, it is uncertain whether these fermions are physically observable. Moreover, the interchain exchange in the real material⁹ may render the free-fermion solutions inapplicable. The present finding that the T-linear behaviour is confined to the QCP region where C/T displays a prominent peak highlights serious gaps in our understanding of the QCP and the effects of strong quantum fluctuations in its vicinity. The heat capacity invites a detailed investigation of the quantum behaviour at the QCP in realistic models applicable to CoNb₂O₆.

The heat capacity experiment shows that, in the vicinity of the QCP, the gapless modes constitute the dominant spin excitations that are affected by the quantum transition induced by the applied transverse H. As seen in the set of curves in Fig. 2b, the QCP strongly affects C/T versus H to produce a profile that, at 0.45 K, rises to a prominent peak at the critical field. We reason that the gapless modes are the relevant modes that participate in the quantum transition at H_c. The dominant fluctuations associated with the quantum transition are inherent to these modes. From the spin entropy, we infer that they account for nearly of the total spin degrees of freedom in the sample. As discussed, the gapless modes display a fermion-like heat capacity below 1 K over a broad region of the ordered phase below H_c.

Methods

Crystal growth

The CoNb₂O₆ powder was packed and sealed into a rubber tube evacuated using a vacuum pump. The powder was then compacted into a rod, typically 6 mm in diameter and 70-mm long, using a hydraulic press under an isostatic pressure of 7 × 10⁷ Pa. After removal from the rubber tube, the rods were sintered in a box furnace at 1,375° C for 8 h in air.

Single crystals of ∼5 mm in diameter and 30 mm in length were grown from the feed rods in a four-mirror optical floating zone furnace (Crystal System Inc. FZ-T-4000-H-VII-VPO-PC) equipped with four 1-kW halogen lamps as the heating source. In all the growth processes, the molten zone was moved upwards with the seed crystal being at the bottom and the feed rod above it. Growths were carried out under 2 bar O₂–Ar (50/50) atmosphere with the flow rate of 50 ml min⁻¹, at the zoning rate of 2.5 mm h⁻¹, with rotation rates of 20 r.p.m. for the growing crystal (lower shaft) and 10 r.p.m. for the feed rod (upper shaft). In all runs, only one zone pass was performed.

Phase identification and structural characterization were obtained using a Bruker D8 Focus X-ray diffractometer operating with Cu K radiation and Lynxeye silicon strip detector on finely ground powder from the crystal boules, while back-reflection X-ray Laue diffraction was utilized to check the crystalline qualities and orientations of the crystals. Measurements were carried out on oriented thin rectangular-shaped samples cut directly from the crystals using a diamond wheel.

A.C. calorimetry

The heat capacity was measured using the a.c. calorimetry technique²² on a crystal of CoNb₂O₆ (∼1 × 3 × 0.5 mm along the a, b and c axes, respectively) in a magnetic field H applied along the b axis. Using an a.c. current of frequency , we applied the a.c. power (P₀/2)exp(iωt) to the sample via a 1-kΩ RuO₂ thin-film resistor (P₀ ranged from ∼0.4 to 5 μW for measurements below 4 K, and ∼5 to 400 μW from 4 to 30 K). The (complex) temperature was detected at the thermometer (a 20-kΩ RuO₂ thin-film resistor). In the slab geometry of ref. 22, is given by

where is the complex angle

Here, K_int is the thermal conductance of the sample, K_b the thermal conductance between the sample and the thermal bath and C the heat capacity of the sample.

Taking into account that the thermal conductance between the thermometer and the sample, heater and the sample is finite, and under the condition that the internal relaxation time constant (where τ_i=C/6K_int, τ_θ=C_θ/K_θ, τ_h=C_h/K_h, C_θ heat capacity of the thermometer, C_h heat capacity of the heater, K_θ thermal conductance between the thermometer and the sample, K_h thermal conductance between the heater and the sample) and the external relaxation time constant τ_ext≡C/K_b satisfy ωτ₁<<1<<ωτ_ext, equation (3) reduces to

from which the heat capacity is obtained as , where ω* is within the sweet spot (ωτ₁<<1<<ωτ_ext). The inequalities in equation (6) determine the optimal frequency ω*.

In the experiment, the measurements extended from 0.45 to 30 K in temperature and from 0 to 8 T in field. In each representative region of the T–H plane investigated, we have measured the frequency spectrum of the effective heat capacity . We carried out fits to the equations above, and found the optimal frequency ω* in each region of the T–H plane. The fit to one of the spectra is shown in Fig. 6b. The flat portion of the spectrum corresponds to the sweet spot in which C is identified with . The fits to the real and imaginary parts of equation (3) are shown in Fig. 6a.

Figure 6: Spectra of the observed complex temperature and the inferred effective heat capacity at 1 K and 6.5 T.

(a) The frequency dependence of the real (red dots) and imaginary part (black dots) of complex temperature . The thin red curves show the fits to equation (3).(b) Frequency dependence of the effective heat capcacity (black squares) and the fit (red curve). The effective heat capacity C_eff coincides with the sample heat capacity when the frequency ω* falls within the sweet spot ωτ₁<<1<<ωτ_ext. Throughout, f=ω/2π is the frequency of the a.c. power.

Figure 7a displays the spectrum of C_eff at 0.55 K at several values of H. The evolution of the sweet spot as H varies is apparent. As H approaches the critical field H_c, the sweet spot moves to lower frequencies, reflecting the increase of the heat capacity of the sample.

Figure 7: Spectra of the effective heat capacity C_eff at 0.55 K at selected fields.

(a) Frequency dependence of the sweet spot near the quantum critical point. As the magnetic field reaches the quantum critical point, the sweet spot moves to lower frequencies, reflecting the increase of the heat capacity. (b) Glassy behaviour below 3.5 T and above 7 T. Effective heat capacity C_eff decreases monotonically as the frequency increases. No sweet spot was found in this field range. For clarity, the curves have been shifted vertically to avoid overlap.

An important benefit of mapping the spectra over the entire T–H plane is that we can observe the onset of glassy behaviour. In the glass-like regions (which appear below 1 K in specific field ranges), the spectra decrease monotonically with increasing ω. The flat portion is not observed. Several traces for H below 3.5 T and above 7 T are shown in Fig. 7b (all curves are at 0.55 K). In these regimes (demarcated by the dashed curves in Fig. 2), the measured spectrum cannot be fitted to equation (3), so we cannot extract a value for C.

Phonon contribution

Below 4 K, the contribution of the phonon to the heat capacity is negligible. However, above 4 K, the phonon contribution to the observed C is substantial. To isolate the heat capacity of the spin degrees of freedom, we estimate the phonon contribution in CoNb₂O₆ as equivalent to the heat capacity in ZnNb₂O₆ measured by Hanawa et al.¹². ZnNb₂O₆ is a nonmagnetic analogue of CoNb₂O₆ with nearly identical lattice structure. In Fig. 8a, the raw curves for CoNb₂O₆ (before the phonon subtraction) are plotted. The heat capacity of ZnNb₂O₆ is also plotted alongside with a slight rescaling (by 13%) to achieve asymptotic agreement with our zero-H curve when T exceeds 25 K. The rescaling is consistent with the combined experimental uncertainties in the two experiments. Curves of the spin contribution to the heat capacity C_s(T), obtained after phonon subtraction, are plotted in Fig. 8b.

Figure 8: Behaviour of the heat capacity measured up to 30 K at selected magnetic fields.

(a) The bold curves are the total heat capacity C measured below 30 K in fixed field H=0–8 T. The thin curve (wine coloured) shows the heat capacity of the nonmagnetic analogue ZnNb₂O₆ measured by Hanawa et al. We slightly rescaled their data by ∼13% to match our measured curves (the disagreement arises from uncertainties in estimating the crystal size). The phonon contribution to the total heat capacity is negligible below 4 K. (b) The spin heat capacity C_s obtained after subtraction of the phonon contribution at the selected H.

Free-fermion solution

We use the free-fermion solution^15,17 of the one-dimensional Transverse Ising Model to calculate the heat capacity of the spin d.o.f. The TIM Hamiltonian is

with Γ the applied transverse field (along ) and J the easy-axis exchange (along ). The spin operators S_i^x and S_i^y, expressed in the combination

are converted by the Jordan–Wigner transformation into the fermion operators

H is then reduced to terms bilinear in the fermion operators. A final Bogolyubov transformation to the new fermion operators , η_k achieves diagonalization, and gives

The free-fermion excitation energy is ɛ_k=ΓΛ_k, with

The free energy is given by

with β=1/(k_BT). From F, we obtain the molar heat capacity in equation (2).