Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Article
  • Published:

Contextuality supplies the ‘magic’ for quantum computation

Nature volume 510, pages 351–355 (2014)Cite this article

Subjects

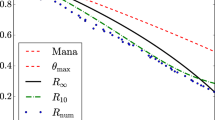
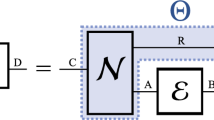
Abstract

Quantum computers promise dramatic advantages over their classical counterparts, but the source of the power in quantum computing has remained elusive. Here we prove a remarkable equivalence between the onset of contextuality and the possibility of universal quantum computation via ‘magic state’ distillation, which is the leading model for experimentally realizing a fault-tolerant quantum computer. This is a conceptually satisfying link, because contextuality, which precludes a simple ‘hidden variable’ model of quantum mechanics, provides one of the fundamental characterizations of uniquely quantum phenomena. Furthermore, this connection suggests a unifying paradigm for the resources of quantum information: the non-locality of quantum theory is a particular kind of contextuality, and non-locality is already known to be a critical resource for achieving advantages with quantum communication. In addition to clarifying these fundamental issues, this work advances the resource framework for quantum computation, which has a number of practical applications, such as characterizing the efficiency and trade-offs between distinct theoretical and experimental schemes for achieving robust quantum computation, and putting bounds on the overhead cost for the classical simulation of quantum algorithms.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Figure 1: A two-dimensional slice through qutrit state space.
Figure 2: Our construction applied to two qubits.

Similar content being viewed by others

References

  1. Shor, P. W. in Proc. IEEE Foundations of Computer Science (FOCS) (ed. Goldwasser, S. ) 124–134 http://dx.doi.org/10.1109/SFCS.1994.365700 (IEEE Computer Society, 1994)

    Google Scholar 

  2. Lloyd, S. Universal quantum simulators. Science 273, 1073–1078 (1996)

    Article  ADS  MathSciNet  CAS  MATH  PubMed  Google Scholar 

  3. Deutsch, D. Quantum theory, the Church-Turing principle and the universal quantum computer. Proc. R. Soc. A. 400, 97–117 (1985)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  4. Vidal, G. Efficient classical simulation of slightly entangled quantum computations. Phys. Rev. Lett. 91, 147902 (2003)

    Article  ADS  CAS  PubMed  Google Scholar 

  5. Datta, A., Shaji, A. & Caves, C. M. Quantum discord and the power of one qubit. Phys. Rev. Lett. 100, 050502 (2008)

    Article  ADS  PubMed  CAS  Google Scholar 

  6. Steane, A. M. A quantum computer only needs one universe. Preprint at http://arxiv.org/abs/quant-ph/0003084 (2000)

  7. Vedral, V. The elusive source of quantum speedup. Found. Phys. 40, 1141–1154 (2010)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  8. Brodutch, A. Discord and quantum computational resources. Phys. Rev. A 88, 022307 (2013)

    Article  ADS  CAS  Google Scholar 

  9. Van den Nest, M. Universal quantum computation with little entanglement. Phys. Rev. Lett. 110, 060504 (2013)

    Article  ADS  PubMed  CAS  Google Scholar 

  10. Knill, E. & Laflamme, R. Power of one bit of quantum information. Phys. Rev. Lett. 81, 5672–5675 (1998)

    Article  ADS  CAS  Google Scholar 

  11. Briegel, H. J., Browne, D. E., Dür, W., Raussendorf, R. & Van den Nest, M. Measurement-based quantum computation. Nature Phys. 5, 19–26 (2009)

    Article  CAS  Google Scholar 

  12. Bell, J. On the problem of hidden variables in quantum mechanics. Rev. Mod. Phys. 38, 447–452 (1966)

    Article  ADS  MathSciNet  CAS  MATH  Google Scholar 

  13. Kochen, S. & Specker, E. P. The problem of hidden variables in quantum mechanics. J Math. Mech. 17, 59–87 (1968)

    MathSciNet  MATH  Google Scholar 

  14. Bravyi, S. & Kitaev, A. Universal quantum computation with ideal Clifford gates and noisy ancillas. Phys. Rev. A 71, 022316 (2005)

    Article  ADS  MathSciNet  MATH  CAS  Google Scholar 

  15. Knill, E. Quantum computing with realistically noisy devices. Nature 434, 39–44 (2005)

    Article  ADS  CAS  PubMed  Google Scholar 

  16. Campbell, E. T., Anwar, H. & Browne, D. E. Magic state distillation in all prime dimensions using quantum Reed-Muller codes. Phys. Rev. X 2, 041021 (2012)

    CAS  Google Scholar 

  17. Mermin, N. D. Simple unified form for the major no-hidden-variables theorems. Phys. Rev. Lett. 65, 3373–3376 (1990)

    Article  ADS  MathSciNet  CAS  PubMed  MATH  Google Scholar 

  18. Buhrman, H., Cleve, R., Massar, S. & de Wolf, R. Nonlocality and communication complexity. Rev. Mod. Phys. 82, 665–698 (2010)

    Article  ADS  Google Scholar 

  19. Acín, A. et al. Device-independent security of quantum cryptography against collective attacks. Phys. Rev. Lett. 98, 230501 (2007)

    Article  ADS  PubMed  CAS  Google Scholar 

  20. Reichardt, B. W., Unger, F. & Vazirani, U. Classical command of quantum systems. Nature 496, 456–460 (2013)

    ADS  CAS  PubMed  Google Scholar 

  21. Vazirani, U. & Vidick, T. Fully device independent quantum key distribution. Preprint at http://arxiv.org/abs/1210.1810 (2012)

  22. Raussendorf, R. Contextuality in measurement-based quantum computation. Phys. Rev. A 88, 022322 (2013)

    Article  ADS  CAS  Google Scholar 

  23. Hoban, M. J., Wallman, J. J. & Browne, D. E. Generalized Bell-inequality experiments and computation. Phys. Rev. A 84, 062107 (2011)

    Article  ADS  CAS  Google Scholar 

  24. Gottesman, D. Theory of fault-tolerant quantum computation. Phys. Rev. A 57, 127–137 (1998)

    Article  ADS  CAS  Google Scholar 

  25. Yu, A. Fault-tolerant quantum computation by anyons. Ann. Phys. 303, 2–30 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  26. Raussendorf, R., Harrington, J. & Goyal, K. A fault-tolerant one-way quantum computer. Ann. Phys. 321, 2242–2270 (2006)

    Article  ADS  MathSciNet  CAS  MATH  Google Scholar 

  27. Dennis, E., Kitaev, A., Landahl, A. & Preskill, J. Topological quantum memory. J. Math. Phys. 43, 4452–4505 (2002)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  28. Anwar, H., Brown, B. J., Campbell, E. T. & Browne, D. E. Efficient decoders for qudit topological codes. Preprint at http://arxiv.org/abs/1311.4895 (2013)

  29. Fowler, A. G., Mariantoni, M., Martinis, J. M. & Cleland, A. N. Surface codes: towards practical large-scale quantum computation. Phys. Rev. A 86, 032324 (2012)

    Article  ADS  CAS  Google Scholar 

  30. Aaronson, S. & Gottesman, D. Improved simulation of stabilizer circuits. Phys. Rev. A 70, 052328 (2004)

    Article  ADS  CAS  Google Scholar 

  31. Cabello, A., Severini, S. & Winter, A. (Non-)Contextuality of physical theories as an axiom. Preprint at http://arxiv.org/abs/1010.2163 (2010)

  32. Cabello, A., Severini, S. & Winter, A. Graph-theoretic approach to quantum correlations. Phys. Rev. Lett. 112, 040401 (2014)

    Article  ADS  PubMed  CAS  Google Scholar 

  33. Cormick, C., Galvão, E. F., Gottesman, D., Pablo Paz, J. & Pittenger, A. O. Classicality in discrete Wigner functions. Phys. Rev. A 73, 012301 (2006)

    Article  ADS  MathSciNet  CAS  Google Scholar 

  34. Howard, M. & Vala, J. Qudit versions of the qubit π/8 gate. Phys. Rev. A 86, 022316 (2012)

    Article  ADS  CAS  Google Scholar 

  35. Veitch, V., Ferrie, C., Gross, D. & Emerson, J. Negative quasi-probability as a resource for quantum computation. N. J. Phys. 14, 113011 (2012)

    Article  Google Scholar 

  36. Mari, A. & Eisert, J. Positive Wigner functions render classical simulation of quantum computation efficient. Phys. Rev. Lett. 109, 230503 (2012)

    Article  ADS  CAS  PubMed  Google Scholar 

  37. van Dam, W. & Howard, M. Noise thresholds for higher-dimensional systems using the discrete Wigner function. Phys. Rev. A 83, 032310 (2011)

    Article  ADS  CAS  Google Scholar 

  38. Appleby, D. M., Bengtsson, I. & Chaturvedi, S. Spectra of phase point operators in odd prime dimensions and the extended Clifford group. J. Math. Phys. 49, 012102 (2008)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  39. Wallman, J. J. & Bartlett, S. D. Non-negative subtheories and quasiprobability representations of qubits. Phys. Rev. A 85, 062121 (2012)

    Article  ADS  CAS  Google Scholar 

  40. Gross, D. Hudson’s theorem for finite-dimensional quantum systems. J. Math. Phys. 47, 122107 (2006)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  41. Wootters, W. K. The discrete Wigner function. Ann. NY Acad. Sci. 480, 275–282 (1986)

    Article  ADS  MathSciNet  Google Scholar 

  42. Gibbons, K. S., Hoffman, M. J. & Wootters, W. K. Discrete phase space based on finite fields. Phys. Rev. A 70, 062101 (2004)

    Article  ADS  MathSciNet  MATH  CAS  Google Scholar 

  43. Östergård, P. R. J. A fast algorithm for the maximum clique problem. Discrete Appl. Math. 120, 197–207 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  44. Veitch, V., Mousavian, S. A. H., Gottesman, D. & Emerson, J. The resource theory of stabilizer computation. N. J. Phys. 16, 013009 (2014)

    Article  MathSciNet  Google Scholar 

  45. Howard, M., Brennan, E. & Vala, J. Quantum contextuality with stabilizer states. Entropy 15, 2340–2362 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  46. Peres, A. Two simple proofs of the Kochen-Specker theorem. J. Phys. A 24, L175–L178 (1991)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  47. Spekkens, R. W. Contextuality for preparations, transformations, and unsharp measurements. Phys. Rev. A 71, 052108 (2005)

    Article  ADS  CAS  Google Scholar 

  48. Acín, A., Fritz, T., Leverrier, A. & Sainz, A. B. A combinatorial approach to nonlocality and contextuality. Preprint at http://arxiv.org/abs/1212.4084 (2012)

Download references

Acknowledgements

M.H. was supported by the Irish Research Council (IRC) as part of the Empower Fellowship programme, and all authors acknowledge support from CIFAR and the Government of Canada through NSERC.

Author information

Authors and Affiliations

  1. Department of Mathematical Physics, National University of Ireland, Maynooth, Ireland

    Mark Howard

  2. Institute for Quantum Computing and Department of Applied Mathematics, University of Waterloo, Waterloo, Ontario N2L 3G1, Canada,

    Mark Howard, Joel Wallman, Victor Veitch & Joseph Emerson

  3. Department of Statistics, University of Toronto, 100 St George Street, Toronto, Ontario M5S 3G3, Canada,

    Victor Veitch

Authors
  1. Mark Howard
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Joel Wallman
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Victor Veitch
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Joseph Emerson
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

All authors made significant contributions to the results, interpretation and presentation of this Article.

Corresponding author

Correspondence to Joseph Emerson.

Ethics declarations

Competing interests

The authors declare no competing financial interests.

Related audio

Supplementary information

Supplementary information

This file contains the extended mathematical proof of the part of Theorem 1 pertaining to the independence number. (PDF 273 kb)

PowerPoint slides

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Howard, M., Wallman, J., Veitch, V. et al. Contextuality supplies the ‘magic’ for quantum computation. Nature 510, 351–355 (2014). https://doi.org/10.1038/nature13460

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/nature13460

This article is cited by

Comments

By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.

Search

Advanced search

Quick links

Nature Briefing AI and Robotics

Sign up for the Nature Briefing: AI and Robotics newsletter — what matters in AI and robotics research, free to your inbox weekly.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing: AI and Robotics